Select the correct match
A
f:[1,∞)→[1,∞),f(x)=2x(x−1)⇒f−1(x)=12(1±√1+4log2x)
B
f:[−1,∞]→[−1,∞],f(x)=(x+1)2−1 and f(x)=f−1(x) then x = {0, 1} only
C
f:R→R,f(x)=3x+5 then f−1(x)=x−53
D
f:[1,∞)→[2,∞),f(x)=x+1x, then f−1(x)=x−√x2−42
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video & image solution for Select the correct match by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - C) Objective Type Questions (More than one option are correct)
17 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - D) Linked Comprehension Type Questions
17 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - A) Objective Type Questions (one option is correct)
102 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-J (aakash challengers questions)
11 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
11 Videos
Similar Questions
Select a correct match:
View SolutionSelect a correct match
View SolutionSelect a correct match
View SolutionSelect out the correct match
View SolutionSelect correct matching :-
View SolutionSelect correct matched pair/ pairs:
View SolutionSelect correct match :

View SolutionSelect correct match:

View SolutionSelect correct match

View SolutionMatch the following and select the correct option
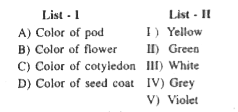
The correct match is
View Solution
