Three persons A, B and C speak at a function along with 5 other persons. If the persons speak at random, find the probability that A speaks before B and B speaks before C
38
16
35
56
The correct Answer is:B
To solve the problem, we need to find the probability that person A speaks before person B, and person B speaks before person C when all three are speaking at a function along with 5 other persons.
1. Total Number of Speakers:
There are 3 specific persons (A, B, C) and 5 other persons, making a total of 8 persons.
2. Total Arrangements:
The total number of ways to arrange 8 persons is given by the factorial of the number of persons, which is:
8!=40320
3. Favorable Outcomes:
We want to find the arrangements where A speaks before B, and B speaks before C.
- The three persons A, B, and C can be arranged in any order among themselves. The total arrangements of A, B, and C is 3! (which is 6).
- However, we are only interested in the arrangement where A is before B and B is before C. There is only 1 favorable arrangement (A, B, C).
4. Arranging Other Persons:
The remaining 5 persons can be arranged in any order. The number of arrangements for these 5 persons is:
5!=120
5. Total Favorable Outcomes:
The total number of favorable outcomes where A is before B and B is before C is:
5!×1=120
6. Probability Calculation:
The probability that A speaks before B and B speaks before C is given by the ratio of favorable outcomes to total outcomes:
P(A before B and B before C)=Favorable OutcomesTotal Outcomes=12040320
Simplifying this gives:
P=1336
Final Answer:
The probability that A speaks before B and B speaks before C is:
16
There are 3 specific persons (A, B, C) and 5 other persons, making a total of 8 persons.
The total number of ways to arrange 8 persons is given by the factorial of the number of persons, which is:
8!=40320
We want to find the arrangements where A speaks before B, and B speaks before C.
- The three persons A, B, and C can be arranged in any order among themselves. The total arrangements of A, B, and C is 3! (which is 6).
- However, we are only interested in the arrangement where A is before B and B is before C. There is only 1 favorable arrangement (A, B, C).
The remaining 5 persons can be arranged in any order. The number of arrangements for these 5 persons is:
5!=120
The total number of favorable outcomes where A is before B and B is before C is:
5!×1=120
The probability that A speaks before B and B speaks before C is given by the ratio of favorable outcomes to total outcomes:
P(A before B and B before C)=Favorable OutcomesTotal Outcomes=12040320
Simplifying this gives:
P=1336
The probability that A speaks before B and B speaks before C is:
16
Topper's Solved these Questions
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT (SECTION - C Objective Type Questions (More than one options are correct))
21 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT (SECTION - D Linked Comprehension Type Questions)
20 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT (SECTION - A Competition Level Questions)
114 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
Similar Questions
Among 10 persons, A, B, C are to speak at a function. The number of ways in which it can be done if A wants to speak before B and B wants to speak before C is a. 10!/24 b. 9!/6 c. 10!/6 d. none of these
View SolutionA and B stand in a ring with 10 other persons . If the arrangement of the twelve persons is at random , find the chance that there are exactly three persons between A and B.
View SolutionA recent survey found that the edges ages of workers in a factory as follow.
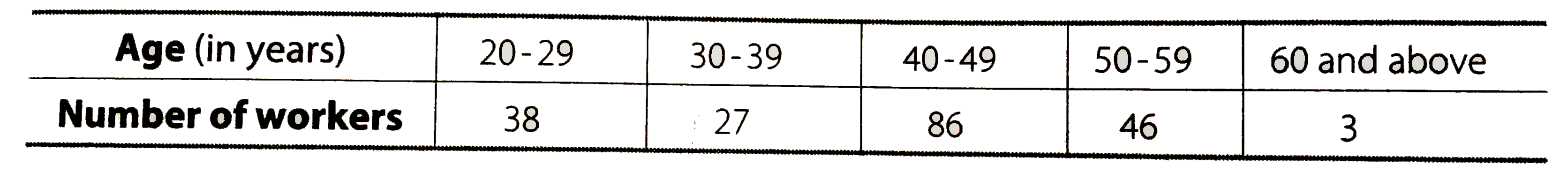
If a person is selected at random, find the probability that the person is
View SolutionThere are 7 seats in a row. Three persons take seats at random the probability that the middle seat is always occupied and no two persons are consecutive is
View SolutionThere are 7 seats in a row. Three persons take seats at random the probability that the middle seat is always occupied and no two persons are consecutive is
View SolutionIn an automobile factory, certain parts are to be fixed into the chassis in a section before it moves into another section. On a given day, one of the three persons A, B and C carries out this task. A has 45% chance, B has 35% chance and C has 20% chance of doing the task. The probability that A, B and C will take more than the allotted time is 16,110and120 respectively. If it is found that the time taken is more than the allotted time, what is the probability that A has done the task ?
View SolutionFifteen persons, among whom are A and B, sit down at random on a round table. The probability that there are 4 persons between A and B, is
View SolutionFifteen persons, among whom are A and B, sit down at random on a round table. The probability that there are 4 persons between A and B, is
View SolutionThree persons A , B and C shoot to hit a target. Their probabilities of hitting the target are 56,45and34 respectively. Find the probability that :
At least one person hit the target.
View SolutionThree persons A, B and C shoot to hit a target. Their probabilities of hitting the target are 56,45and34 respectively. Find the probability that:
Exactly two persons hit the target.
View Solution
