5 Indian and 5 Russian couples meet at a party and shake hands.
The probability that no Indian wife shakes hands with a male is
1419
919
2738
2728
The correct Answer is:A
To solve the problem of finding the probability that no Indian wife shakes hands with a male at a party where there are 5 Indian and 5 Russian couples, we can follow these steps:
1. Identify the Total Number of People:
- There are 5 Indian couples and 5 Russian couples, which means there are:
5 Indian husbands+5 Indian wives+5 Russian husbands+5 Russian wives=20 people
2. Calculate the Total Number of Handshakes:
- The total number of ways to choose 2 people from 20 to shake hands is given by the combination formula (nr):
Total handshakes=(202)=20×192=190
3. Determine the Handshakes Involving Indian Wives:
- Each Indian wife can shake hands with any of the 10 males (5 Indian husbands + 5 Russian husbands). Therefore, the number of handshakes involving Indian wives is:
Handshakes by Indian wives=5 Indian wives×10 males=50
4. Calculate the Number of Handshakes Where No Indian Wife Shakes Hands with a Male:
- If we want to find the scenarios where no Indian wife shakes hands with any male, we subtract the handshakes involving Indian wives from the total handshakes:
Handshakes where no Indian wife shakes hands with males=190−50=140
5. Calculate the Probability:
- The probability that no Indian wife shakes hands with a male is the number of favorable outcomes divided by the total outcomes:
P(no Indian wife shakes hands with a male)=140190=1419
Thus, the probability that no Indian wife shakes hands with a male is 1419.
- There are 5 Indian couples and 5 Russian couples, which means there are:
5 Indian husbands+5 Indian wives+5 Russian husbands+5 Russian wives=20 people
- The total number of ways to choose 2 people from 20 to shake hands is given by the combination formula (nr):
Total handshakes=(202)=20×192=190
- Each Indian wife can shake hands with any of the 10 males (5 Indian husbands + 5 Russian husbands). Therefore, the number of handshakes involving Indian wives is:
Handshakes by Indian wives=5 Indian wives×10 males=50
- If we want to find the scenarios where no Indian wife shakes hands with any male, we subtract the handshakes involving Indian wives from the total handshakes:
Handshakes where no Indian wife shakes hands with males=190−50=140
- The probability that no Indian wife shakes hands with a male is the number of favorable outcomes divided by the total outcomes:
P(no Indian wife shakes hands with a male)=140190=1419
Topper's Solved these Questions
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-E (assertion -reason type questions)
14 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-F (matrix-match type questions )
5 Videos
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT (SECTION - C Objective Type Questions (More than one options are correct))
21 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
Similar Questions
5 Indian and 5 Russian couples meet at a party and shake hands.
The probability that no wife shakes hands with her husband and no Indian wife shakes hands with a male is
View Solution5 Indian and 5 Russian couples meet at a party and shake hands.
The probability that no wife shakes hands with her husband is
View Solution5 Indian & 5 American couples meet at a party& shake hands. If no wife shakes hands with her own husband & no Indian wife shakes hands with a male, then the number of hand shakes that takes place in an party
View SolutionGopi buys a fish from a shop for his aquarium. The shopkeeper takes out one fish at random from a tank containing 5 male fish and 8 female fish. What is the probability that the fish taken out is a male fish?
View SolutionGopi buys a fish from a shop for his aquarium. The shopkeeper takes out one fish at random from a tank containing 5 male fish and 8 female fish. What is the probability that the fish taken out is a male fish?
View SolutionShalu buys a fish from a shop for her aquarium. The shopkeeper takes out one fish at random from a tank containing 5 male fish and 8 female fish. What is the probability that the fish taken out is a male fish?
View SolutionThere are n married couples at a party. Each person shakes hand with every person other than her or his spouse. Find the total m of hand shakes.
View SolutionThe probability that a married man watches certain TV show is 0.4 and the probability that a married woman watches the show is 0.5. The probability that a man watches the show, given that his wife does, is 0.7. Then
View SolutionFrom the employees of a company 5 persons are elected to represent them n the managing committee of the company. Particulars of the five persons are as follows: S. No. Person Age (in years) 1 2 3 4 5 Male Male Female Female Male 30 33 46 28 41 A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
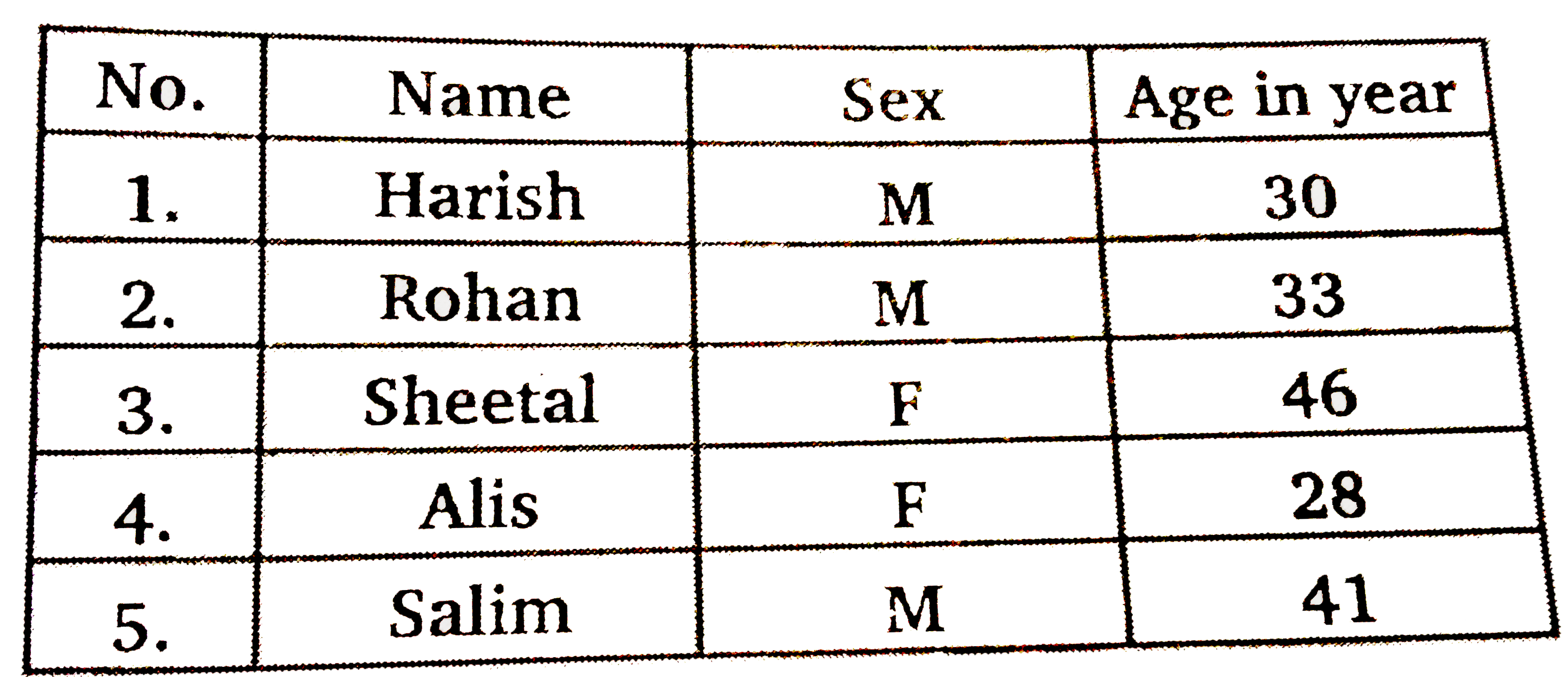
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View Solution
