A uniform tube closed at one end, contains a pallet of mercury 4 cm long. When the tube is kept vertically with closed end upwards, the length of air column between closed end and mercury pallet is 10 cm. the tube is inverted so that open end becomes upwards. find the length of trapped air column (in cm) trapped. take atmospheric pressure 76 cm of Hg.
The correct Answer is:9
To solve the problem step by step, we will analyze the situation before and after the tube is inverted.
Step 1: Understand the Initial Conditions
When the tube is vertical with the closed end upwards:
- The length of the mercury column is 4 cm.
- The length of the air column above the mercury is 10 cm.
- The total height of the mercury column and the air column is 14 cm.
Step 2: Calculate the Pressure of the Air Column (P1)
Using the atmospheric pressure (P_atm) given as 76 cm of Hg:
- The pressure exerted by the air column (P1) plus the pressure exerted by the mercury column (4 cm of Hg) must equal the atmospheric pressure.
P1+4 cm=76 cm
Rearranging gives:
P1=76 cm−4 cm=72 cm
Step 3: Invert the Tube
When the tube is inverted:
- The closed end is now downwards, and the open end is upwards.
- The mercury column remains 4 cm long, but now it will exert pressure downwards.
Step 4: Calculate the Pressure of the Air Column After Inversion (P2)
The pressure exerted by the air column (P2) now must balance the atmospheric pressure plus the pressure due to the mercury column:
P2=Patm+4 cm
Substituting the atmospheric pressure:
P2=76 cm+4 cm=80 cm
Step 5: Relate the Volumes Before and After Inversion
Using the ideal gas law, we know that:
P1⋅V1=P2⋅V2
Where:
- V1 is the volume of the air column before inversion.
- V2 is the volume of the air column after inversion.
The volume of the air column can be expressed as:
- V1=A⋅h1=A⋅10 cm (where A is the cross-sectional area)
- V2=A⋅h2=A⋅D (where D is the new height of the air column we want to find)
Step 6: Substitute into the Equation
Substituting the volumes into the equation gives:
72⋅(10A)=80⋅(DA)
Step 7: Cancel the Area
Since A is present in both sides, we can cancel it out:
72⋅10=80⋅D
Step 8: Solve for D
Now, we can solve for D:
720=80D
Dividing both sides by 80:
D=72080=9 cm
Final Answer
The length of the trapped air column after the tube is inverted is 9 cm.
---
When the tube is vertical with the closed end upwards:
- The length of the mercury column is 4 cm.
- The length of the air column above the mercury is 10 cm.
- The total height of the mercury column and the air column is 14 cm.
Using the atmospheric pressure (P_atm) given as 76 cm of Hg:
- The pressure exerted by the air column (P1) plus the pressure exerted by the mercury column (4 cm of Hg) must equal the atmospheric pressure.
P1+4 cm=76 cm
When the tube is inverted:
- The closed end is now downwards, and the open end is upwards.
- The mercury column remains 4 cm long, but now it will exert pressure downwards.
The pressure exerted by the air column (P2) now must balance the atmospheric pressure plus the pressure due to the mercury column:
Using the ideal gas law, we know that:
- V1 is the volume of the air column before inversion.
- V2 is the volume of the air column after inversion.
- V1=A⋅h1=A⋅10 cm (where A is the cross-sectional area)
- V2=A⋅h2=A⋅D (where D is the new height of the air column we want to find)
Substituting the volumes into the equation gives:
Since A is present in both sides, we can cancel it out:
Now, we can solve for D:
The length of the trapped air column after the tube is inverted is 9 cm.
Topper's Solved these Questions
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-H) (Multiple True-false Type questions)
3 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-I) (Subjective Type Questions)
5 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-F) (Matrix-match type questions)
3 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
Similar Questions
In given figure, A uniform cylindrical tube closed at one end, contains a pallet of mercury 5cm long. When the tube is kept verticaly with the closed end downward, the length of the air column trapped is 30cm. Now the tube is inverted so that the closed end goes up find the new length of the air column trapped. atmospheric pressure =75cm of mercury. (Assume temperature remains constant in this process)

View SolutionA uniform tube closed at aone end, contains a pellet of mercury 10cm long. When the tube is lept vertically with the closed-end upward, the length of the air column trapped is 20cm. Find the length of the air column trapped when the tube is inverted so that the closed-end goes down. Atmospheric pressure=75cm of mercury.
View Solution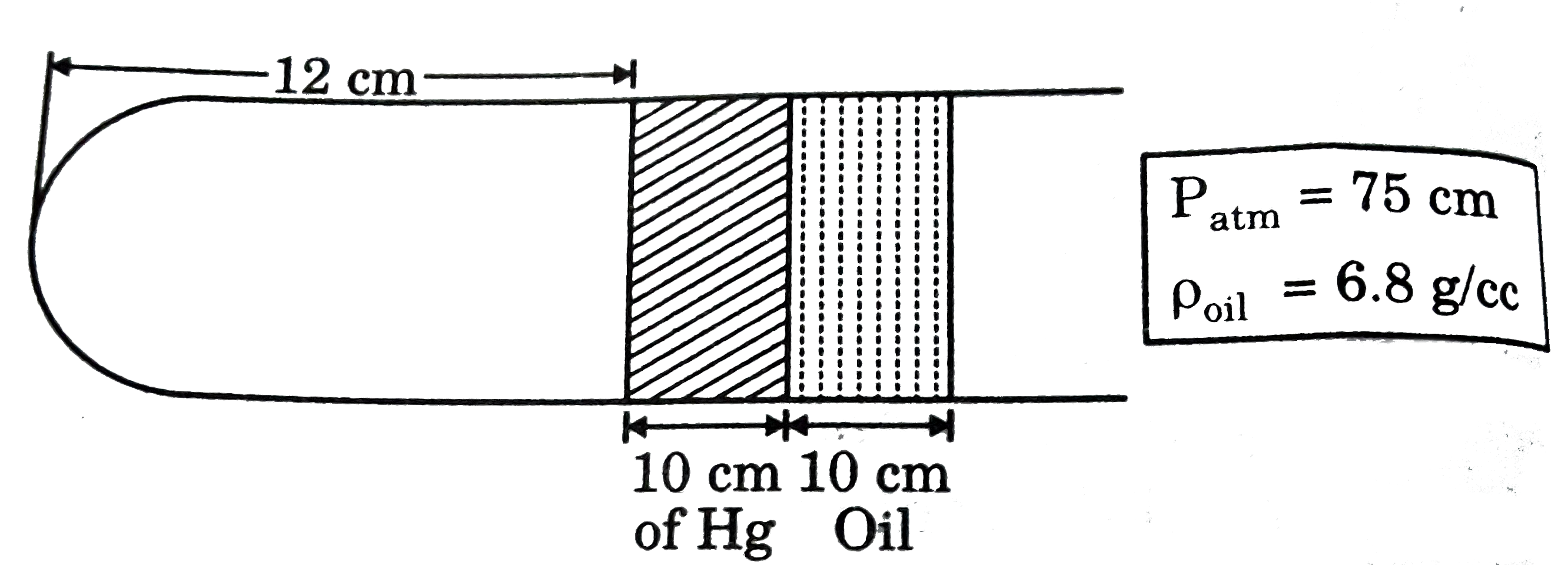
If above tube is placed vertically with the open and upward then find the length of the air column.
View SolutionThe length of an air column trapped in a capillary tube by means of a mercury pellet is 5 cm at 30∘C. If the tube is heated uniformly to 80∘C, the new length of air column is :
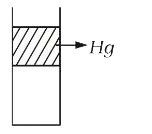
View SolutionAn air column closed in a tube sealed at one end by a Hg column having height h. When the tube is placed with open end down, the height of the air column Iis l1. If the tube is turned so that its open end is at the top, the height of the air column is l2. What is the atmospheric pressure (P0).
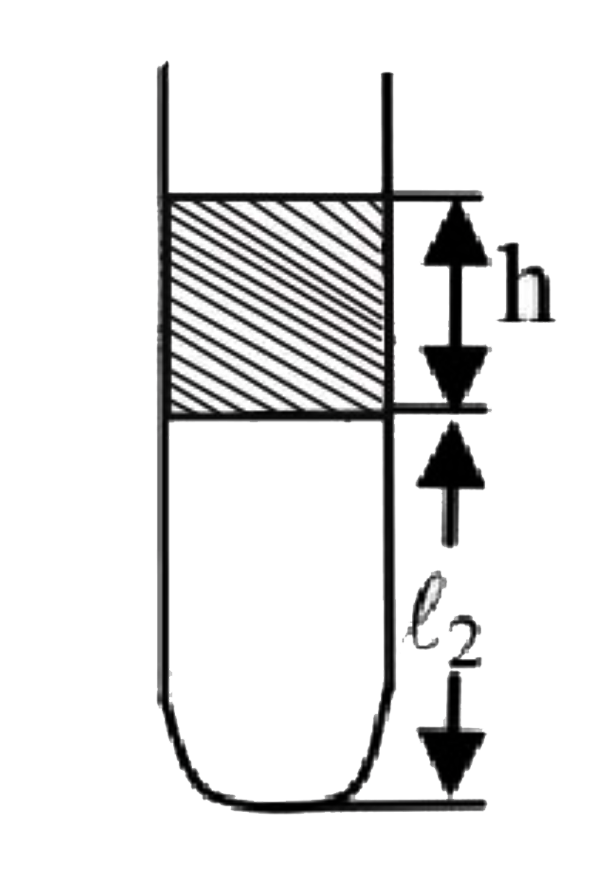
View SolutionA narrow tube 50 cm length is closed at one end contains air trapped by a certain column of Hg. When the tubes is held horizontally, the air column in if has a length of 10cm. What is the pressure exerted by air when the tube is held vertically with the open end at the bottom and the length of the air column is 15cm? Also find the vertical height of the mercury column inside the tube.
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. What will be length of the air column above mercury in the tube now? (atmospheric pressure =76 cm of Hg)
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. What will be length of the air column above mercury in the tube now? (atmospheric pressure =76 cm of Hg)
View SolutionAn ideal gas in enclosed in a tube and is held in the vertical position with the closed end upward. The length of pellet of mercury entrapping the gas is h=10 cm and the length of the tube occupied by gas is l=40 cm. Calculate the length occupied by the gas when it is turned through θ=60∘ and 90∘. Atmospheric pressure , H=76 cm of mercury.
_E01_265_Q01.png)
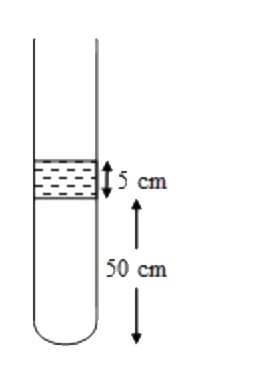
View SolutionA vertical tube of length 100 cm contains a mercury pallet of length 5 cm as shown in the figure. The length of the tube above mercury pallet if the tube is inverted is nearly: (atmospheric pressure = 75 cm Hg of Hg)
View Solution
