A uniformly charged disc of radius R with a total charge Q lies in the XY -plane. Find the electric field at a point at a point P, along the Z-a xis that passes through the centre of the discc perpendicular to its plane. Discuss the limit when R>>z.
Strategy : By treating the disc as a set of concentric uniformly charged rings, the problem could be solved by using the result obtained in example for ring. Consider a ring of radius of radius r' and thickness as shown in figure.
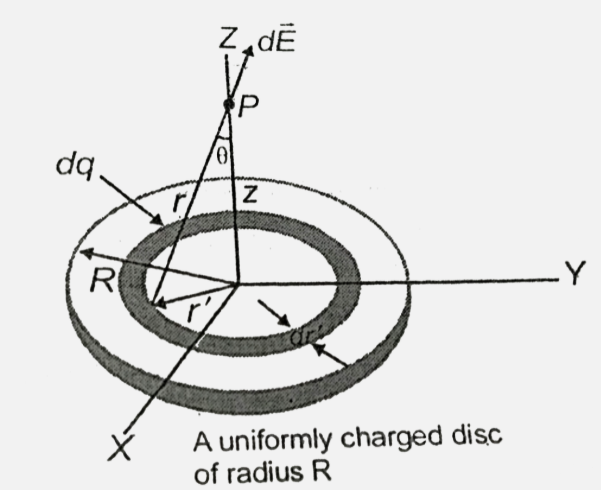
By symmetry arguments the electric field at P points in the +z direction . Since the ring has charge dq=σ(2πr'dr') , we see that the ring givesa contribution.

dEz=14πε0zdq(r'2+z2)3/2=14πε0z(2πσr'dr')(r'2+z2)3/2
integrating from '=0 to r'=R the total electric fieldat P becomes.
Ez=∫dEz=σz2ε0∫R0r'dr'(r'r+z2)3/2=σz4ε0∫R2+z2z2duu3/2=σz4ε0u−1/2(−1/2))∣∣
∣∣R+z2z2
=−σz2ε0[1√R2+z2−1z]=σ2ε0[1−z√R2+z2]
⇒Ez=σ2ε0[1−z√R2+z2]
To show that the "point-charge" limit the recovered for z>>R, we make use of the Binomial expansion
1−z√z2+R2=1−(1−R2z2)−1/2=1−(1−12R2z2+.....)=12R2z2
This gives
Ez=σ2εR2z2=14πε0σπR2z2=14πε0Qz2
which is indeed the expected "point-charge" result . On the other hand, we may also consider the limit where R>>z. Physically this means that the plane is very large , or the field point P is extremely close to the surface of the plane. The electric field in this limit becomes,in unit-vector rotation.
→E=⎧⎪⎨⎪⎩σ2ε0ˆk,z>0−σ2ε0ˆk,z>0
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - TRY YOURSELF33videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - ASSIGNMENT(SECTION-A) Objective Type Question45videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)10videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)15videos
ELECTROMAGNETIC INDUCTION
Similar Questions
If a circular concentric hole is made on a disc then about an axis passing through the centre of the disc and perpendicular to its plane
View SolutionConsider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
View SolutionA ring of radius a contains a charge q distributed. uniformly ober its length. Find the electric field at a point. on the axis of the ring at a distance x from the centre.
View SolutionRadius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
View SolutionA disc has mass 9 m. A hole of radius R/3 is cut from it as shown in the figure. The moment of inertia of remaining part about an axis passing through the centre 'O' of the disc and perpendicular to the plane of the disc is :
 View Solution
View SolutionA charge q is uniformly distributed on a non-conducting disc of radius R. It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
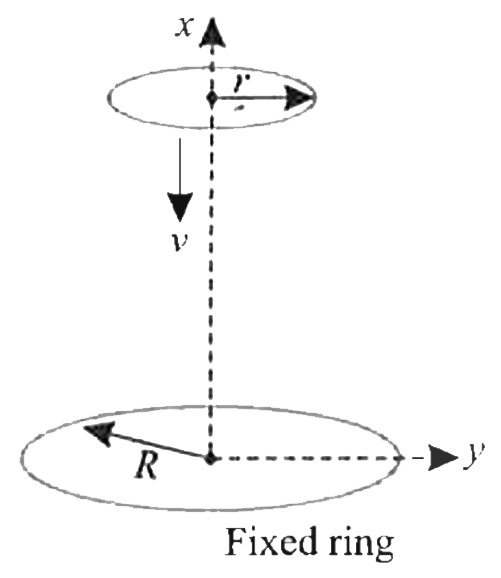
View SolutionA ring of radius R is placed in the plane its centre at origin and its axis along the x-asis and having uniformly distributed positive charge. A ring of radius r(<<R) and coaxial with the larger ring is moving along the axis with constant velcity , then the variation of electrical flux (ϕ) passing through the smaller ring with position will be best represent by :
 View Solution
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .
 View Solution
View SolutionThe radius of gyration of a uniform disc of radius R, about an axis passing through a point R2 away from the centre of disc, and perpendicular to the plane of disc is:
View SolutionThe radius of gyration of a uniform disc of radius R, about an axis passing through a point R2 away from the centre of disc, and perpendicular to the plane of disc is:
View Solution

