Two point charges of +16μCand−9μC are placed 8 cm apart in air. Determine the position of the point at which the resultant electric field is zero.
To solve the problem of finding the position where the resultant electric field is zero due to two point charges of +16 µC and -9 µC placed 8 cm apart, we can follow these steps:
1. Identify the Charges and Their Positions:
- Let charge A=+16μC be at position x=0 cm.
- Let charge B=−9μC be at position x=8 cm.
2. Assume a Point Where the Electric Field is Zero:
- Since the charges are of opposite signs, the point where the electric field is zero will be outside the two charges. Let's denote this point as C and assume it is located at a distance x from charge B (the negative charge).
3. Express Distances:
- The distance from charge A to point C will then be (8+x) cm, and the distance from charge B to point C will be x cm.
4. Write the Electric Field Equations:
- The electric field EA due to charge A at point C is given by:
EA=14πϵ016×10−6(8+x)2
- The electric field EB due to charge B at point C is given by:
EB=14πϵ09×10−6x2
5. Set the Magnitudes Equal:
- For the resultant electric field to be zero, the magnitudes of EA and EB must be equal:
EA=EB
16(8+x)2=9x2
6. Cross Multiply:
- Cross multiplying gives:
16x2=9(8+x)2
7. Expand and Rearrange the Equation:
- Expanding the right side:
16x2=9(64+16x+x2)
16x2=576+144x+9x2
- Rearranging gives:
16x2−9x2−144x−576=0
7x2−144x−576=0
8. Use the Quadratic Formula:
- Using the quadratic formula x=−b±√b2−4ac2a:
- Here, a=7, b=−144, c=−576.
- Calculate the discriminant:
b2−4ac=(−144)2−4×7×(−576)=20736+16128=36864
- Now calculate x:
x=144±√368642×7=144±19214
- This gives two potential solutions:
x1=33614=24cm(valid)
x2=−4814(not valid, as distance cannot be negative)
9. Conclusion:
- The point where the resultant electric field is zero is 24cm from charge B (the -9 µC charge).
Final Answer:
The position at which the resultant electric field is zero is 24 cm from the -9 µC charge.
- Let charge A=+16μC be at position x=0 cm.
- Let charge B=−9μC be at position x=8 cm.
- Since the charges are of opposite signs, the point where the electric field is zero will be outside the two charges. Let's denote this point as C and assume it is located at a distance x from charge B (the negative charge).
- The distance from charge A to point C will then be (8+x) cm, and the distance from charge B to point C will be x cm.
- The electric field EA due to charge A at point C is given by:
EA=14πϵ016×10−6(8+x)2
- The electric field EB due to charge B at point C is given by:
EB=14πϵ09×10−6x2
- For the resultant electric field to be zero, the magnitudes of EA and EB must be equal:
EA=EB
16(8+x)2=9x2
- Cross multiplying gives:
16x2=9(8+x)2
- Expanding the right side:
16x2=9(64+16x+x2)
16x2=576+144x+9x2
- Rearranging gives:
16x2−9x2−144x−576=0
7x2−144x−576=0
- Using the quadratic formula x=−b±√b2−4ac2a:
- Here, a=7, b=−144, c=−576.
- Calculate the discriminant:
b2−4ac=(−144)2−4×7×(−576)=20736+16128=36864
- Now calculate x:
x=144±√368642×7=144±19214
- This gives two potential solutions:
x1=33614=24cm(valid)
x2=−4814(not valid, as distance cannot be negative)
- The point where the resultant electric field is zero is 24cm from charge B (the -9 µC charge).
The position at which the resultant electric field is zero is 24 cm from the -9 µC charge.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - ASSIGNMENT(SECTION-A) Objective Type Question
45 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION-B(OBJECTIVE TYPE QUESTION( ONLY ONE ANSWER)
12 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
Similar Questions
The position of the point where the net electric field will be zero is
View SolutionTwo point charges2μC and 8μC areplaced 12 cm apart. Find the position of the point where the electric field intensity will be zero.
View SolutionTwo point charges of 5μC and 20μC are separated by a distance of 2m. Find the point on the line joining them at which electric field intensity is zero.
View SolutionAnswer the following:
(a) Derive the expression for the electric potential at any point P, at distance r from the centre of an electric dipole, making angle α, with its axis.
(b) Two point charges 4μCand+1μC are separated by a distance of 2m in air. Find the point on the line joining charges at which the net electric field of the system is zero.
View SolutionTwo electric charges of 9 μC and -3 μC are placed 0.16 m apart in air. There will be a point P at which electric potential is zero on the line joining two charges and in between them. The distance of P from 9 μC is
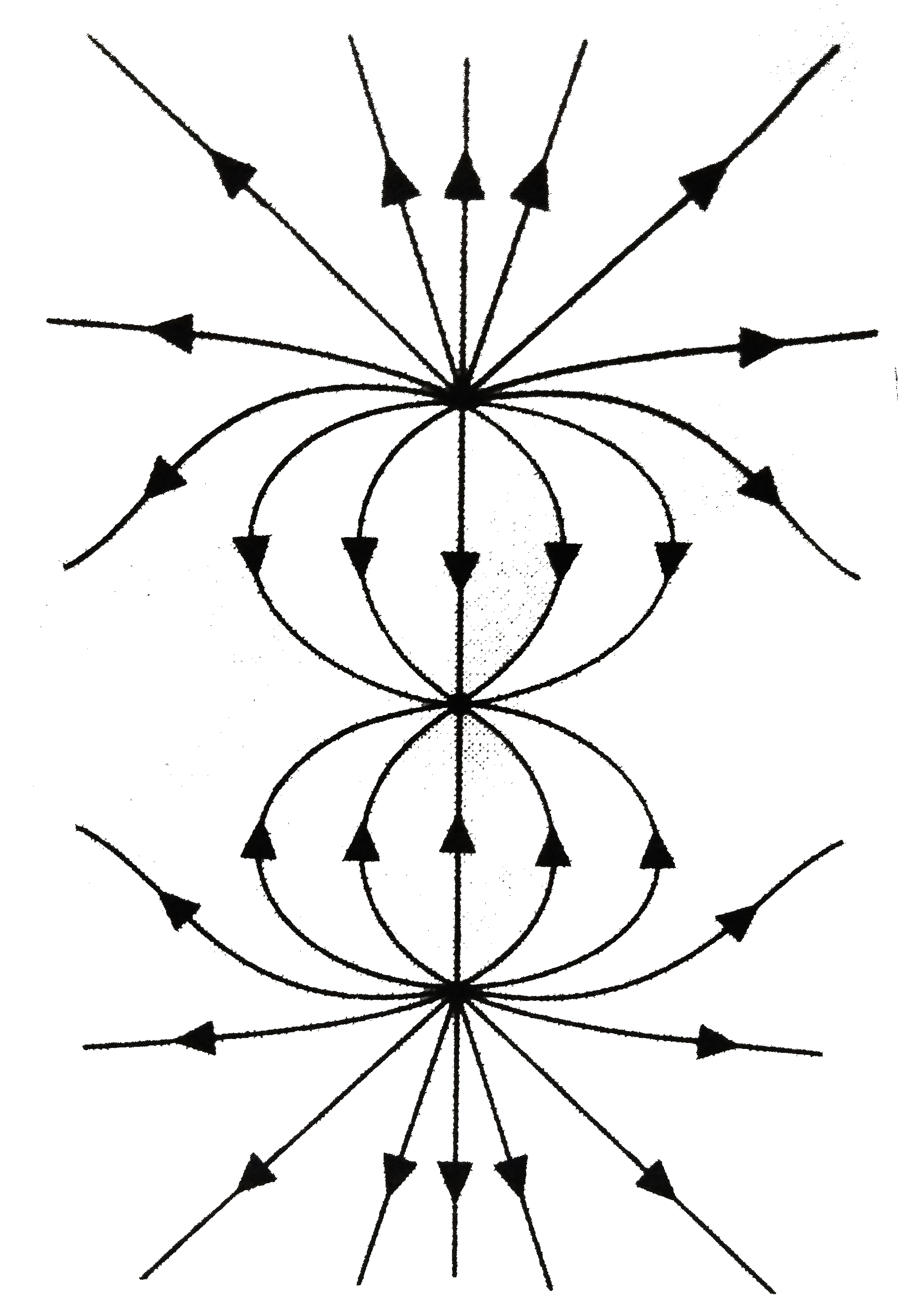
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is the magnitude of the electric field the smallest? Explain your reasoning. Explain how the fields produced by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View SolutionTwo point charge - 5μC and + 3μC are placed 64 cm apart. At what points on the line joining the two charges is the electric potential zero. Assume the potential at infinity to be zero.
View SolutionTwo electric charges 12μC and −6μC are placed 20cm apart in air. There will be a point P on the line joining these charges and outside the region between them, at which the electric potential is zero. The distance of P from 6μC charge is
View SolutionTwo positive point charges of 12 and 5 microcoulombs, are placed 10 cm apart in air. The work needed to bring them 4 cm closer is
View SolutionTwo positive point charges of 12 and 5 microcoulombs, are placed 10 cm apart in air. The work needed to bring them 4 cm closer is
View Solution
