The maximum electric field upon the axis of a circular ring (q,R) is given by E=qπε0R2×16√n . Find n.
The correct Answer is:3
To solve the problem of finding the value of n in the equation for the maximum electric field along the axis of a circular ring, we can follow these steps:
Step 1: Understand the Electric Field Expression
The electric field E along the axis of a circular ring with charge q and radius R is given by:
E=qπϵ0R2⋅16√n
Step 2: Find the Expression for Electric Field on the Axis
The electric field E at a distance x from the center of the ring along its axis is given by:
E(x)=qx(R2+x2)3/2
Step 3: Differentiate the Electric Field Expression
To find the maximum electric field, we need to differentiate E(x) with respect to x and set the derivative to zero:
dEdx=0
Step 4: Apply the Product and Chain Rule
Using the quotient rule for differentiation, we differentiate E(x):
dEdx=(R2+x2)3/2⋅q−qx⋅32(R2+x2)1/2⋅2x(R2+x2)3
Setting this equal to zero gives:
(R2+x2)3/2⋅q−3qx2(R2+x2)1/2=0
Step 5: Simplify the Equation
Factoring out q and simplifying:
(R2+x2)1/2⋅(R2+x2−3x2)=0
This leads to:
R2−2x2=0⟹R2=2x2⟹x=R√2
Step 6: Substitute x Back into the Electric Field Expression
Substituting x=R√2 into the electric field expression:
E(R√2)=q⋅R√2(R2+(R√2)2)3/2
Calculating the denominator:
R2+R22=3R22
Thus,
E(R√2)=q⋅R√2(3R22)3/2=q⋅R√23√3R34=4q3√3R2√2
Step 7: Equate to Given Expression
Now we equate this to the given expression:
4q3√3R2√2=qπϵ0R2⋅16√n
Cancelling q and R2 gives:
43√3√2=1πϵ0⋅16√n
Step 8: Solve for n
Rearranging gives:
6√n=3√3√2πϵ04
Squaring both sides:
36n=(3√3√2πϵ0)216
Thus,
n=(3√3√2πϵ0)2576
After simplification, we find that n=3.
Final Answer
Therefore, the value of n is:
3
The electric field E along the axis of a circular ring with charge q and radius R is given by:
E=qπϵ0R2⋅16√n
The electric field E at a distance x from the center of the ring along its axis is given by:
E(x)=qx(R2+x2)3/2
To find the maximum electric field, we need to differentiate E(x) with respect to x and set the derivative to zero:
dEdx=0
Using the quotient rule for differentiation, we differentiate E(x):
dEdx=(R2+x2)3/2⋅q−qx⋅32(R2+x2)1/2⋅2x(R2+x2)3
Setting this equal to zero gives:
(R2+x2)3/2⋅q−3qx2(R2+x2)1/2=0
Factoring out q and simplifying:
(R2+x2)1/2⋅(R2+x2−3x2)=0
This leads to:
R2−2x2=0⟹R2=2x2⟹x=R√2
Substituting x=R√2 into the electric field expression:
E(R√2)=q⋅R√2(R2+(R√2)2)3/2
Calculating the denominator:
R2+R22=3R22
Thus,
E(R√2)=q⋅R√2(3R22)3/2=q⋅R√23√3R34=4q3√3R2√2
Now we equate this to the given expression:
4q3√3R2√2=qπϵ0R2⋅16√n
Cancelling q and R2 gives:
43√3√2=1πϵ0⋅16√n
Rearranging gives:
6√n=3√3√2πϵ04
Squaring both sides:
36n=(3√3√2πϵ0)216
Thus,
n=(3√3√2πϵ0)2576
After simplification, we find that n=3.
Therefore, the value of n is:
3
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION-H( MULTIPLE TRUE-FALSE QUESTIONS)
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION-I(SUBJECTIVE TYPE QUESTIONS)
2 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION-F ( Matrix-Match Type Questions)
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
Similar Questions
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be
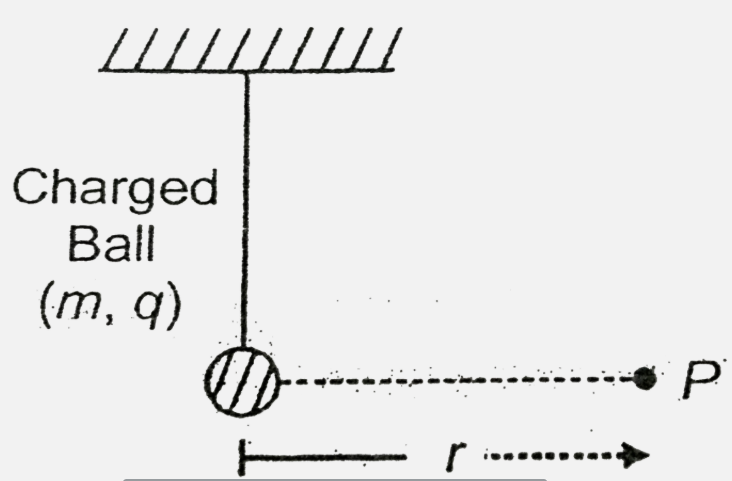
View SolutionThe electric field at point P due to a charged ball is given by
Ep=14πε0qr2
To measure 'E' at point P, A test charge q0 is placed at point P and measure electric force F upon the test charge. Check whether Fq0 is equal to 14πε0qr2 or not .
View SolutionThe maximum electric field at a point on the axis of a uniformly charged ring is E0. At how many points on the axis will the magnitude of the electric field be E0/2.
View SolutionIn the following question a statement of assertion (A) is followed by a statement of reason (R )
A : Two large conducting spheres carrying charges Q1andQ2 are brought close to each other. The magnitude of electrostatic force between them is exactly given by Q1Q2/4πε0r2 , where r is the distance between their centres.
R: Here charges Q1andQ2 can be assumed to be concentrated at the centres of their respective spheres.
View SolutionFor spherical symmetrical charge distribution, variation of electric potential with distance from centre is given in diagram Given that : V=q4πε0R0 for r≤R0andV=q4πε0r for r≥R0
Then which option (s) are correct :
(1) Total charge within 2R0 is q
(2) Total electrosstatic energy for r≤R0 is non-zero
(3) At r=R0 electric field is discontinuous
(4) There will be no charge anywhere except at r<R0

View SolutionElectric potentiall at centre of non conducting sphere of radii .r. is ____ [q is charge in itk=14πε0]
View SolutionIn the following question a statement of assertion (A) is followed by a statement of reason (R )
A: If E be electric field at a point , in free space then energy density at that point will be 12ε0E2 .
R , electrostatic field is a conservative field .
View SolutionElectric field on the axis of a small electric dipole at a distance r is →E1 and →E2 at a distance of 2r on a line of perpendicular bisector is
View SolutionA dipole of dipole moment P is kept at the centre of a ring of radius R and charge Q. If the dipole lies along the axis of the ring. Electric force on the ring due to the dipole is : (K=14πε0)
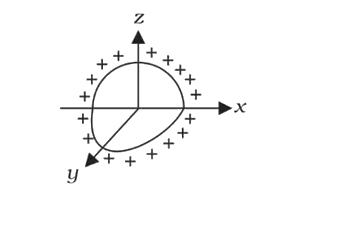
View SolutionIn the given figure two semicircular wires are connected which are in and plane respectively. And +2q0 charge is distributed uniformly over it. The magnitude of electric field intensity at the origin is q02n/2π2ε0R2 find n.
View Solution
