The centre of mass of a solid cone along the line form the center of the base to the vertex is at
One-fourth of the height
One-third of the height
One-fifth of the height
None of these
The correct Answer is:A
To find the center of mass of a solid cone along the line from the center of the base to the vertex, we can follow these steps:
1. Identify the Geometry of the Cone:
- Consider a solid cone with height H and a circular base. The vertex of the cone is at the top, and the center of the base is at the bottom.
2. Understand the Center of Mass Concept:
- The center of mass of an object is the point where the mass of the object can be considered to be concentrated. For symmetrical objects, it often lies along the axis of symmetry.
3. Determine the Position of the Center of Mass:
- For a solid cone, the center of mass is located along the vertical axis from the base to the vertex.
4. Use the Formula for the Center of Mass of a Solid Cone:
- The center of mass of a solid cone is located at a distance of H4 from the base of the cone. This is derived from the integration of the mass distribution within the cone.
5. Conclusion:
- Therefore, the center of mass of the solid cone along the line from the center of the base to the vertex is at a height of H4 from the base.
Final Answer:
The center of mass of a solid cone along the line from the center of the base to the vertex is at a distance of H4 from the base.
---
- Consider a solid cone with height H and a circular base. The vertex of the cone is at the top, and the center of the base is at the bottom.
- The center of mass of an object is the point where the mass of the object can be considered to be concentrated. For symmetrical objects, it often lies along the axis of symmetry.
- For a solid cone, the center of mass is located along the vertical axis from the base to the vertex.
- The center of mass of a solid cone is located at a distance of H4 from the base of the cone. This is derived from the integration of the mass distribution within the cone.
- Therefore, the center of mass of the solid cone along the line from the center of the base to the vertex is at a height of H4 from the base.
The center of mass of a solid cone along the line from the center of the base to the vertex is at a distance of H4 from the base.
Similar Questions
Find the centre of mass of a uniform solid cone.
View SolutionA uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, as shown. The centre of mass of the composite solid lies at the centre of base of the cone. The height of the cone is

View Solution
Knowledge Check
The centre of gravity of a hollow cone of height h is at distance x from its vertex where the value of x is:
Ah/3Bh/4C2h/3D3h/4
A solid sphere of radius r is melted and cast into the shape of a solid cone of height r , the radius of the base of the cone is (a) 2r (b) 3r (c) r (d) 4r
View SolutionDistance of the centre of mass of a solid uniform cone from its vertex is z0. It the radius of its base is R and its height is h then z0 is equal to:
View SolutionA solid sphere of radius r is melted and cast into the shape of a solid cone of height r , the radius of the base of the cone is
View SolutionThe height of a solid cone is 12cm and the area of the circular base is 64πcm2.A plane parallel to the base of the cone cuts through the cone 9cm above the vertex of the cone ,the area of the base of the new cone so formed is a)9πcm2 b)16πcm2 c)25πcm2 d)36πcm2
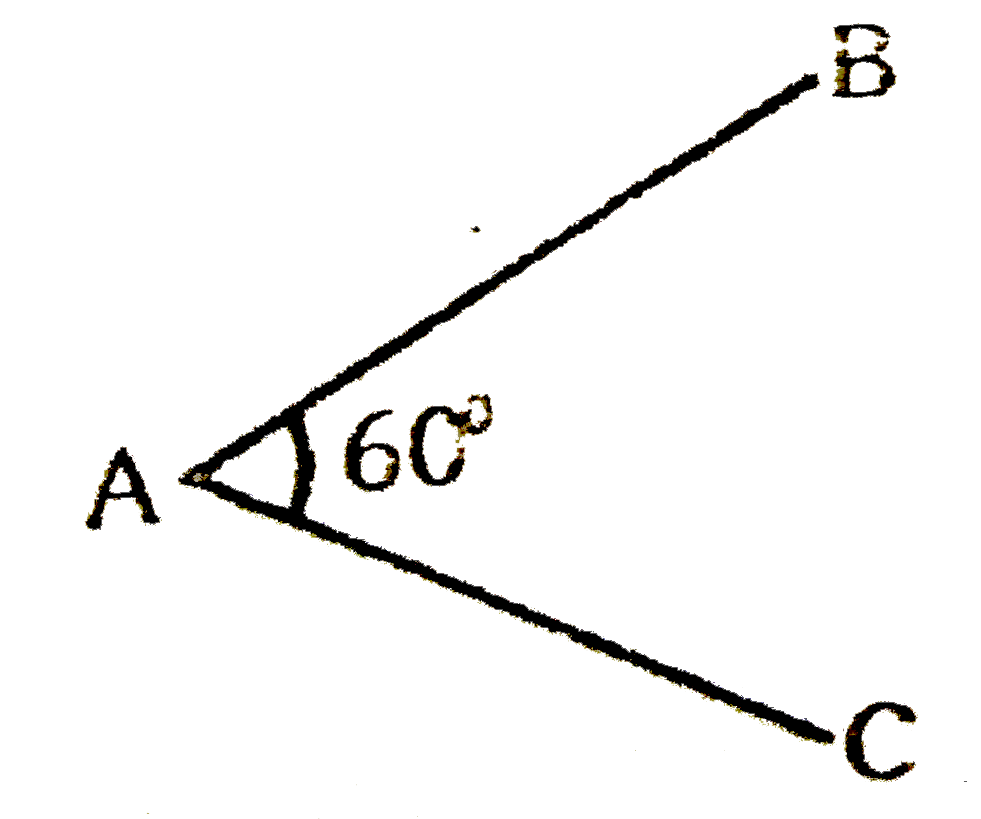
View SolutionA uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
View SolutionLet the base of a triangle lie along the line x = a and be of length a. The area of this triangles is a2, if the vertex lies on the line
View SolutionThe surface area of a solid metallic sphere is 616 cm2 . It is melted and recast into a cone of height 28 cm. Find the diameter of the base of the cone so formed (Use π=22/7 )
View Solution
