Ratio among linear expansion coefficient (c), area expansion coefficient (B) and volume expansion coefficient (Y) is
1:2:3
3:2:1
4:3:2
All of these
To find the ratio among the linear expansion coefficient (c), area expansion coefficient (B), and volume expansion coefficient (Y), we can follow these steps:
Step 1: Understanding Linear Expansion Coefficient
The linear expansion coefficient (c) is defined as the fractional change in length per degree change in temperature. For a solid body undergoing isotropic expansion, we can assume the same coefficient applies in all directions.
Step 2: Relating Linear Expansion to Area Expansion
For a solid body, the area (A) is related to the length (L) by the formula:
A=L2
When the temperature changes, the new length (L') can be expressed as:
L′=L0(1+cΔT)
where L0 is the original length and ΔT is the change in temperature.
Thus, the new area becomes:
A′=(L0(1+cΔT))2
Expanding this, we get:
A′=L20(1+2cΔT+c2(ΔT)2)
Neglecting the higher-order term c2(ΔT)2 (since c is very small), we have:
A′≈A0(1+2cΔT)
Thus, the change in area is:
ΔA≈2cA0ΔT
From this, we can define the area expansion coefficient (B):
B=ΔAA0ΔT=2c
Step 3: Relating Linear Expansion to Volume Expansion
For a cube of side length L, the volume (V) is given by:
V=L3
When the temperature changes, the new volume (V') can be expressed as:
V′=(L0(1+cΔT))3
Expanding this, we get:
V′=L30(1+3cΔT+3c2(ΔT)2+c3(ΔT)3)
Neglecting the higher-order terms, we have:
V′≈V0(1+3cΔT)
Thus, the change in volume is:
ΔV≈3cV0ΔT
From this, we can define the volume expansion coefficient (Y):
Y=ΔVV0ΔT=3c
Step 4: Finding the Ratio
Now that we have the relationships:
- B=2c
- Y=3c
We can express the ratio of the coefficients:
c:B:Y=c:2c:3c
Thus, simplifying this gives:
1:2:3
Conclusion
The ratio among the linear expansion coefficient (c), area expansion coefficient (B), and volume expansion coefficient (Y) is:
1:2:3
---
The linear expansion coefficient (c) is defined as the fractional change in length per degree change in temperature. For a solid body undergoing isotropic expansion, we can assume the same coefficient applies in all directions.
For a solid body, the area (A) is related to the length (L) by the formula:
A=L2
L′=L0(1+cΔT)
where L0 is the original length and ΔT is the change in temperature.
A′=(L0(1+cΔT))2
Expanding this, we get:
A′=L20(1+2cΔT+c2(ΔT)2)
A′≈A0(1+2cΔT)
Thus, the change in area is:
ΔA≈2cA0ΔT
B=ΔAA0ΔT=2c
For a cube of side length L, the volume (V) is given by:
V=L3
V′=(L0(1+cΔT))3
Expanding this, we get:
V′=L30(1+3cΔT+3c2(ΔT)2+c3(ΔT)3)
V′≈V0(1+3cΔT)
Thus, the change in volume is:
ΔV≈3cV0ΔT
Y=ΔVV0ΔT=3c
Now that we have the relationships:
- B=2c
- Y=3c
c:B:Y=c:2c:3c
1:2:3
The ratio among the linear expansion coefficient (c), area expansion coefficient (B), and volume expansion coefficient (Y) is:
1:2:3
Similar Questions
Unit of coefficient of linear expansion
View SolutionDefine the coefficient of linear expansion.
View SolutionMercury has a small coefficient of volume expansion.
View SolutionFor a solid with a small expansion coefficient,
View SolutionFor a solid with a small expansion coefficient,
View SolutionThe coefficient of xn in the expansion of ex is
View SolutionThe coefficient of x5 in the expansion of (x+3)6,is
View Solution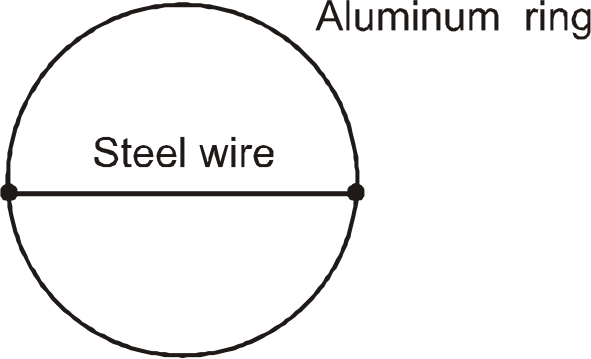
A steel wire is rigidly fixed along diameter of aluminium ring of radius R as shown. Linear expansion coefficient of steel is half of linear expansion coefficient for aluminium thent he thermal stress developed in steel wire is:
View SolutionThe coefficient of xn in the expansion of loga(1+x) is
View SolutionCoefficient of linear and coefficient of superficial expansion
View Solution
