Two SHM's with same amplitude and time period, when acting together in perpendicular directions with a phase difference of π2 give rise to
Straight motion
Elliptical motion
Circular motion
None of these
The correct Answer is:C
To solve the problem, we need to analyze the two simple harmonic motions (SHMs) acting in perpendicular directions with a phase difference of π2.
1. Define the SHM Equations:
- Let the first SHM in the x-direction be represented as:
x=Asin(ωt)
- The second SHM in the y-direction, with a phase difference of π2, can be represented as:
y=Asin(ωt+π2)
2. Simplify the y-direction SHM:
- Using the trigonometric identity, sin(θ+π2)=cos(θ), we can rewrite the y-equation:
y=Acos(ωt)
3. Square both equations:
- Now, we will square both equations:
- For the x-direction:
x2=A2sin2(ωt)
- For the y-direction:
y2=A2cos2(ωt)
4. Use the Pythagorean Identity:
- We know that sin2(θ)+cos2(θ)=1. Therefore, we can express y2 in terms of x2:
y2=A2−A2sin2(ωt)=A2−x2
5. Combine the equations:
- Rearranging gives us:
x2+y2=A2
- This is the equation of a circle with radius A.
6. Conclusion:
- The resultant motion of the two SHMs acting together is circular motion.
Final Answer:
The two SHMs give rise to circular motion.
---
- Let the first SHM in the x-direction be represented as:
x=Asin(ωt)
- The second SHM in the y-direction, with a phase difference of π2, can be represented as:
y=Asin(ωt+π2)
- Using the trigonometric identity, sin(θ+π2)=cos(θ), we can rewrite the y-equation:
y=Acos(ωt)
- Now, we will square both equations:
- For the x-direction:
x2=A2sin2(ωt)
- For the y-direction:
y2=A2cos2(ωt)
- We know that sin2(θ)+cos2(θ)=1. Therefore, we can express y2 in terms of x2:
y2=A2−A2sin2(ωt)=A2−x2
- Rearranging gives us:
x2+y2=A2
- This is the equation of a circle with radius A.
- The resultant motion of the two SHMs acting together is circular motion.
The two SHMs give rise to circular motion.
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
13 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section - B) (OBJECTIVE TYPE QUESTIONS)
30 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
A: The phase difference between the two particles shown below is π. (Assuming both particles have same time periods and same amplitudes).
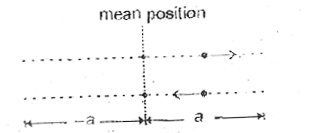
R: If the particles cross each other while they move in the opposite direction, they have a phase difference of π radian.
View SolutionTwo waves of same frequency but of amplitude a and 2a respectively superimpose over each other. The intensity at a point where the phase difference is 3π2, will be proportional to :
View Solution
Knowledge Check
Two particles execute SHM of same amplitude and same time period, about same mean position but with a phase difference between them. At an instant they are found to cross each other at x=+A3. The phase difference between them is
A2cos−1(15)B2sin−1(15)C2cos−1(13)D2sin−1(15)Two particles execute SHMs of the same amplitude and frequency along the same straight line. They cross one another when going in opposite direction. What is the phase difference between them when their displacements are half of their amplitudes ?
A60∘B30∘C120∘D150∘
When two waves with same frequency and constant phase difference interfere,
View SolutionTwo particles are performing SHM with same amplitude and time period. At an instant two particles are having velocity 1m/s but one is on the right and the other is on left of their mean positiion. When the particles have same position there speed is √3m/s. Find the maximum speed (in m/s) of particles during SHM.
View SolutionShow that for a particle executing SHM, velocity and dispacement have a phase difference of π/2.
View SolutionTwo bodies performing S.H.M. have same amplitude and frequency. Their phases at a certain instant are as shown in the figure. The phase difference between them is
_E01_002_Q01.png)
View Solutiontwo partical excuting SHM with same frequency and amplitudes Aand2A on same straight line with same mean position cross each other in opposite direction at a distance A/3 from mean possion. Find the phase difference in the two SHM s.
View SolutionWhen two waves of the same amplitude and frequency but having a phase difference of ϕ, travelling with the same speed in the same direction (positive x), interfere, then
View Solution
