A small conducting sphere is hanged by an insulating thread between the plates of a parallel plate capacitor as shown in figure. The net force on the sphere is
Towards plate A
Towards plate B
Upwards
Zero
The correct Answer is:D
To determine the net force on a small conducting sphere suspended between the plates of a parallel plate capacitor, we can follow these steps:
Step 1: Understand the setup
We have a small conducting sphere hanging by an insulating thread between the plates of a parallel plate capacitor. The capacitor consists of two plates, one positively charged and the other negatively charged.
Hint: Visualize the arrangement of the capacitor and the sphere to understand the forces acting on it.
Step 2: Identify the charges on the plates
Let’s denote the charge on the positive plate as +Q and the charge on the negative plate as −Q. The charges on the plates are equal in magnitude but opposite in direction.
Hint: Remember that in a parallel plate capacitor, the charges on the plates are equal and opposite.
Step 3: Determine the induced charge on the sphere
When the conducting sphere is placed in the electric field between the plates, it will experience induction. The charge on the sphere will be influenced by the electric field created by the plates.
- The charge induced on the side of the sphere facing the positive plate will be −Q′ (negative).
- The charge induced on the side of the sphere facing the negative plate will be +Q′ (positive).
Hint: Consider how the electric field affects the charges on the conducting sphere.
Step 4: Calculate the net induced charge on the sphere
Since the charges induced on the sphere are equal in magnitude but opposite in sign, the net induced charge Qnet on the sphere will be:
Qnet=−Q′+Q′=0
Hint: Remember that the net charge is the sum of all induced charges.
Step 5: Determine the force on the sphere
The force F acting on the sphere due to the electric field E between the plates is given by:
F=Qnet⋅E
Since we found that Qnet=0:
F=0⋅E=0
Hint: The force on a charge in an electric field is zero if the charge itself is zero.
Step 6: Conclusion
The net force acting on the small conducting sphere is zero.
Final Answer: The net force on the sphere is 0.
We have a small conducting sphere hanging by an insulating thread between the plates of a parallel plate capacitor. The capacitor consists of two plates, one positively charged and the other negatively charged.
Let’s denote the charge on the positive plate as +Q and the charge on the negative plate as −Q. The charges on the plates are equal in magnitude but opposite in direction.
When the conducting sphere is placed in the electric field between the plates, it will experience induction. The charge on the sphere will be influenced by the electric field created by the plates.
- The charge induced on the side of the sphere facing the negative plate will be +Q′ (positive).
Since the charges induced on the sphere are equal in magnitude but opposite in sign, the net induced charge Qnet on the sphere will be:
The force F acting on the sphere due to the electric field E between the plates is given by:
The net force acting on the small conducting sphere is zero.
Similar Questions
Knowledge Check
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
A slab of copper of thickness y is inserted between the plates of parallel plate capacitor as shown in figure. The separation between the plates is d. If y=d4, then the ratio of capacitance of the capacitor after nd before inserting the slab is

View SolutionA slab of copper of thickness b is inserted in between the plates of parallel plate capacitor as shown in figure. The separation of the plate is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting that slab will be

View SolutionThe separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
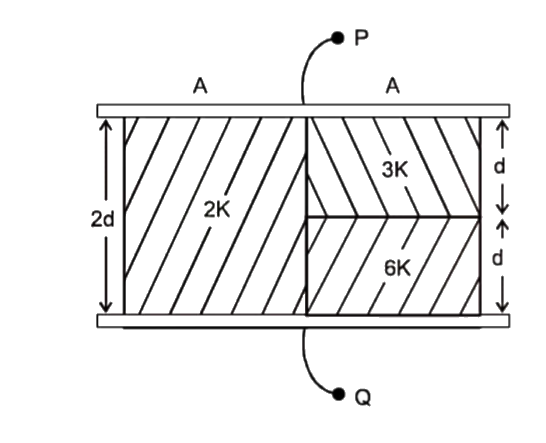
View SolutionThree different types of dielectric slabs have been arranged between the plates of a parallel plate capacitor, as shown in the figure. The equivalent capacitance of the system between the points P and Q will be
View SolutionThe expression for the capacity of the capacitor formed by compound dielectric placed between the plates of a parallel plate capacitor as shown in figure, will be (area of plate =A)

View SolutionThe plates of a parallel plate capacitor are not exactly parallel. The surface charge density is therefore,
View SolutionFor a charged parallel plate capacitor shown in the figure, the force experienced by an alpha particle will be :

View Solution
