In the above problem (example 3) what is the emf induced when current is reduced to zero and then increases again to 1.5 A in reverse direction in 25ms?
To solve the problem of finding the induced EMF when the current is reduced to zero and then increases again to 1.5 A in the reverse direction over a time interval of 25 ms, we will follow these steps:
Step 1: Understand the Problem
We need to find the induced EMF when the current changes from 1.5 A to 0 A and then back to -1.5 A (reverse direction) over a time period of 25 ms.
Step 2: Identify the Change in Magnetic Flux
The induced EMF (ε) can be calculated using Faraday's law of electromagnetic induction, which states that:
ϵ=−dΦdt
where Φ is the magnetic flux.
Step 3: Calculate Initial and Final Magnetic Flux
1. Initial Flux (Φ1) when the current is 1.5 A:
Φ1=μ0⋅n⋅π4⋅(r22−r21)⋅I
where:
- μ0=4π×10−7T m/A (permeability of free space)
- n=220 (number of turns)
- r1=2.1cm=0.021m
- r2=3.2cm=0.032m
- I=1.5A
Plugging in the values:
Φ1=4π×10−7⋅220⋅π4⋅(0.0322−0.0212)⋅1.5
2. Final Flux (Φ2) when the current is -1.5 A:
Φ2=μ0⋅n⋅π4⋅(r22−r21)⋅(−1.5)
Step 4: Calculate the Change in Flux
The change in flux (ΔΦ) is:
ΔΦ=Φ2−Φ1
Step 5: Calculate the Induced EMF
Using the change in flux and the time interval:
ϵ=−ΔΦΔt
where Δt=25ms=25×10−3s.
Step 6: Substitute Values and Solve
1. Calculate Φ1 and Φ2.
2. Find ΔΦ.
3. Substitute ΔΦ and Δt into the EMF formula to find the induced EMF.
Final Calculation
After performing the calculations, we find:
ϵ≈7.4688×10−4V
Conclusion
The induced EMF when the current is reduced to zero and then increases again to 1.5 A in the reverse direction in 25 ms is approximately 7.4688×10−4V.
---
We need to find the induced EMF when the current changes from 1.5 A to 0 A and then back to -1.5 A (reverse direction) over a time period of 25 ms.
The induced EMF (ε) can be calculated using Faraday's law of electromagnetic induction, which states that:
ϵ=−dΦdt
where Φ is the magnetic flux.
1. Initial Flux (Φ1) when the current is 1.5 A:
Φ1=μ0⋅n⋅π4⋅(r22−r21)⋅I
where:
- μ0=4π×10−7T m/A (permeability of free space)
- n=220 (number of turns)
- r1=2.1cm=0.021m
- r2=3.2cm=0.032m
- I=1.5A
Φ1=4π×10−7⋅220⋅π4⋅(0.0322−0.0212)⋅1.5
Φ2=μ0⋅n⋅π4⋅(r22−r21)⋅(−1.5)
The change in flux (ΔΦ) is:
ΔΦ=Φ2−Φ1
Using the change in flux and the time interval:
ϵ=−ΔΦΔt
where Δt=25ms=25×10−3s.
1. Calculate Φ1 and Φ2.
2. Find ΔΦ.
3. Substitute ΔΦ and Δt into the EMF formula to find the induced EMF.
After performing the calculations, we find:
ϵ≈7.4688×10−4V
The induced EMF when the current is reduced to zero and then increases again to 1.5 A in the reverse direction in 25 ms is approximately 7.4688×10−4V.
Similar Questions
An average induced emf of 0.20 V appears in a coil when the current in it is changed from 5.0 A in one direction to 5.0 A in the opposite direction in 0.20 s. find the self inductance of the coil.
View SolutionAn average induced emf of 0.20 V appears in a coil when the current in it is changed from 5.0A in one direction to 5.0 A in the opposite direction in 0.2 sec.
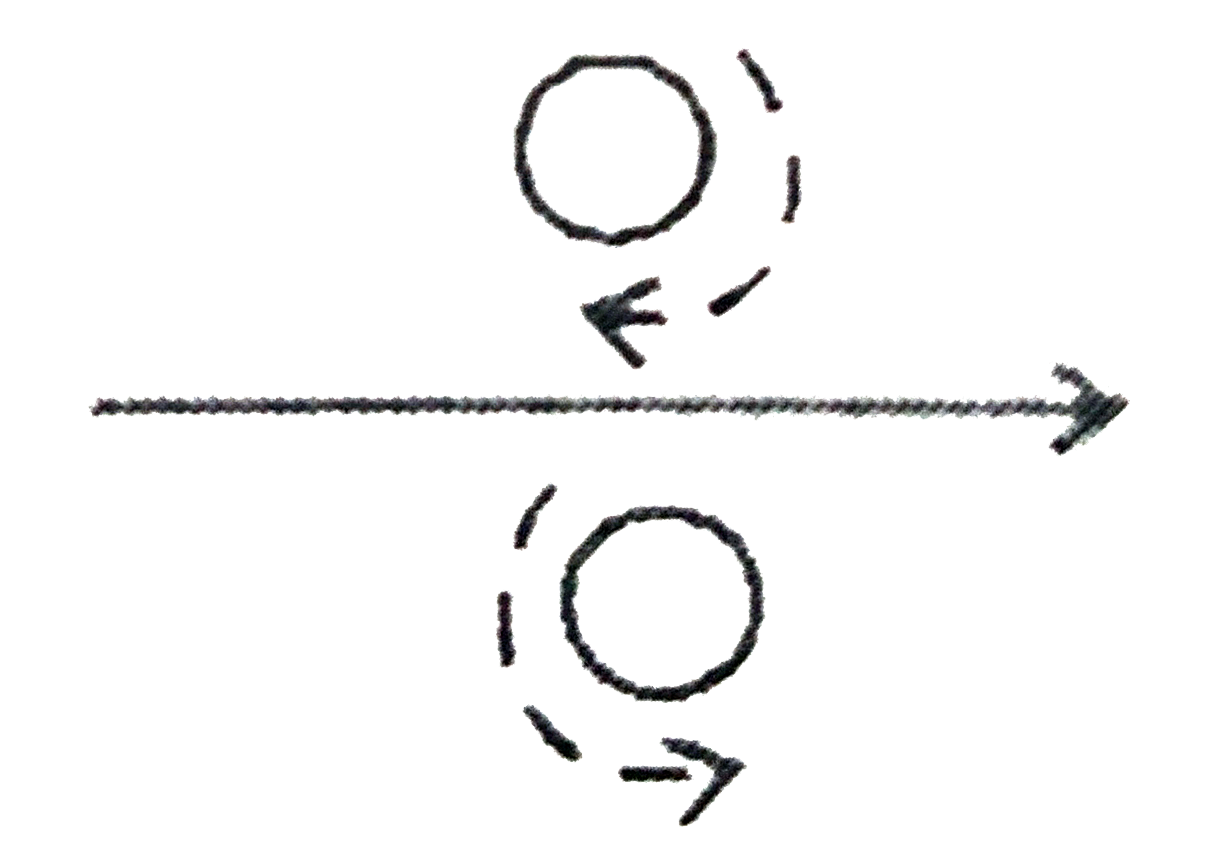
View SolutionPredict the direction of induced current in metal rings 1 and 2 when current I in the wire is steadily decreasing .
View SolutionAn average induced e.m.f. of 1V appears in a coil when the current in it is changed from 10 A in one direction to 10A in opposite direction in 0.5 sec. self-inductance of the coil is
View SolutionWhen a certain curcuit consisting of a constant e.m.f. E an inductance L and a resistance R is closed, the current in it increases with time according to curve 1. After one parameter (E, L or R) is changed, the increase in current is closed second time. Which parameter was changed and in what direction?

View SolutionTwo long conductors, separated by a distance d carry current I1andI2 in the same direction . They exert a force F on each other. Now the current in one of them is increased to two times and its direction is reversed . The distance is also increased to 3d. The new value of the force between them is
View SolutionTwo long conductors, separated by a distance d carry current I1andI2 in the same direction . They exert a force F on each other. Now the current in one of them is increased to two times and its direction is reversed . The distance is also increased to 3d. The new value of the force between them is
View SolutionA long solenoid of cross-sectional area 5.0cm2 is wound with 25 turns of wire per centimetre. It is placed in the middle of a closely wrapped coil of 10 turns and radius 25 cm as shown.
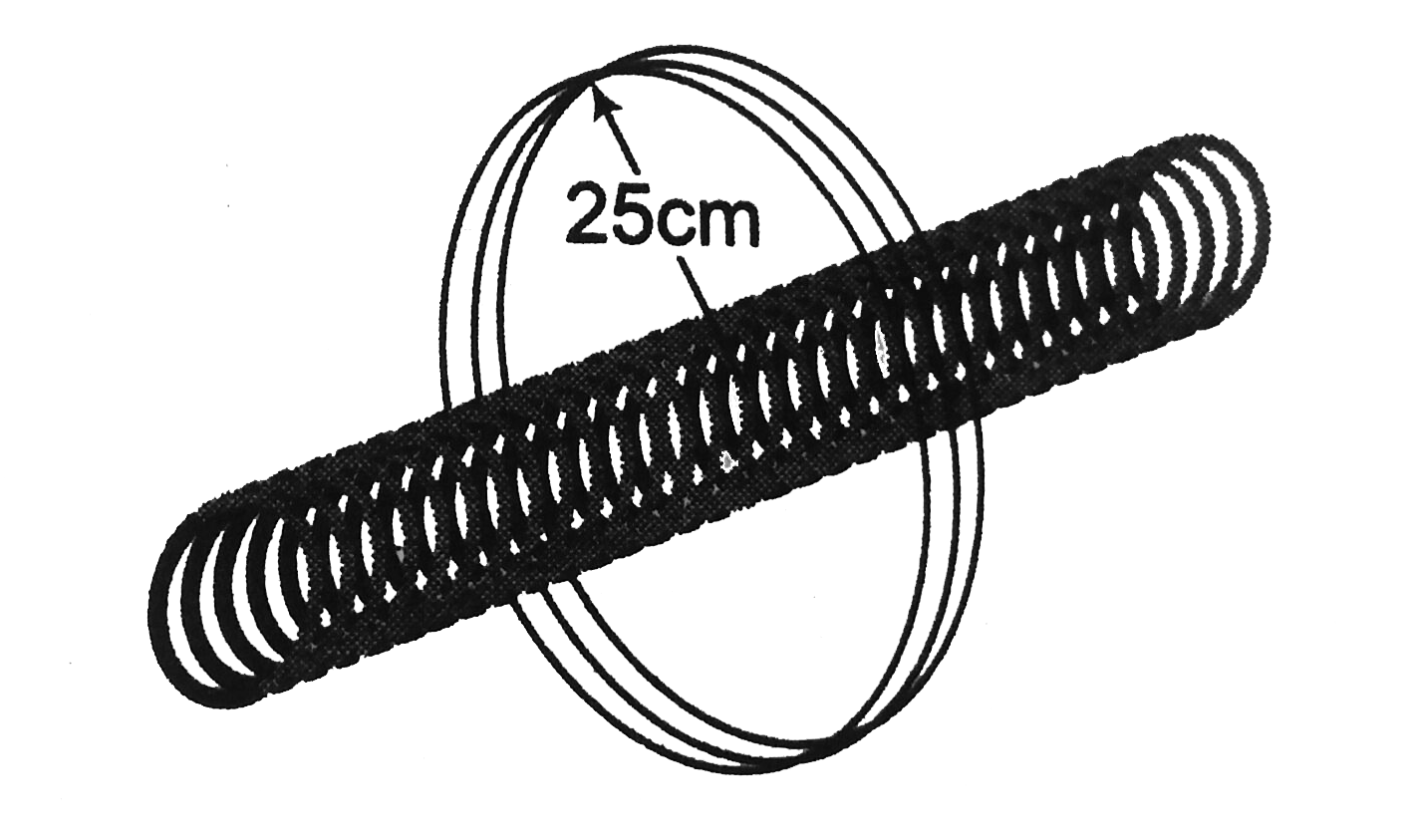
(a) What is the emf induced in the coil when the current through the solenoid is decreasing at a rate −0.20A/s ?
(b) What is the electric field induced in the coil?
View SolutionAn average emf of 20 V is induced in an inductor when the current in it is changed from 2.5A in one direction to the same valute in the opposite direction in 0.1 s. Find the self inductance of the inductor.
View SolutionA centipede was crawling in a straight line. First it crawled for 837 inch, then it crawled in the reverse direction for 558 inch. Once again it reversed direction and crawled for 1112 inch in the original direction. How far away was it finally from the starting point?
View Solution
