Ionization potential of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1 eV. According to Bohr's theory, the spectral lines emitted by hydrogen will be
Two
Three
Four
One
The correct Answer is:B
To solve the problem step by step, we will follow the concepts of Bohr's atomic model and the energy levels of the hydrogen atom.
Step 1: Understand the Ionization Potential
The ionization potential (IP) of hydrogen is given as 13.6 eV. This value corresponds to the energy required to remove an electron from the ground state (n=1) of the hydrogen atom.
Step 2: Determine the Initial Energy
The energy of an electron in the nth level of a hydrogen atom is given by the formula:
En=−13.6eVn2
For the ground state (n=1):
E1=−13.6eV12=−13.6eV
Step 3: Calculate the Final Energy After Excitation
When the hydrogen atom is excited by a photon of energy 12.1 eV, the total energy after excitation becomes:
Efinal=E1+Photon Energy=−13.6eV+12.1eV=−1.5eV
Step 4: Determine the Final State (n)
We need to find the principal quantum number (n) corresponding to the final energy of -1.5 eV. Using the energy formula:
En=−13.6eVn2
Setting En=−1.5eV:
−1.5=−13.6n2
This simplifies to:
1.5n2=13.6
n2=13.61.5≈9.0667
Taking the square root:
n≈3
Step 5: Calculate the Number of Spectral Lines
The number of spectral lines emitted when an electron transitions from a higher energy level (nf) to a lower energy level (ni) can be calculated using the formula:
Number of lines=nf−ni(nf−ni+1)2
Here, ni=1 (initial state) and nf=3 (final state):
Number of lines=3−1(3−1+1)2=2⋅32=3
Conclusion
The number of spectral lines emitted by the hydrogen atom when excited by the photon of energy 12.1 eV is 3.
The ionization potential (IP) of hydrogen is given as 13.6 eV. This value corresponds to the energy required to remove an electron from the ground state (n=1) of the hydrogen atom.
The energy of an electron in the nth level of a hydrogen atom is given by the formula:
En=−13.6eVn2
For the ground state (n=1):
E1=−13.6eV12=−13.6eV
When the hydrogen atom is excited by a photon of energy 12.1 eV, the total energy after excitation becomes:
Efinal=E1+Photon Energy=−13.6eV+12.1eV=−1.5eV
We need to find the principal quantum number (n) corresponding to the final energy of -1.5 eV. Using the energy formula:
En=−13.6eVn2
Setting En=−1.5eV:
−1.5=−13.6n2
This simplifies to:
1.5n2=13.6
n2=13.61.5≈9.0667
Taking the square root:
n≈3
The number of spectral lines emitted when an electron transitions from a higher energy level (nf) to a lower energy level (ni) can be calculated using the formula:
Number of lines=nf−ni(nf−ni+1)2
Here, ni=1 (initial state) and nf=3 (final state):
Number of lines=3−1(3−1+1)2=2⋅32=3
The number of spectral lines emitted by the hydrogen atom when excited by the photon of energy 12.1 eV is 3.
Similar Questions
Ionization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
View SolutionIonization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
View Solution
Knowledge Check
Ionization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
AoneBtwoCthreeDfour
Ionization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
View SolutionIonisation potential of hydrogen atom is 13.6eV. Hydrogen atom in ground state is excited by monochromatic light of energy 12.1eV. The spectral lines emitted by hydrogen according to Bohr's theory will be
View SolutionHydrogen atom in ground state is excited by a monochromatic radiation of λ=975Å. Number of spectral lines in the resulting spectrum emitted will be
View SolutionAn H- atom in the ground state is excited by monochromatic radiation of photon energy 13.056 eV. The number of emission lines will be (given its ionisation energy is 13.6 eV)
View SolutionExcitations energy of hydrogen atom is 13.6eV match the following
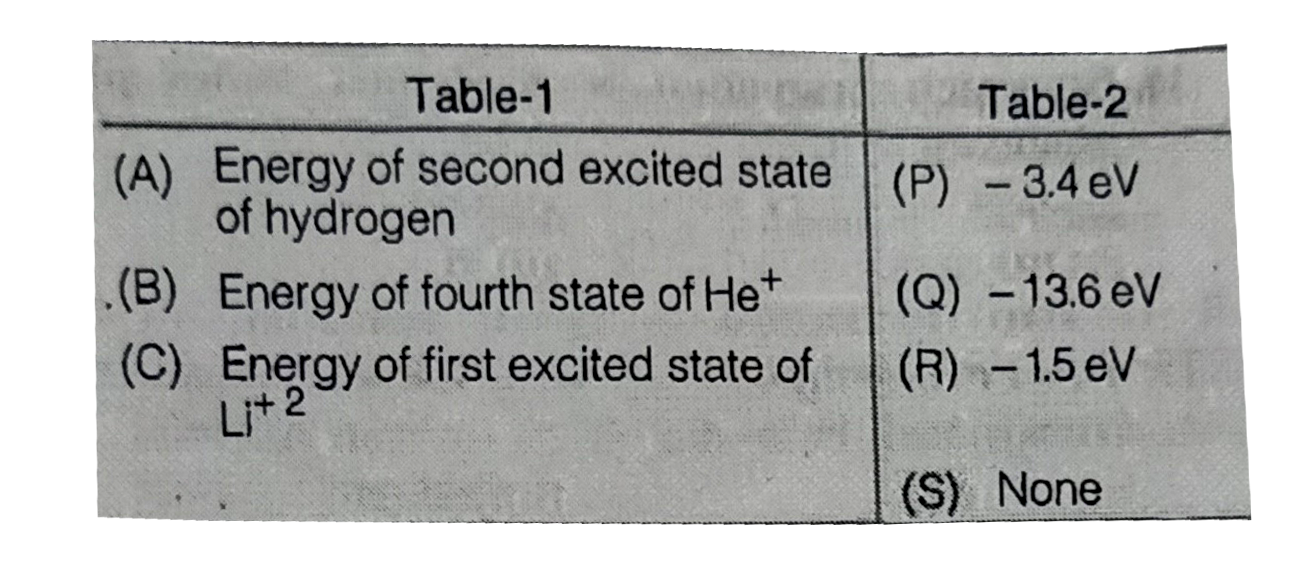
View SolutionA sample consisting of Hydrogen atoms in the ground state is excited by monochromatic radiation of energy 12.75 eV. If we were to observe the emission spectrum of this sample, then the number of spectral lines observed, will be
View SolutionThe hydrogen atom in ground state is excited by a monochromatic radiation of wavelength λ=1025A. The possible number of emmision spectral lines in the spectrum will be (hc = 12400 eV A)
View Solution
