What is the phase difference between the displacement wave and pressure wave in sound wave :-
Zero
The correct Answer is:B
To determine the phase difference between the displacement wave and the pressure wave in a sound wave, we can follow these steps:
1. Write the Equation for the Displacement Wave:
The displacement wave can be represented mathematically as:
y(x,t)=Smsin(ωt−kx)
where Sm is the amplitude, ω is the angular frequency, and k is the wave number.
2. Write the Equation for the Pressure Wave:
The pressure wave can be expressed as:
ΔP(x,t)=ΔPmcos(ωt−kx)
where ΔPm is the maximum change in pressure.
3. Convert the Pressure Wave Equation to Sine Form:
We know that the cosine function can be expressed in terms of sine:
cos(θ)=sin(θ+π2)
Therefore, we can rewrite the pressure wave equation as:
ΔP(x,t)=ΔPmsin(ωt−kx+π2)
4. Identify the Phase of Each Wave:
- For the displacement wave, the phase ϕ1 is:
ϕ1=ωt−kx
- For the pressure wave, the phase ϕ2 is:
ϕ2=ωt−kx+π2
5. Calculate the Phase Difference:
The phase difference Δϕ between the pressure wave and the displacement wave is given by:
Δϕ=ϕ2−ϕ1=(ωt−kx+π2)−(ωt−kx)
Simplifying this, we find:
Δϕ=π2
6. Conclusion:
Thus, the phase difference between the displacement wave and the pressure wave in a sound wave is:
Δϕ=π2 radians
The displacement wave can be represented mathematically as:
y(x,t)=Smsin(ωt−kx)
where Sm is the amplitude, ω is the angular frequency, and k is the wave number.
The pressure wave can be expressed as:
ΔP(x,t)=ΔPmcos(ωt−kx)
where ΔPm is the maximum change in pressure.
We know that the cosine function can be expressed in terms of sine:
cos(θ)=sin(θ+π2)
Therefore, we can rewrite the pressure wave equation as:
ΔP(x,t)=ΔPmsin(ωt−kx+π2)
- For the displacement wave, the phase ϕ1 is:
ϕ1=ωt−kx
- For the pressure wave, the phase ϕ2 is:
ϕ2=ωt−kx+π2
The phase difference Δϕ between the pressure wave and the displacement wave is given by:
Δϕ=ϕ2−ϕ1=(ωt−kx+π2)−(ωt−kx)
Simplifying this, we find:
Δϕ=π2
Thus, the phase difference between the displacement wave and the pressure wave in a sound wave is:
Δϕ=π2 radians
Topper's Solved these Questions
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-C ( Previous year Questions ))
Explore 53 Videos
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
Explore 12 Videos
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-A ( Objective type Questions ))
Explore 50 Videos
- WAVE OPTICSBook:AAKASH INSTITUTE ENGLISHChapter:WAVE OPTICSExercise:Assignment (Section-J (Aakash Challengers question))
Explore 1 Video
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTE ENGLISHChapter:WORK, ENERGY AND POWERExercise:Assignment (SECTION - D)
Explore 13 Videos
Similar Questions
What is the phase difference between the incident wave and the reflected wave in the following ?
1. Wave reflected from rigid boundary.
2. Wave reflected from free boundary.
View SolutionAt the open end of organ pipe
View SolutionWhen a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
View SolutionWhen a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
View SolutionWhat is the difference between a sound wave and a T. V. wave ?
View SolutionAnswer the question briefly and the point:
What is the phase difference between a node and its nearest antinode in a stationary wave?
View SolutionA wave of frequency 500Hz has a wave velocity of 350m/s.
(a) Find the distance between two points which are 60∘ out of phase.
(b) Find the phase difference between two displacement at a certain point at time 10−3s apart.
View SolutionA wave of frequency 500Hz has a wave velocity of 350m/s.
(a) Find the distance between two points which are 60∘ out of phase.
(b) Find the phase difference between two displacement at a certain point at time 10−3s apart.
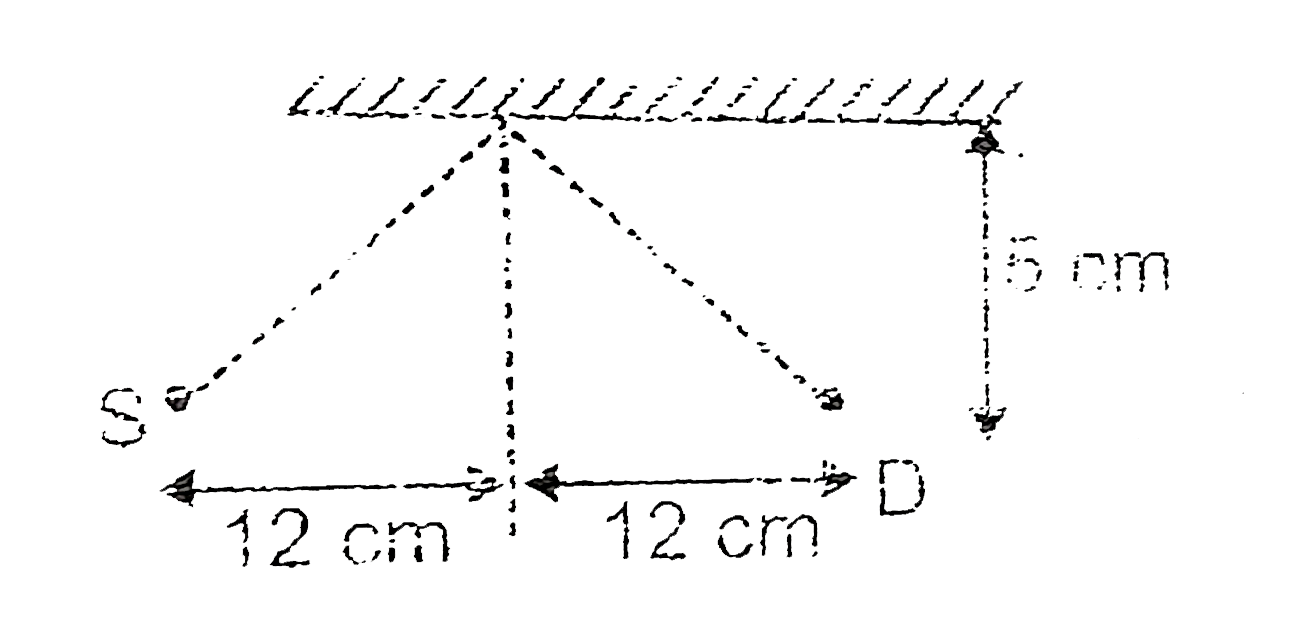
View SolutionA sound source, detector and a cardboard are arranged as shown in figure. The wave is reflected from the cardboard at the line symmetry of source edetector. Initially the path difference between the reflected wave and the direct wave is one third of the wavelength of sound. Find the minimum distance by which the cardboard should be moved upwards so that both waves are in phase.
View SolutionState two differences between light and sound waves.
View Solution