The equation of a projectile is y = ax - bx2. Its horizontal range is
ab
ba
a + b
b - a
The correct Answer is:A
To find the horizontal range of the projectile given the equation y=ax−bx2, we can follow these steps:
Step 1: Identify the coefficients
The given equation of the projectile is in the form y=ax−bx2. Here, a represents the slope of the trajectory at the origin, and b is related to the acceleration due to gravity.
Step 2: Relate coefficients to projectile motion
In projectile motion, the equation of the trajectory can be compared to the standard form:
y=xtanθ−gx22u2cos2θ
From this, we can identify:
- a=tanθ
- b=g2u2cos2θ
Step 3: Find the ratio ab
We can find the ratio ab:
ab=tanθg2u2cos2θ=2u2cos2θtanθg
Since tanθ=sinθcosθ, we can rewrite this as:
ab=2u2cos2θ⋅sinθcosθg=2u2sinθcosθg
Step 4: Use the double angle identity
Using the double angle identity, 2sinθcosθ=sin2θ:
ab=u2sin2θg
Step 5: Solve for the horizontal range
The horizontal range R of the projectile is given by:
R=u2sin2θg
From the previous step, we have ab=u2sin2θg. Therefore, the horizontal range can be expressed as:
R=ab
Final Answer
Thus, the horizontal range of the projectile is:
R=ab
The given equation of the projectile is in the form y=ax−bx2. Here, a represents the slope of the trajectory at the origin, and b is related to the acceleration due to gravity.
In projectile motion, the equation of the trajectory can be compared to the standard form:
y=xtanθ−gx22u2cos2θ
From this, we can identify:
- a=tanθ
- b=g2u2cos2θ
We can find the ratio ab:
ab=tanθg2u2cos2θ=2u2cos2θtanθg
Since tanθ=sinθcosθ, we can rewrite this as:
ab=2u2cos2θ⋅sinθcosθg=2u2sinθcosθg
Using the double angle identity, 2sinθcosθ=sin2θ:
ab=u2sin2θg
The horizontal range R of the projectile is given by:
R=u2sin2θg
From the previous step, we have ab=u2sin2θg. Therefore, the horizontal range can be expressed as:
R=ab
Thus, the horizontal range of the projectile is:
R=ab
Similar Questions
The equation of projectile is y=16x−x24 the horizontal range is:-
View SolutionThe equation of a projectile is y=√3x−(gx22) the horizontal range is
View SolutionThe equation of projectile is y=16x−5x24. Find the horizontal range.
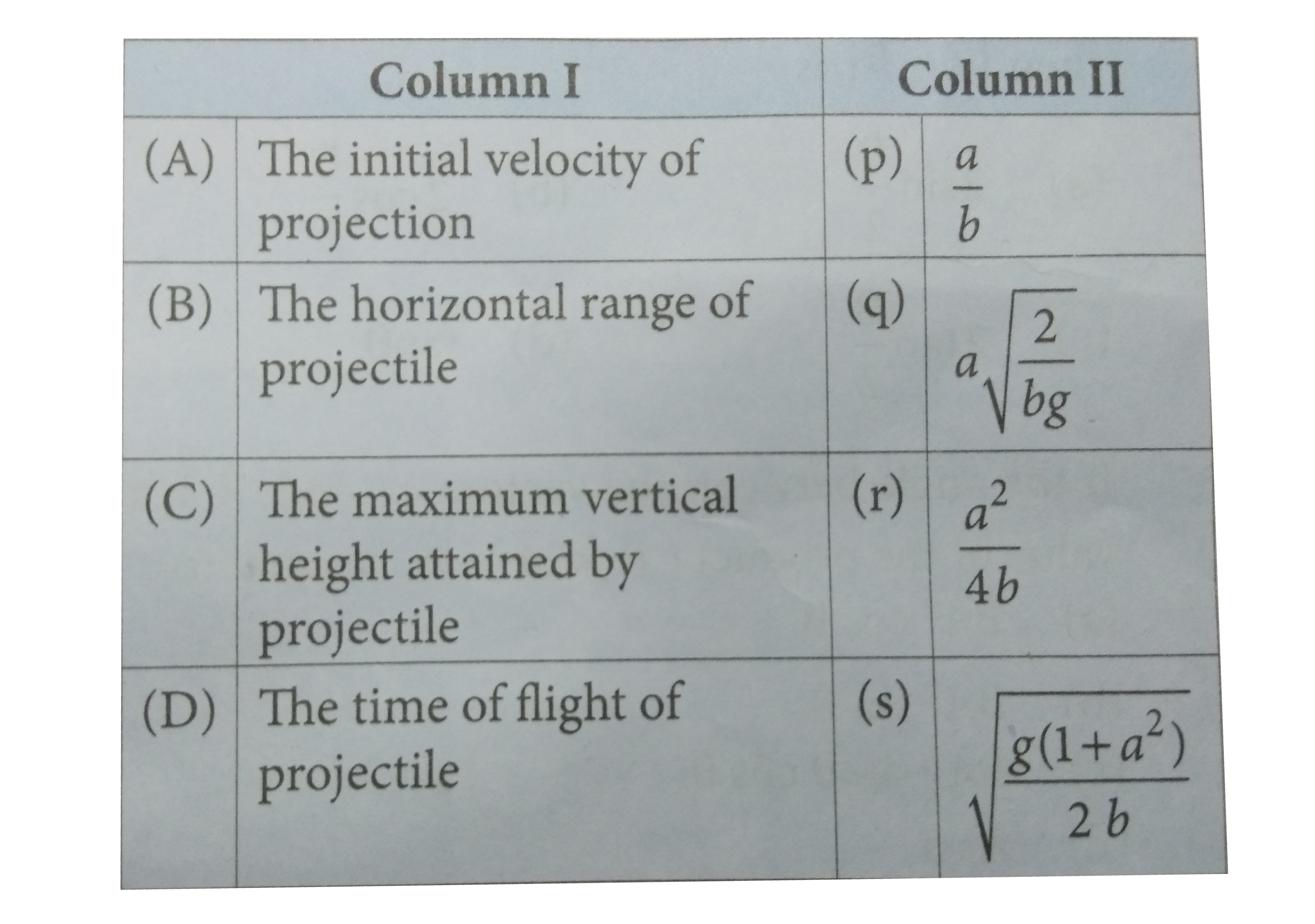
View SolutionThe equation of motion of a projectile is y=ax−bx2, where a and b are constants of motion. Match the quantities in Column I with the relations in Column II.
View SolutionIf the equation of motion of a projectile is y=3x−18x2 the range and maximum height are respectively (y and x are in metres)
View SolutionThe time of flight of a projectile is related to its horizontal range by the equation gT2=2R. The angle of projection is
View SolutionThe equation of motion of a projectile is y=12x−34x2. The horizontal component of velocity is 3ms−1. What is the range of the projectile ?
View SolutionThe equation of motion of a projectile is y=12x−34x2. The horizontal component of velocity is 3ms−1. What is the range of the projectile ?
View SolutionEquation of a projectile is given by y=Px−Qx2 , where P and Q are constants. The ratio of maximum height to the range of the projectile is
View SolutionThe equation of trajectory ofa projectile is y=10x−59x2 If we assume g=10ms−2, the range of projectile (in metre) is
View Solution
