If ΔG for the reaction is A++B−→A2++B2− is x, the ΔG for the reaction is
12A++12B−→12A2++12B2− is
x2
2x
x2
√x
The correct Answer is:A
To solve the problem, we need to analyze the given reactions and their corresponding Gibbs free energy changes (ΔG).
1. Identify the Given Reaction and ΔG:
The first reaction is:
A++B−→A2++B2−
The change in Gibbs free energy (ΔG) for this reaction is given as x.
2. Understand the Relationship Between ΔG and Electrons:
For any redox reaction, the relationship between ΔG and the cell potential (E_cell) is given by:
ΔG=−nFEcell
where n is the number of moles of electrons transferred, F is Faraday's constant, and Ecell is the cell potential.
3. Determine the Number of Electrons Transferred in the First Reaction:
In the first reaction:
- A+ is oxidized to A2+ (loses 1 electron).
- B− is reduced to B2− (gains 1 electron).
Therefore, the total number of electrons transferred n in this reaction is 1.
4. Write the Expression for ΔG of the First Reaction:
From the relationship, we have:
ΔG1=−1⋅F⋅Ecell=x
5. Analyze the Second Reaction:
The second reaction is:
12A++12B−→12A2++12B2−
Here, we need to determine the number of electrons transferred.
6. Determine the Number of Electrons Transferred in the Second Reaction:
In this case:
- 12A+ loses 12 electron.
- 12B− gains 12 electron.
Thus, the total number of electrons transferred n in this reaction is 12.
7. Write the Expression for ΔG of the Second Reaction:
Using the same relationship:
ΔG2=−12⋅F⋅Ecell
8. Relate ΔG of the Second Reaction to the First:
Since we know that ΔG1=x, we can express ΔG2 in terms of x:
ΔG2=−12⋅F⋅Ecell=12ΔG1=12x
9. Final Answer:
Therefore, the change in Gibbs free energy for the second reaction is:
ΔG2=x2
Conclusion:
The answer is x2.
The first reaction is:
A++B−→A2++B2−
The change in Gibbs free energy (ΔG) for this reaction is given as x.
For any redox reaction, the relationship between ΔG and the cell potential (E_cell) is given by:
ΔG=−nFEcell
where n is the number of moles of electrons transferred, F is Faraday's constant, and Ecell is the cell potential.
In the first reaction:
- A+ is oxidized to A2+ (loses 1 electron).
- B− is reduced to B2− (gains 1 electron).
Therefore, the total number of electrons transferred n in this reaction is 1.
From the relationship, we have:
ΔG1=−1⋅F⋅Ecell=x
The second reaction is:
12A++12B−→12A2++12B2−
Here, we need to determine the number of electrons transferred.
In this case:
- 12A+ loses 12 electron.
- 12B− gains 12 electron.
Thus, the total number of electrons transferred n in this reaction is 12.
Using the same relationship:
ΔG2=−12⋅F⋅Ecell
Since we know that ΔG1=x, we can express ΔG2 in terms of x:
ΔG2=−12⋅F⋅Ecell=12ΔG1=12x
Therefore, the change in Gibbs free energy for the second reaction is:
ΔG2=x2
The answer is x2.
Similar Questions
For the reaction
2A+B→C
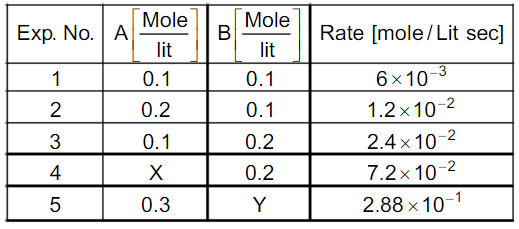
Find X & Y.
View SolutionFor the reaction :
A+2B3+→2B2++A2+
Ec− of the given redox reaction is :
A2++2e−→A Ec−=Xv
B3++e−→B2+ Ec−=yV
View SolutionIf for a reaction A(s)→2B(g),δU+1.2kcaland δS=40calK−1 at 400K then deltaG for the reaction will be (R=2calmol−1K−1
View SolutionFor a reaction A+2B→C, rate is given by R=K[A][B]2. The order of reaction is:
View SolutionIf equilibrium constant for the reaction
A2+B2⇔2AB is k, then for the backward reaction AB⇔1/2A2+1/2B2 the equilibrium constant k' is 1/K.
View SolutionFor the reaction : A+2B→C+D, the expression of rate of reaction will be :
View SolutionThe slowest step of a particular reaction is found to be 1/2X2+Y2→XY2. The order of the reaction is
View SolutionThe mechanism for the overall reaction is
A2+B→C
A2→2A (slow)
2A+B→X (fast)
If a catalyst D changes the mechanism to
A2+D→A2D (slow)
A2D⇒2A+D (fast)
2A+B→C (fast)
Which is the rate expresison for the reaction with and without a catalyst ?
View SolutionProve that : a−1a−1+b−1+a−1a−1−b−1=2b2b2−a2
View SolutionIdentify A and B in the following reaction:
F2+HCl→A+B
View Solution
