At a temperature T K, the pressure of 4.0g argon in a bulb is p. The bulb is put in a bath having temperature higher by 50K than the first one 0.8g of argon gas had to be removed to maintained original pressure. The temperature T is equal to
510 K
200 K
100 K
73 K
To solve the problem step by step, we will use the Ideal Gas Law and the information provided in the question.
Step 1: Understand the Ideal Gas Law
The Ideal Gas Law states that:
PV=nRT
Where:
- P = pressure
- V = volume
- n = number of moles of gas
- R = universal gas constant
- T = temperature in Kelvin
Step 2: Identify the Initial Conditions
Initially, we have:
- Mass of argon = 4.0 g
- Molar mass of argon = 40 g/mol
- Temperature = T K
Calculate the initial number of moles (n1):
n1=massmolar mass=4.0g40g/mol=0.1mol
Step 3: Identify the Final Conditions
When the temperature is increased by 50 K, the new temperature is:
T2=T+50K
After removing 0.8 g of argon, the new mass is:
New mass=4.0g−0.8g=3.2g
Calculate the new number of moles (n2):
n2=3.2g40g/mol=0.08mol
Step 4: Apply the Relationship Between Moles and Temperature
Since the pressure and volume are constant, we can use the relationship:
n1T1=n2T2
Substituting the known values:
(0.1mol)(T)=(0.08mol)(T+50)
Step 5: Solve for T
Expanding the equation:
0.1T=0.08T+4
Rearranging gives:
0.1T−0.08T=4
0.02T=4
Dividing both sides by 0.02:
T=40.02=200K
Final Answer
The temperature T is equal to 200K.
---
The Ideal Gas Law states that:
PV=nRT
Where:
- P = pressure
- V = volume
- n = number of moles of gas
- R = universal gas constant
- T = temperature in Kelvin
Initially, we have:
- Mass of argon = 4.0 g
- Molar mass of argon = 40 g/mol
- Temperature = T K
n1=massmolar mass=4.0g40g/mol=0.1mol
When the temperature is increased by 50 K, the new temperature is:
T2=T+50K
After removing 0.8 g of argon, the new mass is:
New mass=4.0g−0.8g=3.2g
Calculate the new number of moles (n2):
n2=3.2g40g/mol=0.08mol
Since the pressure and volume are constant, we can use the relationship:
n1T1=n2T2
Substituting the known values:
(0.1mol)(T)=(0.08mol)(T+50)
Expanding the equation:
0.1T=0.08T+4
Rearranging gives:
0.1T−0.08T=4
0.02T=4
Dividing both sides by 0.02:
T=40.02=200K
The temperature T is equal to 200K.
Topper's Solved these Questions
- STATES OF MATTERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ASSIGNMENT (SECTION-C)
29 Videos
- STATES OF MATTERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ASSIGNMENT (SECTION-D)
14 Videos
- STATES OF MATTERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ASSIGNMENT (SECTION-A)
50 Videos
- SOME BASIC CONCEPTS OF CHEMISTRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - ASSIGNMENT SECTION J (Aakash Challenges Questions)
10 Videos
- STRUCTURE OF ATOMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - ASSIGNMENT (SECTION -D) Assertion-Reason Type Questions
15 Videos
Similar Questions
At a temperature T,K, the pressure of 4.0gm argon in a bulb is P. The bulb is put in a bath having temperature higher by 50K than the first one 0.8 of argon gas had to be removed to maintain original pressure. The temperature T is
View Solution4.0g of argon gas has pressure P and temperature TK in a vessel. On keeping the vessel at 50∘C higher, 0.8g of argon was given out to maintain the pressure at P. The original temperature was ____×10−2K.
View SolutionPressure of a gas at S.T.P. is doubled and the temperature is raised to 546 K. What is the final volume of the gas ?
View SolutionAt 273K temp, and 9 atm pressure, the compressibility fog a gas is 0.9. The volume of 1 mill-moles of gas at this temperature and pressure is :
View Solution4 g of an ideal gass (Vapour dencity=20) is taken in a bulb of 10dm3volume at a temperature of T K. The bulb is placed in a thermostat maintained at a temperature 125°C more than the initial. To maintain original pressure, 0.8 g of gas has to be removed, then the value of T is
View Solution4gof an ideal has (vapourdensely=20) is taken in abulbe od 10dm3 volume, at a temperature ofT K.The bulb is present on thermostat maintained at a temperature 125°C more than the initial. To maintain original pressure, 0.8 g of gas has to be removed, then the value of T is
View SolutionTwo identical glass bulbs are interconnected by a thin glass tube. A gas is filled in these bulbs at N.T.P. If one bulb is placed in ice and another bulb is placed in hot bath, then the pressure of the gas becomes 1.5 times. The temperature of hot bath will be
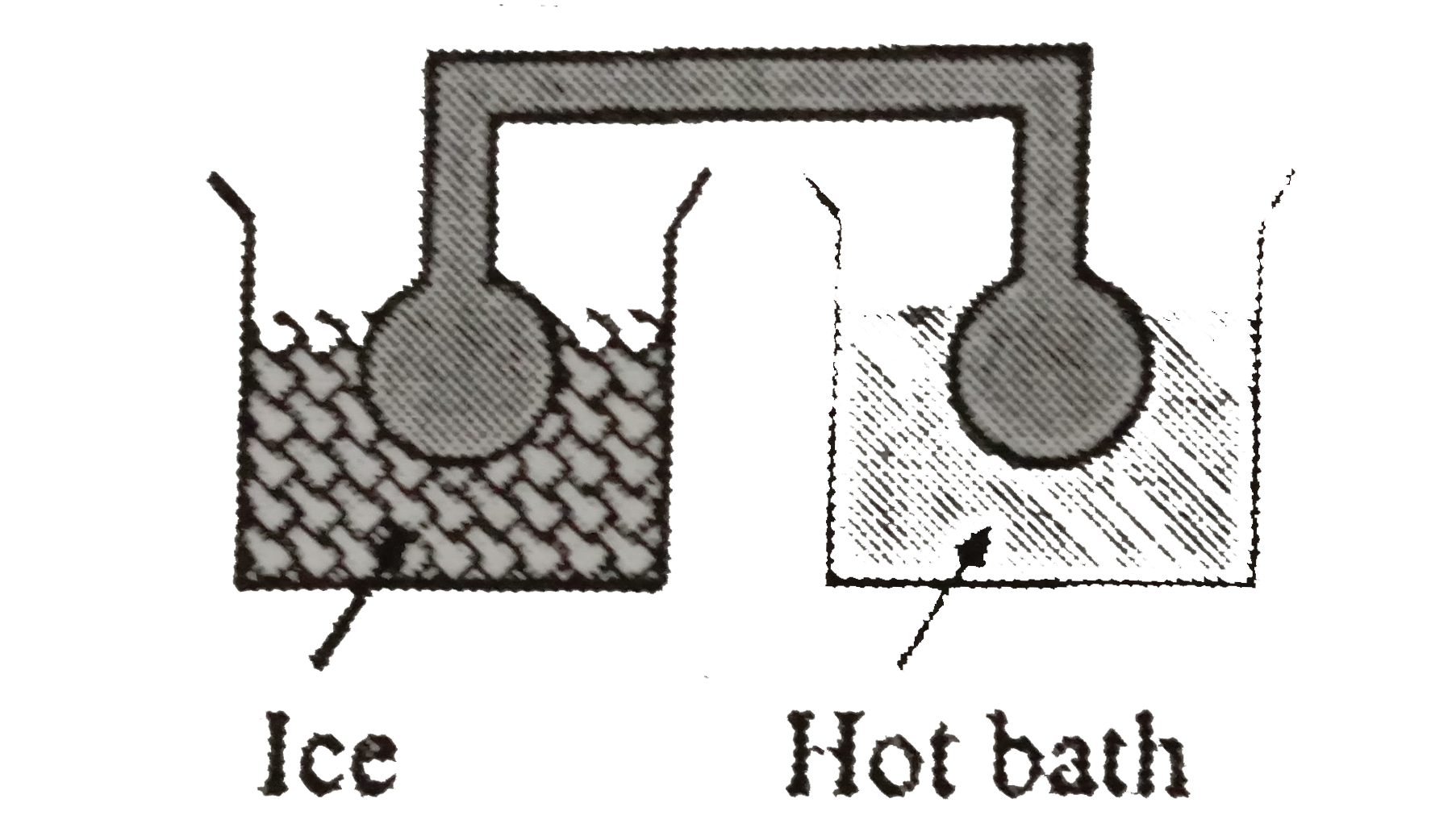
View SolutionThe pressure of a gas filled in the bulb of a constant volume gas thermometer at temperatures 0°Cand100°C are 27.50 cm and 37.50 cm of Hg respectively. At an unknown temperature the pressure is 32.45 cm of Hg. Unknown temperature is -
View Solution300K a gas (γ=5/3) is compressed adiabatically so that its pressure becomes 1/8 of the original pressure. The final temperature of the gas is :
View SolutionThe equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, will be
View Solution
