To move a body along a circular path the direction of centripetal force will be
Towards the centre
Away from the centre
Along tangent
Normal to the plane of motion
The correct Answer is:A
To determine the direction of centripetal force required to move a body along a circular path, we can follow these steps:
1. Understanding Circular Motion:
- When an object moves in a circular path, it is constantly changing direction. This change in direction requires a force to act on the object.
2. Definition of Centripetal Force:
- Centripetal force is the force that keeps an object moving in a circular path. It acts towards the center of the circle.
3. Direction of Centripetal Force:
- The centripetal force always acts along the radius of the circular path and is directed towards the center of the circle. This is crucial for maintaining circular motion.
4. Relationship with Velocity:
- The velocity of the object is tangential to the circular path, meaning it is perpendicular to the radius at any point. The centripetal force does not change the speed of the object; it only changes the direction of the velocity.
5. Mathematical Expression:
- The magnitude of centripetal force (F_c) can be expressed as:
Fc=mv2r
where m is the mass of the object, v is its speed, and r is the radius of the circular path.
6. Conclusion:
- Therefore, the direction of centripetal force is always towards the center of the circular path.
Final Answer:
The direction of centripetal force is towards the center of the circle.
---
- When an object moves in a circular path, it is constantly changing direction. This change in direction requires a force to act on the object.
- Centripetal force is the force that keeps an object moving in a circular path. It acts towards the center of the circle.
- The centripetal force always acts along the radius of the circular path and is directed towards the center of the circle. This is crucial for maintaining circular motion.
- The velocity of the object is tangential to the circular path, meaning it is perpendicular to the radius at any point. The centripetal force does not change the speed of the object; it only changes the direction of the velocity.
- The magnitude of centripetal force (F_c) can be expressed as:
Fc=mv2r
where m is the mass of the object, v is its speed, and r is the radius of the circular path.
- Therefore, the direction of centripetal force is always towards the center of the circular path.
The direction of centripetal force is towards the center of the circle.
Topper's Solved these Questions
Similar Questions
If the speed and radius both are trippled for a body moving on a circular path, then the new centripetal force will be:
View SolutionThe expression from centripetal force depends upon mass of body, speed of the body and the radius of circular path. Find the expression for centripetal force.
View SolutionA particle moving along a circular path due to a centripetal force having constant magnitude is an example of motion with :
View SolutionA particle of mass m moves along a circular path of radius r with a centripetal acceleration an changing with time t as an=kt2, where k is a positive constant. The average power developed by all the forces acting on the particle during the first t0 seconds is
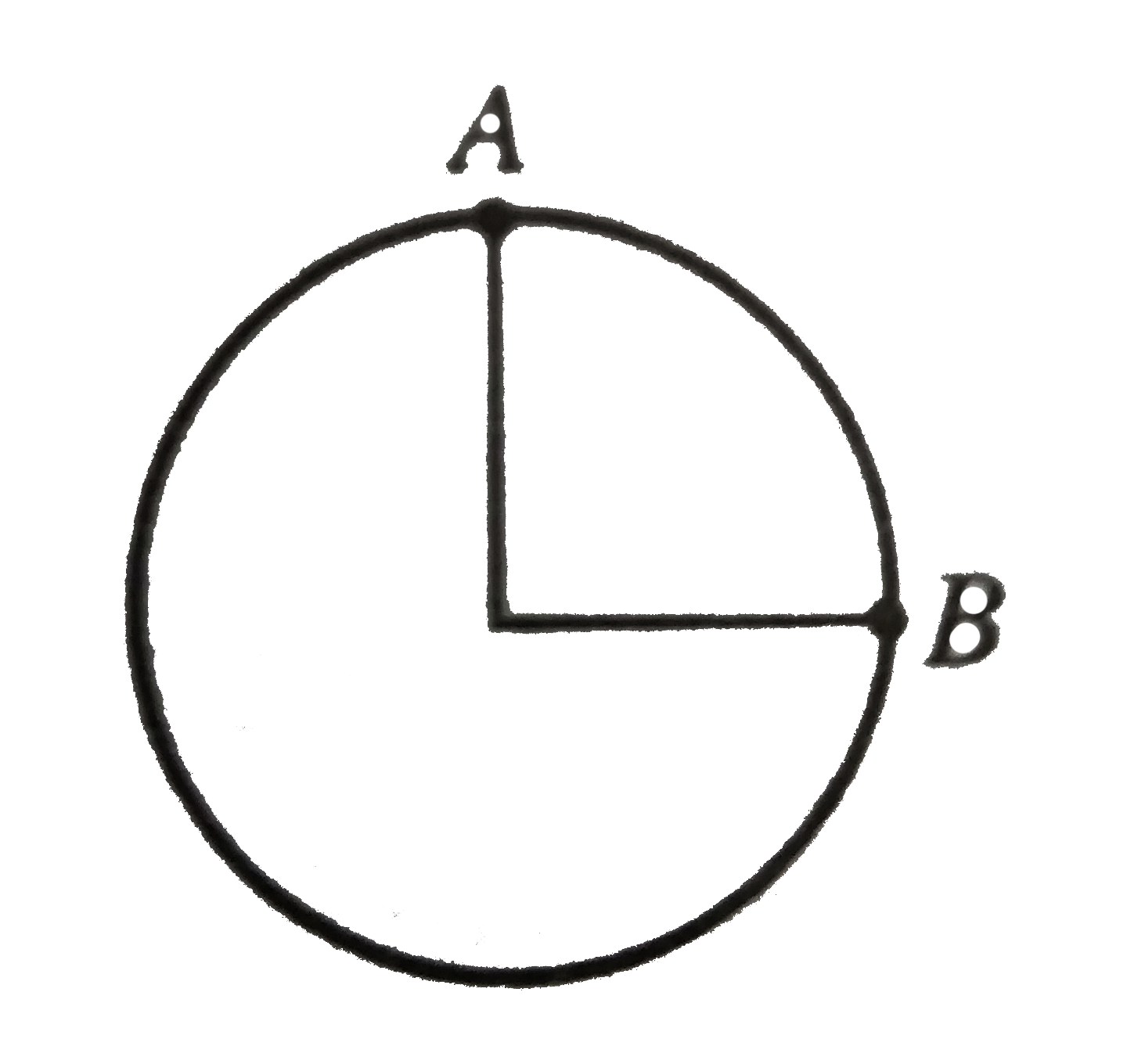
View SolutionA particle is moving in a circular path of radius 1 m. under the action of a centripetal force, the speed √2πms−1 of the particle is constant. Find the average of the velocity (inms−1) between A and B.
View SolutionA particle moves in a circular path of radius (R). If centripetal force (F) is kept constant but the angular velocity is double, the new radius of the path will be
View SolutionA : When a body moves in a circle the work done by the centripetal force is always zero.
R : Centripetal force is perpendicular to displacement at every instant.
View SolutionA particle of mass M moves with constant speed along a circular path of radius r under the action of a force F. Its speed is
View SolutionStatement-I : When the direction of motion of a particle moving in a circular path is reversed the direction of radial acceleration still remains the same (at the given point).
Statement-II : Particle revolves on circular path in any direction such as clockwise or anticlockwise the direction of radius accelerationis always towards the centre of the circular path.
View SolutionTwo particles of masses m and 3m are moving under their mutual gravitational force, around their centre of mass, in circular orbits. The separation between the masses is r. The gravitational attraction the two provides nessary centripetal force for circular motion
The ratio of centripetal forces acting on the two masses will be
View Solution
