A disc is rolling without slipping. The ratio of its rotational kinetic energy and translational kinetic energy would be -
1:2
2:1
1:1
1:4
The correct Answer is:A
To find the ratio of the rotational kinetic energy to the translational kinetic energy of a disc rolling without slipping, we can follow these steps:
1. Identify the Kinetic Energies:
- The translational kinetic energy (TKE) of the disc is given by the formula:
TKE=12mv2
- The rotational kinetic energy (RKE) of the disc is given by the formula:
RKE=12Iω2
where I is the moment of inertia and ω is the angular velocity.
2. Moment of Inertia for a Disc:
- The moment of inertia I for a solid disc about its center is:
I=12mr2
3. Relationship Between Linear and Angular Velocity:
- For a disc rolling without slipping, the relationship between the linear velocity v and the angular velocity ω is:
v=rω⇒ω=vr
4. Substituting ω in RKE:
- Substitute ω into the RKE formula:
RKE=12Iω2=12(12mr2)(vr)2
- Simplifying this gives:
RKE=12⋅12mr2⋅v2r2=14mv2
5. Finding the Ratio:
- Now, we can find the ratio of RKE to TKE:
Ratio=RKETKE=14mv212mv2
- The mv2 terms cancel out:
Ratio=1412=14⋅21=12
6. Final Result:
- Therefore, the ratio of the rotational kinetic energy to the translational kinetic energy is:
Ratio=1:2
Conclusion:
The ratio of the rotational kinetic energy to the translational kinetic energy of a disc rolling without slipping is 1:2.
---
- The translational kinetic energy (TKE) of the disc is given by the formula:
TKE=12mv2
- The rotational kinetic energy (RKE) of the disc is given by the formula:
RKE=12Iω2
where I is the moment of inertia and ω is the angular velocity.
- The moment of inertia I for a solid disc about its center is:
I=12mr2
- For a disc rolling without slipping, the relationship between the linear velocity v and the angular velocity ω is:
v=rω⇒ω=vr
- Substitute ω into the RKE formula:
RKE=12Iω2=12(12mr2)(vr)2
- Simplifying this gives:
RKE=12⋅12mr2⋅v2r2=14mv2
- Now, we can find the ratio of RKE to TKE:
Ratio=RKETKE=14mv212mv2
- The mv2 terms cancel out:
Ratio=1412=14⋅21=12
- Therefore, the ratio of the rotational kinetic energy to the translational kinetic energy is:
Ratio=1:2
The ratio of the rotational kinetic energy to the translational kinetic energy of a disc rolling without slipping is 1:2.
Topper's Solved these Questions
Similar Questions
A ring is rolling without slipping. The ratio of translational kinetic energy to rotational kinetic energy is
View SolutionThe radius of a wheel is R and its radius of gyration about its axis passing through its center and perpendicualr to its plane is K. If the wheel is roling without slipping. Then the ratio of tis rotational kinetic energy to its translational kinetic energy is
View SolutionThe moment of inertia of a solid cylinder about its axis is given by (1/2)MR2. If this cylinder rolls without slipping the ratio of its rotational kinetic energy to its translational kinetic energy is -
View SolutionA solid sphere is rolling without slipping on a horizontal plane. The ratio of its rotational kinetic energy and translational kinetic energy is
View SolutionA hollow sphere is rolling without slipping on a rough surface. The ratio of translational kinetic energy to rotational kinetic energy is
View SolutionWhen a sphere rolls without slipping the ratio of its kinetic energy of translation to its total kinetic energy is.
View SolutionA disc is rolling on a surface without slipping. What is the ratio of its translational to rotational kinetic energies ?
View SolutionA circular disc rolls down an inclined plane . The ratio of rotational kinetic energy to total kinetic energy is
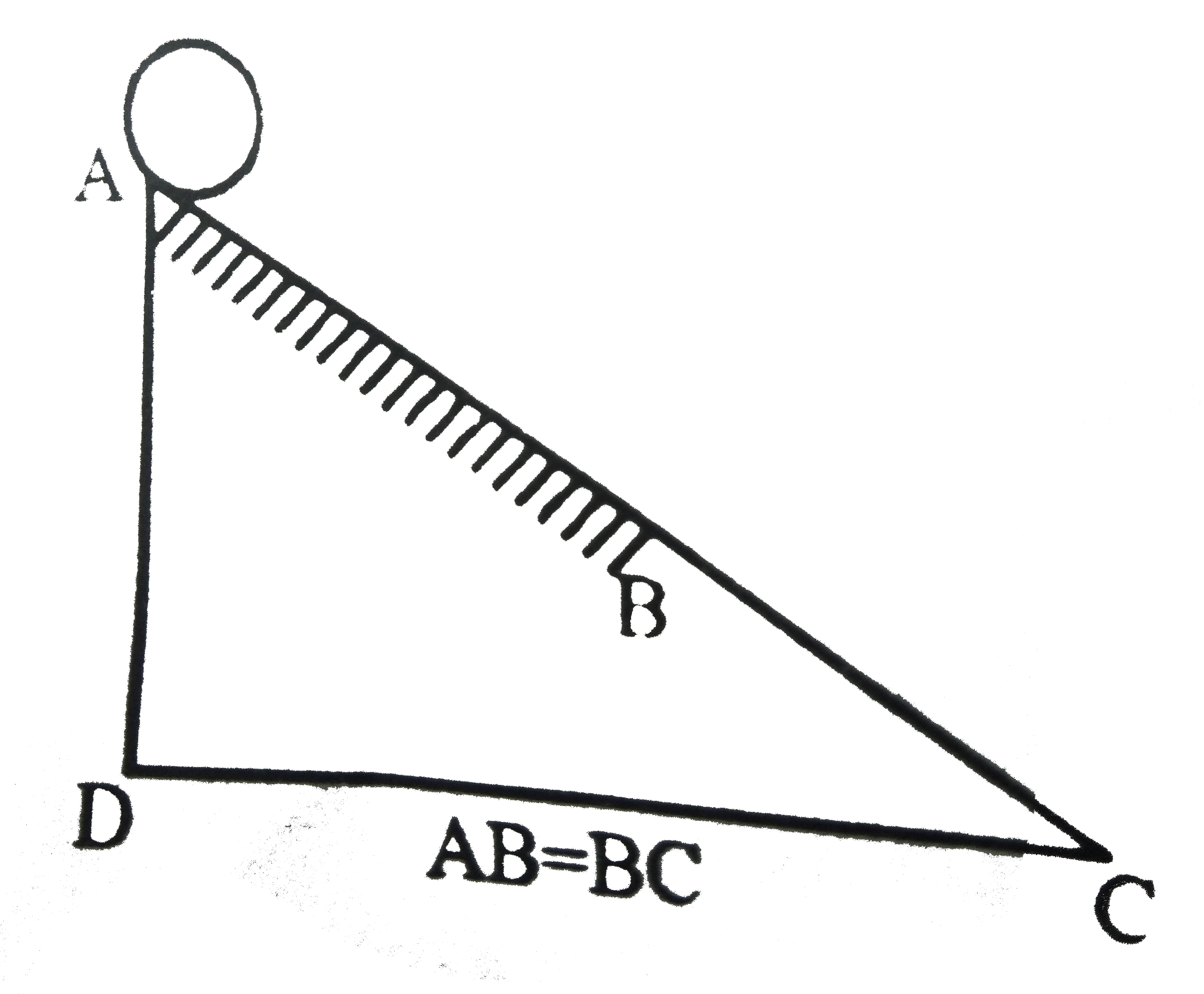
View SolutionPortion AB of the wedge shown in figure is rough and BC is smooth. A solid cylinder rolld without slipping from A to B. Find the ratio of translational kinetic energy to rotational kinetic energy, when the cylinder reaches point C.
 .
.
View SolutionIf a body is rolling on a surface without slipping such that its kinetic energy of translation is equal to kinetic energy of rotation then it is a
View Solution
