If the values of stepwise stability constants for a series of successive reactions are logK1=4,logK2=3.2,logK3=2.7andlogK4=2, what is the value ofβ4?
11.9
7.94 X 1011
1.47 X 105
27.4
To find the value of β4 given the stepwise stability constants logK1=4,logK2=3.2,logK3=2.7, and logK4=2, we can follow these steps:
Step 1: Understand the relationship between stability constants and overall stability constant
The overall stability constant βn for a complex formation reaction can be calculated as the product of the stepwise stability constants:
βn=K1×K2×K3×…×Kn
In logarithmic terms, this relationship can be expressed as:
logβn=logK1+logK2+logK3+…+logKn
Step 2: Substitute the given values into the equation
For β4, we need to sum the logarithms of the first four stability constants:
logβ4=logK1+logK2+logK3+logK4
Substituting the given values:
logβ4=4+3.2+2.7+2
Step 3: Calculate the sum
Now, let's calculate the sum:
logβ4=4+3.2+2.7+2=11.9
Step 4: Find β4 by taking the antilogarithm
To find β4, we take the antilogarithm of logβ4:
β4=1011.9
Step 5: Calculate the value of β4
Using a calculator, we find:
β4≈7.94×1011
Thus, the value of β4 is approximately 7.94×1011.
Summary of the Solution
1. Use the relationship between stability constants and overall stability constant.
2. Substitute the given logarithmic values into the equation.
3. Calculate the sum of the logarithmic values.
4. Take the antilogarithm to find β4.
5. Calculate the final value.
The overall stability constant βn for a complex formation reaction can be calculated as the product of the stepwise stability constants:
βn=K1×K2×K3×…×Kn
In logarithmic terms, this relationship can be expressed as:
logβn=logK1+logK2+logK3+…+logKn
For β4, we need to sum the logarithms of the first four stability constants:
logβ4=logK1+logK2+logK3+logK4
Substituting the given values:
logβ4=4+3.2+2.7+2
Now, let's calculate the sum:
logβ4=4+3.2+2.7+2=11.9
To find β4, we take the antilogarithm of logβ4:
β4=1011.9
Using a calculator, we find:
β4≈7.94×1011
1. Use the relationship between stability constants and overall stability constant.
2. Substitute the given logarithmic values into the equation.
3. Calculate the sum of the logarithmic values.
4. Take the antilogarithm to find β4.
5. Calculate the final value.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If 4x2y=4, what is the value of 3yx ?
A34B43C32D2 - Question 2 - Select One
What is the value of (2+8i)(1-4i)-(3-2i)(6+4i)?
A8B26C34D50 - Question 3 - Select One
What is the value of (2x2+4x+8)-(2x^2-4x+7)?
A4x2+8x+15B2x2+x+1C8x+1D8x+15
If 1x+1y=2 and 3x+4y=7 , what is the value of (x + y) ?
View Solution2(p+3)−74=16−2(3−p)6
In the equation above , what is the value of p ?View SolutionThe temperature dependence of the rate constant k is expressed as k=Ae−Ea/RT
When a plot between logk and 1/T is plotted we get the graph as shown.
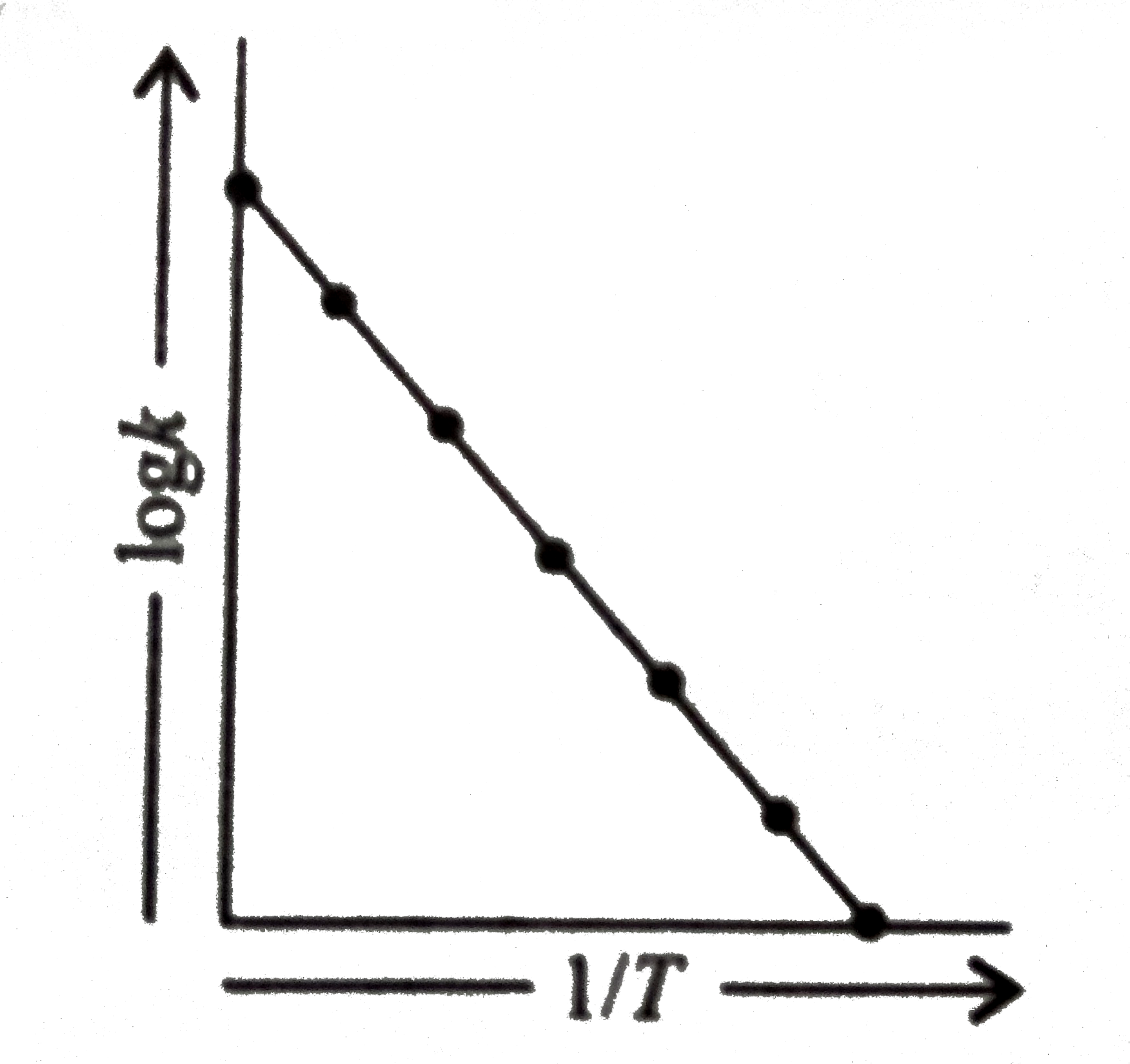
What is the value of slopw in the graph?View SolutionA function a satisfies a(−2)=3anda(3)=8.A function b satisfies b(3)=4andb(7)=−2. What is the value of a(b(7)) ?
View SolutionDetermine the product of the reaction:
.73Li+.42He→?+n
What is the Q value of the reaction?View Solution

