There are some identical bulbs of 50 W, 200 V are connected in parallel across 200 V source using 5 A fuse. How many maximum bulbs can be operated safely among the following options?
19
21
40
15
To solve the problem step by step, we will calculate how many identical bulbs can be safely operated when connected in parallel across a 200 V source with a 5 A fuse.
Step 1: Understand the Power and Voltage Ratings
The power rating of each bulb is given as 50 W, and the voltage rating is 200 V. When connected in parallel, the voltage across each bulb remains the same as the source voltage, which is 200 V.
Step 2: Calculate the Current Drawn by Each Bulb
Using the formula for power:
P=V×I
where:
- P is the power (in watts),
- V is the voltage (in volts),
- I is the current (in amperes).
Rearranging the formula to find the current:
I=PV
Substituting the values:
I=50 W200 V=14 A
This means each bulb draws 0.25 A of current.
Step 3: Determine the Maximum Current Allowed by the Fuse
The fuse can handle a maximum current of 5 A. This means that the total current drawn by all the bulbs connected in parallel must not exceed 5 A.
Step 4: Calculate the Maximum Number of Bulbs
Let n be the number of bulbs that can be safely connected. The total current drawn by n bulbs is:
Total Current=n×(14 A)
Setting this equal to the maximum current allowed by the fuse:
n×14 A≤5 A
To find n, we rearrange the equation:
n≤5 A×4=20
Step 5: Conclusion
The maximum number of bulbs that can be safely operated is n=20.
However, since the question asks for the maximum number of bulbs that can be operated safely among the given options, we need to check the options provided. If 20 is not an option, we would select the highest available option below 20.
Final Answer
The maximum number of bulbs that can be operated safely is 20.
---
The power rating of each bulb is given as 50 W, and the voltage rating is 200 V. When connected in parallel, the voltage across each bulb remains the same as the source voltage, which is 200 V.
Using the formula for power:
P=V×I
where:
- P is the power (in watts),
- V is the voltage (in volts),
- I is the current (in amperes).
I=PV
I=50 W200 V=14 A
The fuse can handle a maximum current of 5 A. This means that the total current drawn by all the bulbs connected in parallel must not exceed 5 A.
Let n be the number of bulbs that can be safely connected. The total current drawn by n bulbs is:
Total Current=n×(14 A)
n×14 A≤5 A
n≤5 A×4=20
The maximum number of bulbs that can be safely operated is n=20.
The maximum number of bulbs that can be operated safely is 20.
Similar Questions
Two bulbs 100W,250V and 200W,250V are connected in parallel across a 500V line. Then-
View SolutionThree bulbs of 40W,60Wand100W are connected in series with a 240V source.
View SolutionWhen three identical bulbs of 60W,200V rating are connected in series to a 200V supply, the power drawn by them will be
View SolutionThree identical bulbs are connected in parallel across an ideal sources of emf E .The ammeter A and voltmeter V are ideal . If bulb B3 gets fused, then
_PHY_E05_094_Q01.png)
View SolutionTwo identical bulbs are conneted in parallel across an ideal source of emf E . The ammeter A voltmeter V are ideal . If bulb B2 gets fused , then
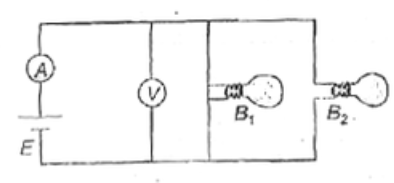 .
.
View SolutionAn electric bulb marked 25 W and 100 V is connected across 50 V supply . Now its power is .
View SolutionTwo bulbs 60 W and 100 W designed for voltage 220 V are connected in series across 220 V source. The net power dissipated is
View SolutionIf two bulbs of 25Wand100W rated at 220V are connected in series across a 440V supply, will both the bulbs fuse ? If not which one ?
View SolutionSome electric bulbs are connected in series across a 220 V supply in a room. If one bulb is fused then remaining bulbs are connected again in series across the same supply. The illumination in the room will
View SolutionIf two bulbs of 25 W and 100 W rated at 200 volts are connected in series across a 440 volts supply, then
View Solution