A force F=(xˆi+2yˆj)N is applied on an object of mass 10 kg, Force displaces the object from position A(1, 0) m to position B(3, 3) m then the work done by the force is (x and y are meter)
8 J
5 J
13 J
16 J
The correct Answer is:C
To solve the problem of calculating the work done by the force F=(x^i+2y^j) N as it displaces an object from position A(1,0) m to position B(3,3) m, we will follow these steps:
Step 1: Understand the Force and Displacement
The force is given as F=x^i+2y^j. The displacement vector ds can be expressed in terms of its components as ds=dx^i+dy^j.
Step 2: Set Up the Work Done Integral
The work done W by a variable force is calculated using the integral:
W=∫F⋅ds
Substituting for F and ds:
W=∫(x^i+2y^j)⋅(dx^i+dy^j)
Step 3: Calculate the Dot Product
Calculating the dot product:
F⋅ds=xdx+2ydy
Thus, the work done becomes:
W=∫(xdx+2ydy)
Step 4: Determine the Path of Integration
Since the force is variable, we need to express y in terms of x or vice versa. The path from A(1,0) to B(3,3) can be described as y=32(x−1) (a straight line from A to B).
Step 5: Express dy in Terms of dx
Differentiating y=32(x−1):
dy=32dx
Step 6: Substitute y and dy into the Work Integral
Now we can substitute y and dy into the work integral:
W=∫31(xdx+2(32(x−1))(32dx))
This simplifies to:
W=∫31(xdx+92(x−1)dx)
Step 7: Combine the Integrals
Combine the integrals:
W=∫31(x+92(x−1))dx=∫31(x+92x−92)dx
=∫31(112x−92)dx
Step 8: Evaluate the Integral
Now, evaluate the integral:
W=(114x2−92x)31
Calculating the limits:
W=(114(32)−92(3))−(114(12)−92(1))
=(11⋅94−272)−(114−92)
=(994−544)−(114−184)
=454−(−74)=45+74=524=13J
Final Answer
The work done by the force is 13J.
---
The force is given as F=x^i+2y^j. The displacement vector ds can be expressed in terms of its components as ds=dx^i+dy^j.
The work done W by a variable force is calculated using the integral:
W=∫F⋅ds
Substituting for F and ds:
W=∫(x^i+2y^j)⋅(dx^i+dy^j)
Calculating the dot product:
F⋅ds=xdx+2ydy
Thus, the work done becomes:
W=∫(xdx+2ydy)
Since the force is variable, we need to express y in terms of x or vice versa. The path from A(1,0) to B(3,3) can be described as y=32(x−1) (a straight line from A to B).
Differentiating y=32(x−1):
dy=32dx
Now we can substitute y and dy into the work integral:
W=∫31(xdx+2(32(x−1))(32dx))
This simplifies to:
W=∫31(xdx+92(x−1)dx)
Combine the integrals:
W=∫31(x+92(x−1))dx=∫31(x+92x−92)dx
=∫31(112x−92)dx
Now, evaluate the integral:
W=(114x2−92x)31
Calculating the limits:
W=(114(32)−92(3))−(114(12)−92(1))
=(11⋅94−272)−(114−92)
=(994−544)−(114−184)
=454−(−74)=45+74=524=13J
The work done by the force is 13J.
Similar Questions
A force bar F = (3hat i-4 hat j +b hat k)N is acting on the particle which is moving from point A ( 0-1, 1) m to the point B(2, 2 3) m If net work done by the force on the particle is zero then value of b is
View SolutionA force →F=(5ˆi+3ˆj)N is applied over a particle which displaces it from its origin to the point →r=(2ˆi−1ˆj) meter. The work done on the particle is :
View SolutionA uniform force of (3ˆi+ˆj) newton acts on a particle of mass 2kg. Hence the particle is displaced from position (2ˆi+ˆk) meter to position (4ˆi+3ˆj−ˆk) meter. The work done by the force on the particle is :
View SolutionAn object is displaced from a point A(0, 0, 0) to B(1m, 1m , 1m) under a force →F=(yˆi+xˆj)N. Find the work done by this force in this process.
View SolutionWork done by a force F on a body is W = F .s, where s is the displacement of body. Given that under a force F=(2ˆi+3ˆj+4ˆk) N a body is displaced from position vector r1=(2ˆi+3ˆj+ˆk) m to the position vector r2=(ˆi+ˆj+ˆk) m. Find the work done by this force.
View SolutionWork done by a force F on a body is W = F .s, where s is the displacement of body. Given that under a force F=(2ˆI+3ˆj+4ˆk) N a body is displaced from position vector r1=(2ˆI+3ˆj+ˆk) m to the position vector r2=(ˆi+ˆj+ˆk) m. Find the work done by this force.
View SolutionA particle acted by constant forces 4ˆi+ˆj−3ˆkand3ˆi+ˆ9j−ˆk is displaced from point ˆi+2ˆj+3ˆk to point 5ˆi+4ˆj+ˆk. find the total work done by the forces in units.
View SolutionIn each of the following, a force, F is acting on an object of mass, m. The direction of displacement is from west to east shown by the longer arrow. Observe the diagrams carefully and state whether the work done by the force is negative, positive or zero.
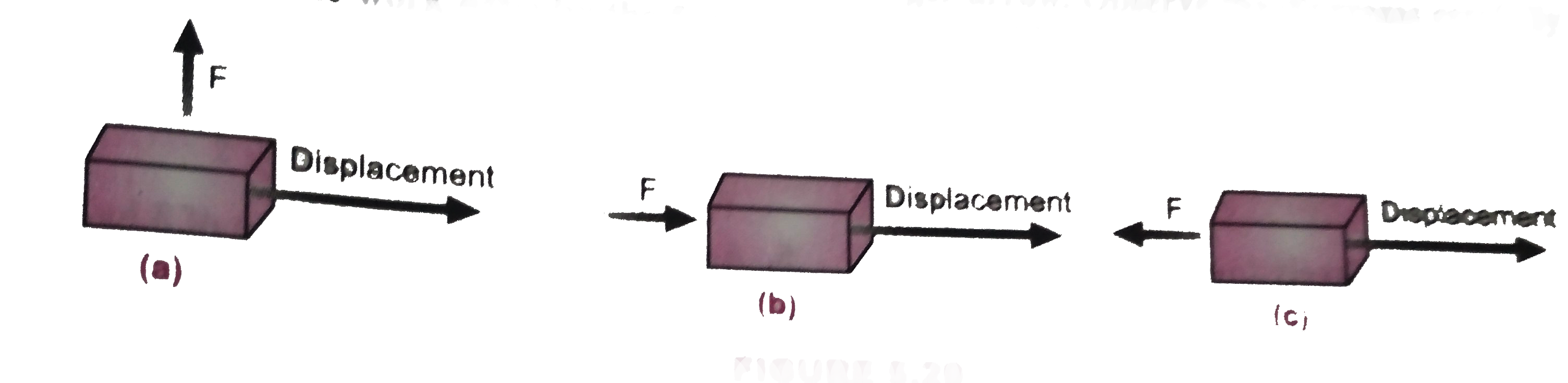
View SolutionA force F acting on a particle varies with the position x as shown in figure. Find the work done by this force in displacing the particle from

View SolutionA force (3ˆi+4ˆj) newton acts on a body and displaces it by (3ˆI+4ˆj) metere. The work done by the force is y Joules. Find y.
View Solution