An element of length →Δl=ΔzˆK is placed at origin and carries a large current l=15A. The magnetic field on the y axis at a distance of 0.75 m is (Δz=0.75cm)
2×10−8 T along negative x-axis
2×10−8T along positive x-axis
Zero
2×10−8T along negative y-axis
To solve the problem step by step, we will calculate the magnetic field generated by a current-carrying wire at a specific point in space.
Step 1: Understand the given information
- The element of length is given as Δ→l=Δz^k.
- The current I=15A.
- The distance from the wire to the point on the y-axis is r=0.75m.
- The length element Δz is given as 0.75cm=0.0075m.
Step 2: Identify the formula for the magnetic field
The magnetic field dB due to a current element is given by the Biot-Savart Law:
dB=μ04πId→l×^rr2
where:
- μ0=4π×10−7T m/A (permeability of free space),
- d→l is the current element vector,
- ^r is the unit vector from the current element to the point where the field is being calculated,
- r is the distance from the current element to the point.
Step 3: Calculate the unit vector ^r
Since the current element is along the z-axis and the point is on the y-axis, the position of the point can be represented as:
- Current element at the origin: (0,0,0)
- Point on the y-axis: (0,0.75,0)
The vector →r from the current element to the point is:
→r=(0−0,0.75−0,0−0)=(0,0.75,0)
The magnitude of r is:
r=0.75m
The unit vector ^r is:
^r=→rr=(0,1,0)=^j
Step 4: Calculate d→l×^r
Given d→l=Δz^k=0.0075^k, we compute the cross product:
d→l×^r=(0.0075^k)×(1^j)=0.0075(^k×^j)
Using the right-hand rule, ^k×^j=−^i:
d→l×^r=−0.0075^i
Step 5: Substitute values into the magnetic field formula
Now we substitute into the Biot-Savart Law:
dB=μ04πI(−0.0075^i)(0.75)2
Substituting the values:
dB=4π×10−74π15×(−0.0075)0.5625^i
This simplifies to:
dB=10−715×(−0.0075)0.5625^i
Calculating the numerical part:
=10−7−0.11250.5625^i=10−7×−0.2^i=−2×10−8^i
Step 6: Final result
The magnitude of the magnetic field is:
B=2×10−8T
The direction of the magnetic field is along the negative x-axis, represented as −^i.
Conclusion
The final answer is:
B=2×10−8T along the negative x-axis
- The element of length is given as Δ→l=Δz^k.
- The current I=15A.
- The distance from the wire to the point on the y-axis is r=0.75m.
- The length element Δz is given as 0.75cm=0.0075m.
The magnetic field dB due to a current element is given by the Biot-Savart Law:
dB=μ04πId→l×^rr2
where:
- μ0=4π×10−7T m/A (permeability of free space),
- d→l is the current element vector,
- ^r is the unit vector from the current element to the point where the field is being calculated,
- r is the distance from the current element to the point.
Since the current element is along the z-axis and the point is on the y-axis, the position of the point can be represented as:
- Current element at the origin: (0,0,0)
- Point on the y-axis: (0,0.75,0)
→r=(0−0,0.75−0,0−0)=(0,0.75,0)
The magnitude of r is:
r=0.75m
The unit vector ^r is:
^r=→rr=(0,1,0)=^j
Given d→l=Δz^k=0.0075^k, we compute the cross product:
d→l×^r=(0.0075^k)×(1^j)=0.0075(^k×^j)
Using the right-hand rule, ^k×^j=−^i:
d→l×^r=−0.0075^i
Now we substitute into the Biot-Savart Law:
dB=μ04πI(−0.0075^i)(0.75)2
Substituting the values:
dB=4π×10−74π15×(−0.0075)0.5625^i
This simplifies to:
dB=10−715×(−0.0075)0.5625^i
Calculating the numerical part:
=10−7−0.11250.5625^i=10−7×−0.2^i=−2×10−8^i
The magnitude of the magnetic field is:
B=2×10−8T
The direction of the magnetic field is along the negative x-axis, represented as −^i.
The final answer is:
B=2×10−8T along the negative x-axis
Similar Questions
An element d→l=dxˆi (where dx=1cm) is placed at the origin and carries a large current i=10A. What is the magnetic field on the Y-axis at a distance of 0.5m?
View SolutionAn element d→l=dxˆi (where dx=1cm) is placed at the origin and carries a large current i=10A. What is the magnetic field on the Y-axis at a distance of 0.5m?
View SolutionAn element of 0.05ˆim is placed at the origin as shown in figure which carries a large current of 10 A. The magnetic field at a distance of 1 m in perpendicular direction is

View SolutionA solenoid of length 10 cm and radius 1 cm contains 200 turns and carries a current of 10 A. Find the magnetic field at a point on the axis at a distance of 10cm from the centre.
View SolutionA long straight wire is carrying a current of 12 A . The magnetic field at a distance of 8 cm is
(μ0=4π×10−7NA2)
View SolutionA thin solenoid of length 0.4m and having 500 turns of wire carries a current 1A,then find the magnetic field on the axis inside the solenoid.
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionAB and CD are long straight conductor, distance d apart, carrying a current l. The magnetic field at the midpoint of BC is

View SolutionA long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
View SolutionA wire of infinite length bent as shown in figure carries current l. What is the magnetic field at the point P ?
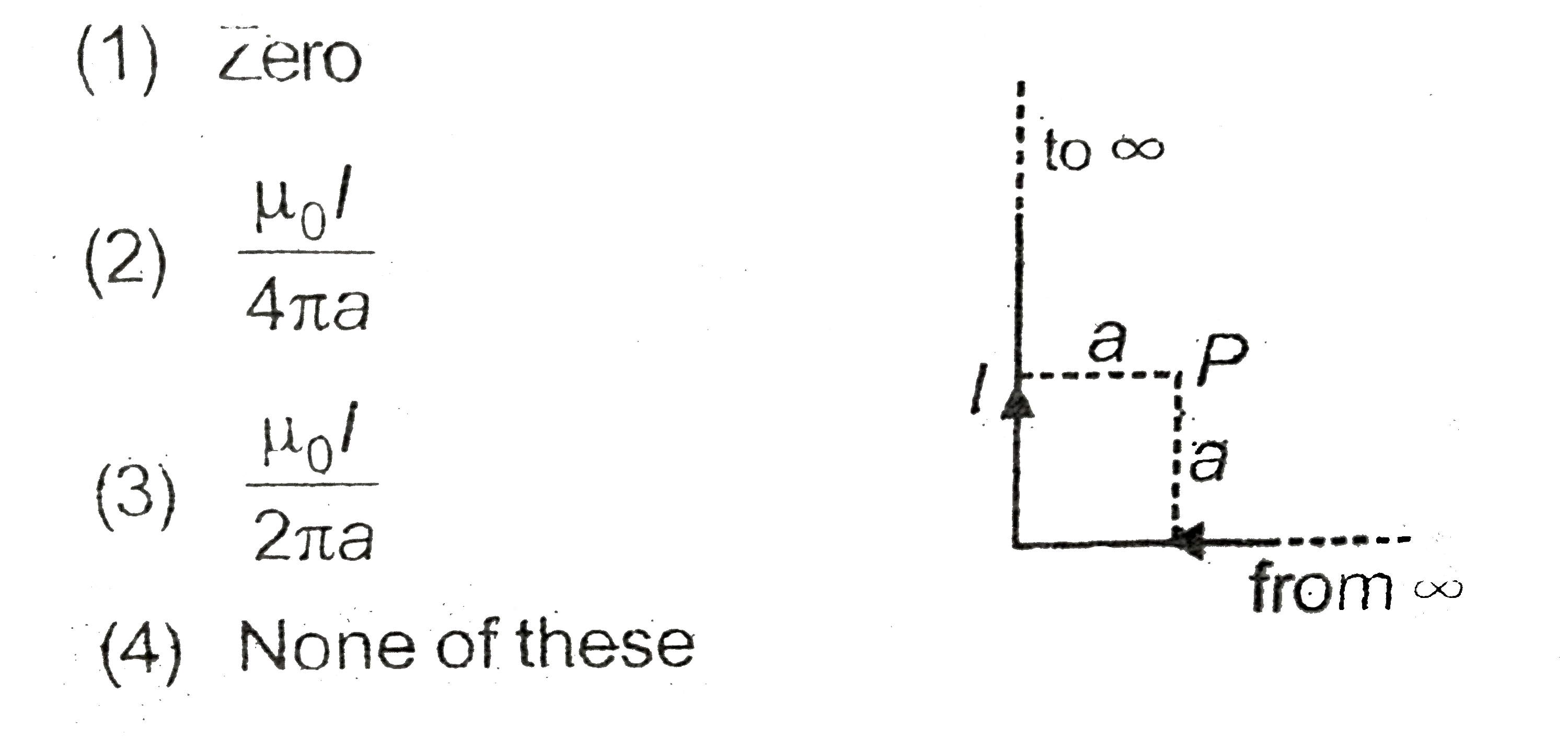
View Solution