In a series LCR circuit resonance of frequency is the half of the frequency of source.The nature of the circuit is
A. Inductive
B. Can not be defined
C. Capacitive
D. Resistive
To solve the problem regarding the nature of a series LCR circuit where the resonance frequency is half of the frequency of the source, we can follow these steps:
1. Understand Resonance in LCR Circuit:
In a series LCR circuit, resonance occurs when the inductive reactance (XL) is equal to the capacitive reactance (XC). This can be expressed mathematically as:
XL=XC
where XL=ωL and XC=1ωC.
2. Relate Resonant Frequency to Source Frequency:
The problem states that the resonance frequency is half of the frequency of the source. If we denote the source frequency as fs, then the resonant frequency fr can be expressed as:
fr=12fs
3. Convert Frequency to Angular Frequency:
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Therefore, the resonant angular frequency ωr can be expressed as:
ωr=2πfr=2π(12fs)=πfs
4. Substitute Resonant Frequency into Reactance Equations:
At resonance, we have:
XL=XC⟹ωrL=1ωrC
Substituting ωr=πfs:
πfsL=1πfsC
5. Analyze the Condition:
Rearranging gives:
(πfs)2LC=1
This indicates a relationship between the inductance L and capacitance C at the resonant frequency.
6. Determine the Nature of the Circuit:
Since the problem states that the resonant frequency is half of the source frequency, we can infer that at this condition, the capacitive reactance XC must be greater than the inductive reactance XL for the circuit to resonate at a lower frequency. This implies that the circuit behaves more like a capacitor than an inductor.
7. Conclusion:
Therefore, the nature of the circuit is capacitive.
Final Answer:
The nature of the circuit is capacitive.
In a series LCR circuit, resonance occurs when the inductive reactance (XL) is equal to the capacitive reactance (XC). This can be expressed mathematically as:
XL=XC
where XL=ωL and XC=1ωC.
The problem states that the resonance frequency is half of the frequency of the source. If we denote the source frequency as fs, then the resonant frequency fr can be expressed as:
fr=12fs
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Therefore, the resonant angular frequency ωr can be expressed as:
ωr=2πfr=2π(12fs)=πfs
At resonance, we have:
XL=XC⟹ωrL=1ωrC
Substituting ωr=πfs:
πfsL=1πfsC
Rearranging gives:
(πfs)2LC=1
This indicates a relationship between the inductance L and capacitance C at the resonant frequency.
Since the problem states that the resonant frequency is half of the source frequency, we can infer that at this condition, the capacitive reactance XC must be greater than the inductive reactance XL for the circuit to resonate at a lower frequency. This implies that the circuit behaves more like a capacitor than an inductor.
Therefore, the nature of the circuit is capacitive.
The nature of the circuit is capacitive.
Similar Questions
Knowledge Check
- Question 1 - Select One
Figure shows a series LCR circuit connected to a variable frequency 230 V source.
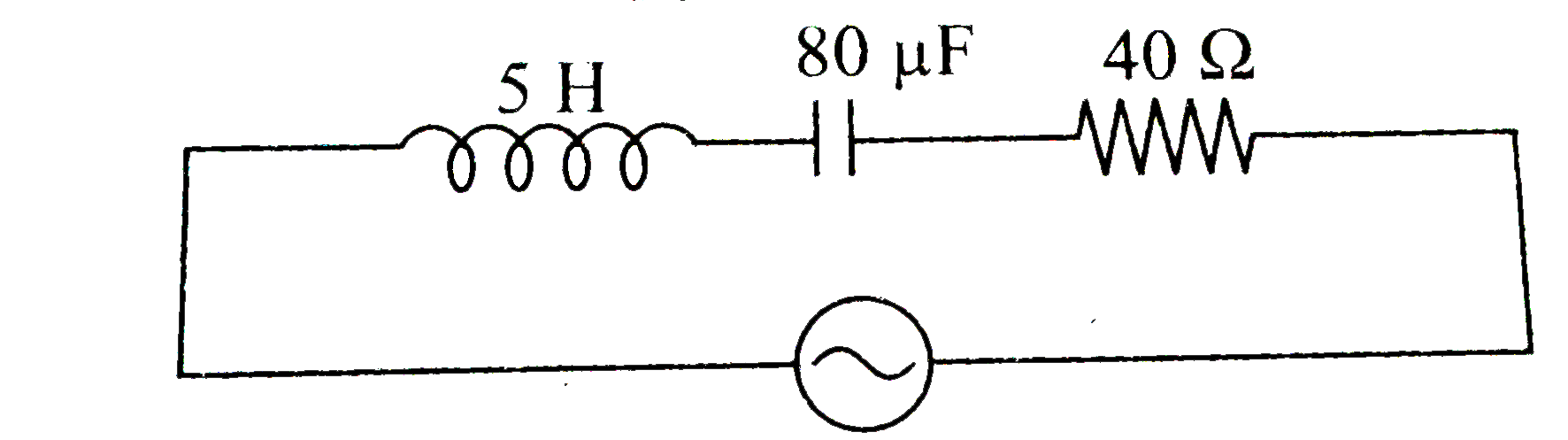
The source frequency which drives the circuit the circuit in resonance isA4 HzB5 HzC6 HzD8 Hz
In a series LCR circuit, at resonance, power factor is …….. .
View SolutionIn a series LCR circuit, the frequency of the source is more than resonance frequency. The current in the circuit leads the voltage in phases(True/false).
View SolutionIn a series LCR circuit, obtain an expression for the resonant frequency.
View SolutionIn a R, L, C circult, three elements is connected in series by an ac source. If frequency is less than resonating frequency then net impedance of the circuit will be
View SolutionAn alternating voltage of frequency f is applied across a series LCR circuit.Le fr be the resonance frequency for the circuit.Will the current in the circuit lag, lead or remain in phase with the applied voltage when (i)f>fr,(ii)f<fr ? Explain your answer in each case.
View SolutionFor the series LCR circuit shown in the figure, what is the resonance frequency and the amplitude of the current at the resonating frequency
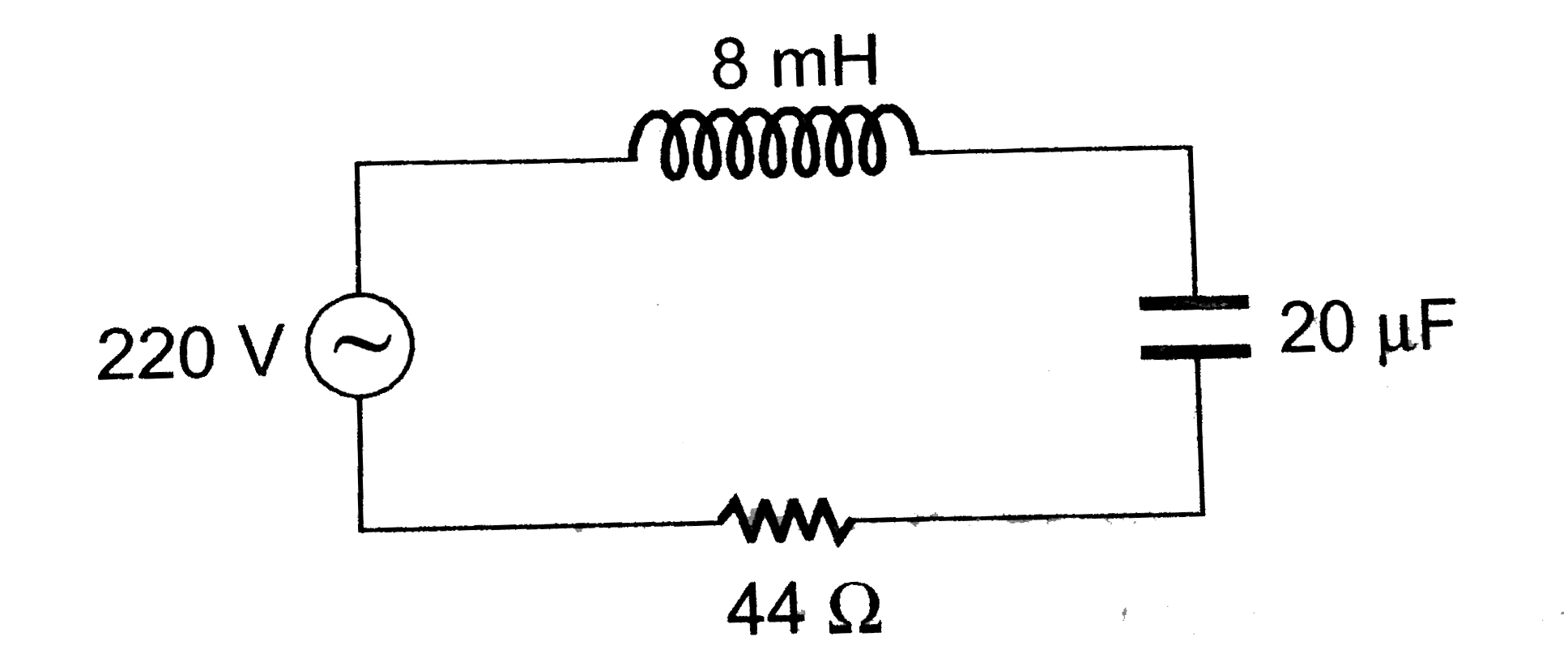 View Solution
View SolutionFor the series LCR circuit shown in the figure, what is the resonance frequency and the amplitude of the current at the resonating frequency
 View Solution
View Solution

