A fruit which is at 20 m above a lake suddenly detaches from the tree.A fish inside the lake , in the line of fall of fruit,is looking at fruit when fruit is at 12.8 m above the water surface.Speed of fruit as observed by fish (μwater=43andg=10ms2)
6 m/s
12 m/s
15 m/s
16 m/s
The correct Answer is:D
To solve the problem step by step, we will follow the physics principles involved in the motion of the fruit and the effect of the refractive index on the speed observed by the fish.
Step 1: Determine the displacement of the fruit
The fruit is initially at a height of 20 m and is at a height of 12.8 m when observed by the fish. The displacement s of the fruit is given by:
s=20m−12.8m=7.2m
Step 2: Use the kinematic equation to find the speed of the fruit
Since the fruit is dropped from rest, its initial velocity u=0 m/s. We can use the kinematic equation:
v2=u2+2as
where:
- v is the final velocity,
- u is the initial velocity (0 m/s),
- a is the acceleration due to gravity (given as g=10m/s2),
- s is the displacement (7.2 m).
Substituting the values:
v2=0+2⋅10⋅7.2
v2=144
v=√144=12m/s
Step 3: Calculate the speed of the fruit as observed by the fish
The speed of the fruit as observed by the fish in the water is affected by the refractive index of water. The relationship is given by:
Vf=μ⋅v
where:
- Vf is the speed of the fruit as observed by the fish,
- μ is the refractive index of water (given as 43),
- v is the speed of the fruit in air (12 m/s).
Substituting the values:
Vf=43⋅12
Vf=483=16m/s
Final Answer
The speed of the fruit as observed by the fish is 16m/s.
---
The fruit is initially at a height of 20 m and is at a height of 12.8 m when observed by the fish. The displacement s of the fruit is given by:
s=20m−12.8m=7.2m
Since the fruit is dropped from rest, its initial velocity u=0 m/s. We can use the kinematic equation:
v2=u2+2as
where:
- v is the final velocity,
- u is the initial velocity (0 m/s),
- a is the acceleration due to gravity (given as g=10m/s2),
- s is the displacement (7.2 m).
v2=0+2⋅10⋅7.2
v2=144
v=√144=12m/s
The speed of the fruit as observed by the fish in the water is affected by the refractive index of water. The relationship is given by:
Vf=μ⋅v
where:
- Vf is the speed of the fruit as observed by the fish,
- μ is the refractive index of water (given as 43),
- v is the speed of the fruit in air (12 m/s).
Vf=43⋅12
Vf=483=16m/s
The speed of the fruit as observed by the fish is 16m/s.
Similar Questions
A ball is dropped from a height of 20m above the surface of water in a lake. The refractive index of water is 4/3. A fish inside the lake , in the line fall of the ball, is looking at the ball. At an instant when the balll is 12.8m above the water surface, the fish sees the speed of ball as .
View SolutionA fish lies at the bottom of a 4m deep water lake. A bird flies 6m above the water surface and refractive index of water is 4/3 . Then the distance between
View Solution
Knowledge Check
- Question 1 - Select One
A fish which is at a depth of 12 cm in water (μ=43) is viewed by an observer on the bank of a lake. Its apparent depth as observed by the observer is :
A3 cmB9 cmC12 cmD16 cm - Question 2 - Select One
The rise in the water level in a capillary tube of radius 0.07 cm when dipped veryically in a beaker containing water of surface tension 0.07Nm−1 is (g = 10ms−2)
A2 cmB4 cmC1.5 cmD3 cm
A pile 4m high driven into the bottom of a lake is 1m above the <br>water . Determine the length of the shadow of the pile on the bottom of the lake if <br> the sun rays make an angle of 45∘ with the water surface . The refractive index<br> if water is 43.
View SolutionA pile 4m high driven into the bottom of a lake is 1m above the <br>water . Determine the length of the shadow of the pile on the bottom of the lake if <br> the sun rays make an angle of 45∘ with the water surface . The refractive index<br> if water is 43.
View SolutionIn a pond of water, a flame is held 2 m above the surface of the water. A fish is at depth of 4 m from the water surface. Refractive index of water is 43. The apparent height of the flame from the eyes of fish is -
View SolutionFind the energy possessed by an object of mass 10 kg when it is at a height of 6 m above the ground.Given, g=9.8m/s2
View SolutionA wooden block in the form of a uniform cylinder floats with one-third length above the water surface. A small chip of this block is held at rest at the bottom of a container containing water to a height of 1m and is then released. The time in which it will rise to the surface of water is =10m/s2)
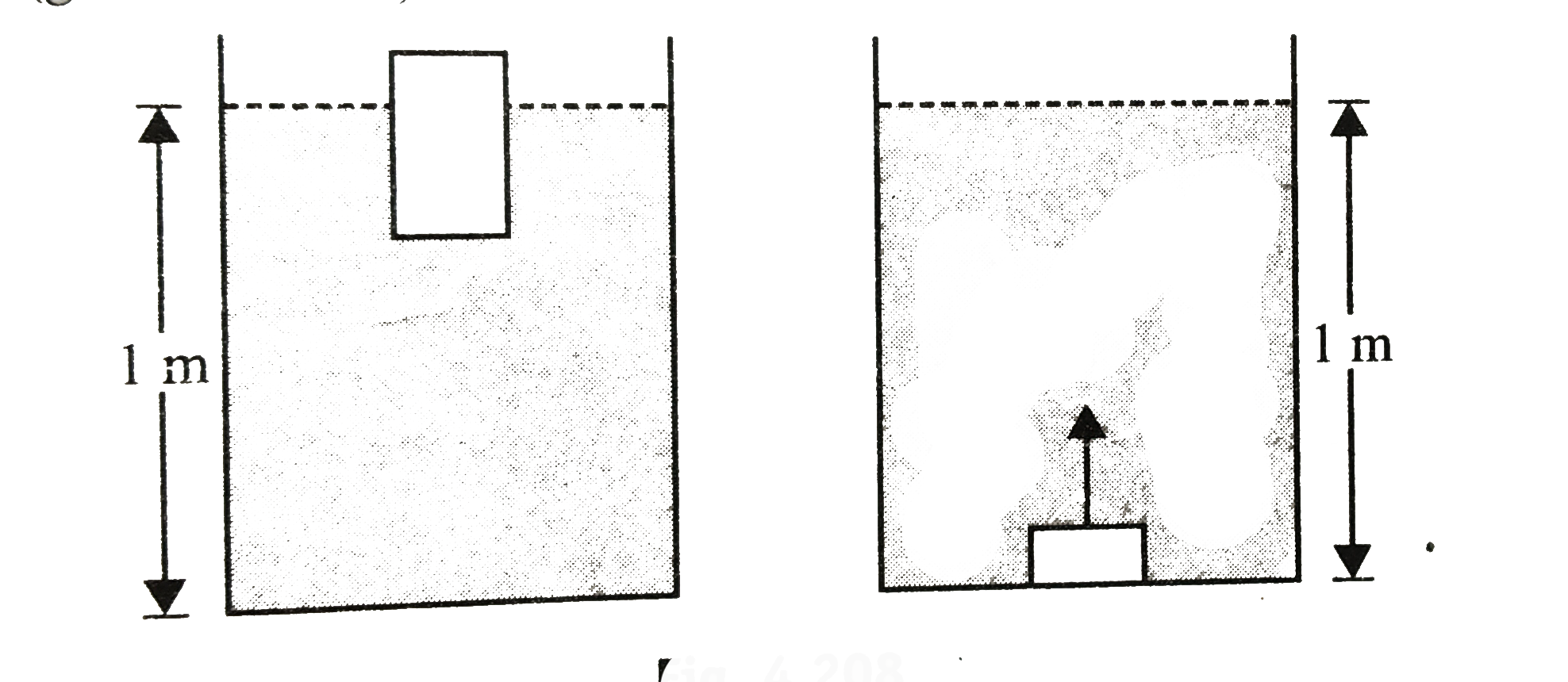 View Solution
View SolutionA small fish 0.4 m below the surface of a lake is viewed through a simple converging lens of focal length 3 m.the lens is kep at 0.2 m above the water surface such that the fish lies on the optical axis of the lens. Find the image of the fish seen by the observed. (μwater=43)
View Solution

