A flower can be cut into two equal or identical halves in any radial plane passing through the centre. This flower will be
Actinomorphic
Bilateral symmetric
Asymmetric
Zygomorphic
To solve the question, we need to understand the terms related to flower symmetry. Here’s a step-by-step breakdown:
1. Understanding Flower Symmetry: Flowers can be classified based on their symmetry into two main types: radial (actinomorphic) and bilateral (zygomorphic).
2. Radial Symmetry (Actinomorphic): A flower is said to be actinomorphic if it can be divided into two identical halves in any radial plane passing through the center. This means that the flower looks the same from multiple angles.
3. Bilateral Symmetry (Zygomorphic): A flower is zygomorphic if it can only be divided into two identical halves along one specific plane. This type of symmetry is more restrictive and is often seen in flowers like peas.
4. Analyzing the Question: The question states that the flower can be cut into two equal or identical halves in any radial plane passing through the center. This directly indicates that the flower exhibits radial symmetry.
5. Conclusion: Since the flower can be divided in any radial plane, it is classified as actinomorphic. Therefore, the answer to the question is that the flower is ectinomorphic.
Final Answer:
The flower will be ectinomorphic.
The flower will be ectinomorphic.
Topper's Solved these Questions
Similar Questions
A flower which can be divided into two exactly equal halves by any vertical planes passing through centre is
View SolutionAssertion :- Pea flowers are zygomorphic.
Reason :-Pea flowers can be cut in two equal halves by any radial plane passing through the centre.
View SolutionAssertion :- Canna flowers are asymmertric.
Reason :- Canna flowers cannot be cut into two equal halves by any vertical plane passing through the centre.
View SolutionBody can be divided into identical halves in many planes, passing through central axis in
View SolutionA flower which can be divided into two equal halves by only one plane is
View SolutionCan a satellite move in a stable orbit in a plane not passing through the earth's centre? Explain,
View SolutionIdentify the wrong statement
(a) A plant that bears male, female and bisexual flowers is polygamous
(b) Actinomorphic flower can be dissected into two equal halves from any plane
(c) Superior ovary is found in hypogynous flower
(d) Side of the flower towards the bract is called posterior side
View SolutionA bar magnet is cut into two equal halves by a plane parallel to the magnetic axis of the following physical quantities the one which remains unchanged is
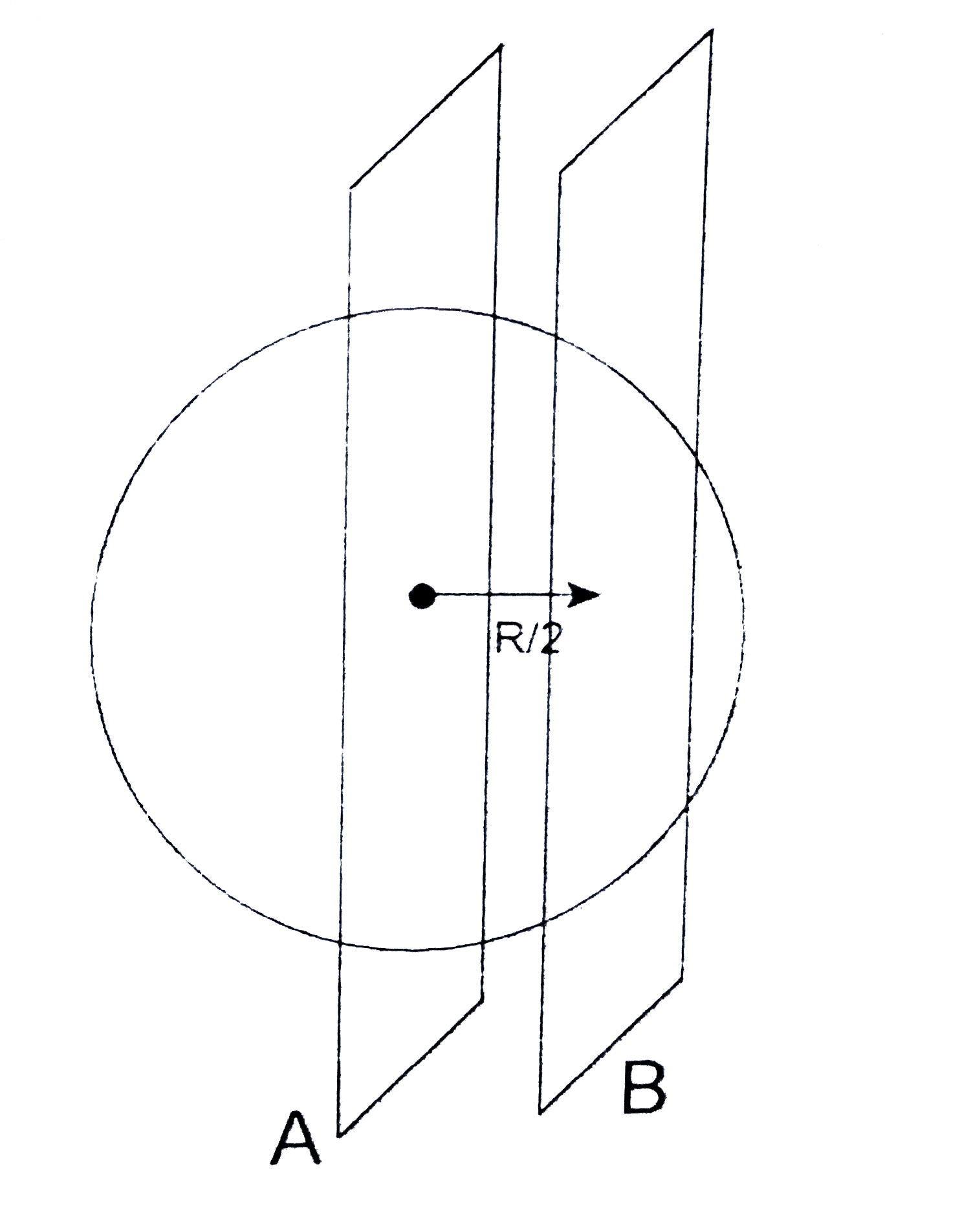
View SolutionFrom a sphere of electrical conductivity K two planes cut a piece such that first plane passes through the centre of sphere and second parallel to first one at distance R/2 from centre then resistance between A and B
View SolutionIf a circular concentric hole is made on a disc then about an axis passing through the centre of the disc and perpendicular to its plane
View Solution
