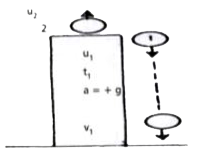
A ball is dropped from building and simultaneously another ball is projected upward with some velocity. Describe the change in relative velocities of the balls as a function of time.

Text Solution

Verified by Experts

For first ball $u = u_{1}, v = v_{1}, a = g, t = t$
Substituting these values in equation
$v = u + a t$ , we get
$v_{1} = u_{1} + >_{1} \dots(1)$
For second ball $u = u_{2}, v = v_{2}, a = - g, t = t_{2}$
Substituting these values in equation, $v = u + a t$ ,
We get, $v_{2} = u_{2} + >_{2} ...(2)$
$(1) - (2) \Rightarrow (v_{1} - v_{2}) = (u_{1} - u_{2}) + g (t_{1} + t_{2})$
$∴ (v_{1} - v_{2}) - (u_{1} - u_{2}) = g (t_{1} + t_{2})$
Given $∴ (v_{1} - v_{2}) - (0 - u_{2}) = g (t_{1} + t_{2})$
$∴$ The change in final relative velocity and initial relative velocity of two balls = Function of time.

Updated on:21/07/2023

A ball $A$ is dropped from a building of height $45 m$ . Simultaneously another ball $B$ is thrown up with a speed $40 m / s$ . Calculate the relative speed of the balls as a function of time.
A0
B $10 m s^{- 1}$
C $25 m s^{- 1}$
D $50 m s^{- 1}$
A ball is dropped from the top of a building 100 m high. At the same instant another ball is thrown upwards with a velocity of $40 m s^{- 1}$ form the bottom of the building. The two balls will meet after.
A5 s
B2.5 s
C2 s
D3 s
From the top of the tower of height $400 m$ , a ball is dropped by a man, simultaneously from the base of the tower, another ball is thrown up with a velocity $50 m / s$ . At what distance will they meet from the base of the tower ?
A100 m
B320 m
C80 m
D240 m