A car weight 1800 kg. The distance between its front and back axies is 1.8 m. Its centre of gravity is 1.05 m behind the front axle. Determine the force exerted by the level ground on each front wheel and each back wheel.

Text Solution

Verified by Experts

Here, m = 1800 kg
Distance between front and back axles = 1.8 m
Distance of center of gravity (c ) behind the front axle = 1.05 m
Let $R_{1} and R_{2}$ be the forces exerted by the level ground on each front wheel and each back wheel. As is clear from fig.
$R_{1} + R_{2} = m g = 1800 \times 9.8$

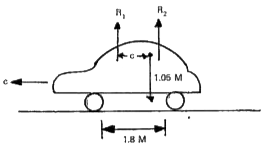
For rotational equilibrium about C,
$R_{1} \times 1.05 = R_{2} (1.8 - 1.05) = R_{2} \times 0.75 - (i)$
$\frac{R_{1}}{R_{2}} = \frac{0.75}{1.05} = \frac{5}{7}$
Putting in (i)
$\frac{5}{7} R_{2} + R_{2} = 1800 \times 9.8$
$R_{2} = \frac{7 \times 1800 \times 9.8}{12}$
= 10290 N
$R_{1} = \frac{5}{7} R_{2}$
$= \frac{5}{7} \times 10290$
= 7350 N.

Updated on:21/07/2023

A car weighs $1800 k g$ . The distance between its front and back axles is $1.8 m$ . Its centre of gravity is $1.05 m$ behind the front axle. Determine the force exerted by the level ground on each front wheel and each back wheel.
A4000 N on each front wheel, 5000 N on each back wheel
B5000 N on each front wheel, 4000 N an each back wheel
C4500 N on each wheel, 4500 N on each back wheel
D3000 N on each front wheel, 6000 N on each back wheel
A car weighs 1800 kg. The distance between its front and back axles is 1.8 m. Its centre of gravity is 1 m behind the front axle. The force exerted by the level ground on each front wheel and each back wheel is (Take $g : 10 m s^{- 1}$ )
A4000 N on each front wheel, 5000 N on each back wheel
B5000 N on each front wheel, 4000 N an each back wheel
C4500N on each front wheel, 4500 N on each back wheel
D3000 N on each front wheel, 6000 N on each back wheel
The centre of gravity of a car is at a height $h$ and the distance between its wheel is 2a. The car moves on a level curve of radius r with speed $v$ . Let $N_{1} and N_{2}$ be the normal reactions on the inner and outer wheels of the car. Then
A $N_{1} > N_{2}$
B $N_{2} > N_{1}$
C $N_{1} = N_{2}$
Dthe maximum value of v to avoid overturning is $\sqrt{\frac{a r g}{h}}$