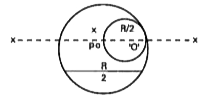
From a uniform disk of radis R, a circular hole of radius R/2 is cut out. The centre of the hole is a R/2 from the centre of the original disc. Locate the centre of gravity of the resulting flat body.

Text Solution

Verified by Experts

Suppose mass per unit area of the disc = M
$∴$ Mass of original disc

$M = π R^{2} \times m$
Mass of portion removed from the disc
$M . = π {(\frac{R}{2})}^{2} \times m = \frac{π R^{2}}{4} m = \frac{M}{4}$
In fig, mass M is connected at O and mass M. is concentrated at O., where OO. = $\frac{R}{2}$ .
After the circular disc of mass M. is removed, the remaining portion can be considered as a system of two masses M at O and - M. = $\frac{- M}{4}$ at O.. If x is the distance of centre of mass $(ρ)$ of the remaining part, then
$x = \frac{M \times O - M . \times \frac{R}{2}}{M - M .}$
$= \frac{\frac{- M}{4} \times \frac{R}{2}}{M - \frac{M}{4}} = \frac{- M R}{8} \times \frac{4}{3 M} = \frac{- R}{6}$
Negative sign shows that p is the left O.

Updated on:21/07/2023