A block and tackle system has 5 pulleys. In ideal situation,
(i) how is load L related to the effort E, and
(ii) how is the distance de moved by effort related to the distance d, moved by load?
To solve the question regarding the block and tackle system with 5 pulleys, we will break it down into two parts:
Part (i): Relationship between Load (L) and Effort (E)
1. Understanding Mechanical Advantage (MA):
- The mechanical advantage of a pulley system is defined as the ratio of the load (L) lifted to the effort (E) applied.
- In mathematical terms, this is expressed as:
MA=LE
2. Calculating Mechanical Advantage for 5 Pulleys:
- In an ideal block and tackle system, the mechanical advantage is equal to the number of supporting ropes. For a system with 5 pulleys, the mechanical advantage is:
MA=5
3. Relating Load and Effort:
- From the definition of mechanical advantage, we can express the relationship between load and effort as:
L=MA×E
- Substituting the value of MA:
L=5E
Part (ii): Relationship between Distance Moved by Effort (dE) and Distance Moved by Load (d)
1. Understanding Velocity Ratio (VR):
- The velocity ratio of a pulley system is defined as the ratio of the distance moved by the effort (dE) to the distance moved by the load (d).
- This can be expressed mathematically as:
VR=dEd
2. Calculating Velocity Ratio for 5 Pulleys:
- In an ideal system, the velocity ratio is equal to the number of pulleys. Therefore, for 5 pulleys:
VR=5
3. Relating Distances:
- From the definition of velocity ratio, we can express the relationship between the distances as:
dE=VR×d
- Substituting the value of VR:
dE=5d
Final Answers:
- (i) The relationship between load (L) and effort (E) is given by:
L=5E
- (ii) The relationship between the distance moved by effort (dE) and the distance moved by load (d) is given by:
dE=5d
- The mechanical advantage of a pulley system is defined as the ratio of the load (L) lifted to the effort (E) applied.
- In mathematical terms, this is expressed as:
MA=LE
- In an ideal block and tackle system, the mechanical advantage is equal to the number of supporting ropes. For a system with 5 pulleys, the mechanical advantage is:
MA=5
- From the definition of mechanical advantage, we can express the relationship between load and effort as:
L=MA×E
- Substituting the value of MA:
L=5E
- The velocity ratio of a pulley system is defined as the ratio of the distance moved by the effort (dE) to the distance moved by the load (d).
- This can be expressed mathematically as:
VR=dEd
- In an ideal system, the velocity ratio is equal to the number of pulleys. Therefore, for 5 pulleys:
VR=5
- From the definition of velocity ratio, we can express the relationship between the distances as:
dE=VR×d
- Substituting the value of VR:
dE=5d
- (i) The relationship between load (L) and effort (E) is given by:
L=5E
- (ii) The relationship between the distance moved by effort (dE) and the distance moved by load (d) is given by:
dE=5d
Topper's Solved these Questions
Similar Questions
Draw a diagram of a block and tackle system of pulleys having a velocity ratio of 5. in your diagram indicate clearly the points of application and the directions of the load L and effort E. also mark the tension T in each strand.
View SolutionA block and tackle system has 5 pulleys. If an effort of 1000 N is needed in downward direction to raise a load of 4500 N, calculate:
(a) the mechanical advantage,
(b) the velocity ratio, and
(c) the efficiency of the systemView Solution
Knowledge Check
- Question 1 - Select One
A block and tackle system of pulleys is used to lift a load of 360kgf by applying an effort of 200kgf such that load is lifted to a height of 2m and effort is applied through a distance of 8m. Find MA and VR of the system.
AMA = 3.6 and VR = 2BMA = 2 and VR = 8CMA = 1.8 and VR=4DMA = 4 and VR = 6 - Question 2 - Select One
In a block and tackle system of pulleys the load is lifted through a height of 2m while the effort is applied for 6m. Hence the velocity ratio of this system is
A2B3C6D12
A block and tackle has two pulleys in each block, with the tackle tied to the hook of the lower block and the effort being applied upwards.
(a) draw a neat diagram to show this arrangement and calculate its mechanical advantage.
(b) If the load moves up a distance x, by what distance will the fre end of the string move up ?View SolutionA block and tackle system has V.R. = 5.
Draw a neat labelled diagram of a system indicating the direction of its load and effort.View SolutionFigure shows a block and tackle system of pulleys used to lift a load.
(a) How many strands of tackle are supporting the load ?
(b) Draw arrows to represent tension T in each strand.
(c) What is the mechanical advantage of the system?
(d) When load is pulled up by a distance 1 m, how far does the effort end move ?
(e) How much effort is needed to lift a load of 100 N?
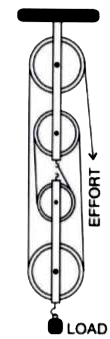 View Solution
View SolutionA block and tackle system of pulleys has velocity ratio 4.
Draw a neat labelled diagram of the system indicating clearly the points of application and direction of load and effort.View SolutionIn a block and tackle system consisting of 3 pulleys, a load of 75 kgf is raised with an effort of 25 kgf. Find : (i) the mechanical advantage, (ii) the velocity ratio, and (iii) the efficiency.
View SolutionIn the figure shown co-efficient of friction between the block B and C is 0.4. There is no friction between the block C and hte surface on which it is placed. The system of blocks is released from rest in the shown situation. Find the distance moved by the block C when block A descends through a distance 2m. Given masses of the blocks are mA=3kg,mB=5kgandmC=10kg.
 View Solution
View Solution

