The pressure P1 at the top of a dam and P2 at a depth h from the top inside water (density ρ) are related as :
P1>P2
P1=P2
P1−P2=hρg
P2−P1=hρg
The correct Answer is:A, B
To find the relationship between the pressure P1 at the top of a dam and the pressure P2 at a depth h from the top inside the water with density ρ, we can follow these steps:
Step 1: Understand the Concept of Pressure in Fluids
Pressure in a fluid increases with depth due to the weight of the fluid above. The pressure at a certain depth can be calculated using the formula:
P=P0+ρgh
where:
- P is the pressure at depth,
- P0 is the pressure at the surface (or at the top),
- ρ is the density of the fluid,
- g is the acceleration due to gravity,
- h is the depth from the surface.
Step 2: Identify the Pressures
In this case:
- P1 is the pressure at the top of the dam (which is P0).
- P2 is the pressure at depth h.
Step 3: Write the Pressure Relation
According to the formula, the pressure at depth h can be expressed as:
P2=P1+ρgh
Step 4: Rearranging the Equation
To find the relationship between P2 and P1, we can rearrange the equation:
P2−P1=ρgh
Step 5: Conclusion
Thus, the relationship between the pressures is:
P2−P1=ρgh
This indicates that the pressure at depth h is greater than the pressure at the top of the dam by an amount equal to the weight of the water column above that depth.
Final Answer
The correct relationship is:
P2−P1=ρgh
---
Pressure in a fluid increases with depth due to the weight of the fluid above. The pressure at a certain depth can be calculated using the formula:
P=P0+ρgh
where:
- P is the pressure at depth,
- P0 is the pressure at the surface (or at the top),
- ρ is the density of the fluid,
- g is the acceleration due to gravity,
- h is the depth from the surface.
In this case:
- P1 is the pressure at the top of the dam (which is P0).
- P2 is the pressure at depth h.
According to the formula, the pressure at depth h can be expressed as:
P2=P1+ρgh
To find the relationship between P2 and P1, we can rearrange the equation:
P2−P1=ρgh
Thus, the relationship between the pressures is:
P2−P1=ρgh
This indicates that the pressure at depth h is greater than the pressure at the top of the dam by an amount equal to the weight of the water column above that depth.
The correct relationship is:
P2−P1=ρgh
Topper's Solved these Questions
- BOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise-4(A)numericals10videos
PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE
- BOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise-4(A)Assumptions4videos
PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE
- BOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise-4(A)31videos
PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE
- BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (C) ( Multiple choice type :)17videos
MOTION IN ONE DIMENSION
- BOOK - ICSECHAPTER - PROPAGATION OF SOUND WAVES EXERCISE - EXERCISE -8(B) (Multiple choice type)3videos
PROPAGATION OF SOUND WAVES
Similar Questions
If P is the pressure and ρ is the density of a gas, then P and ρ are related as :
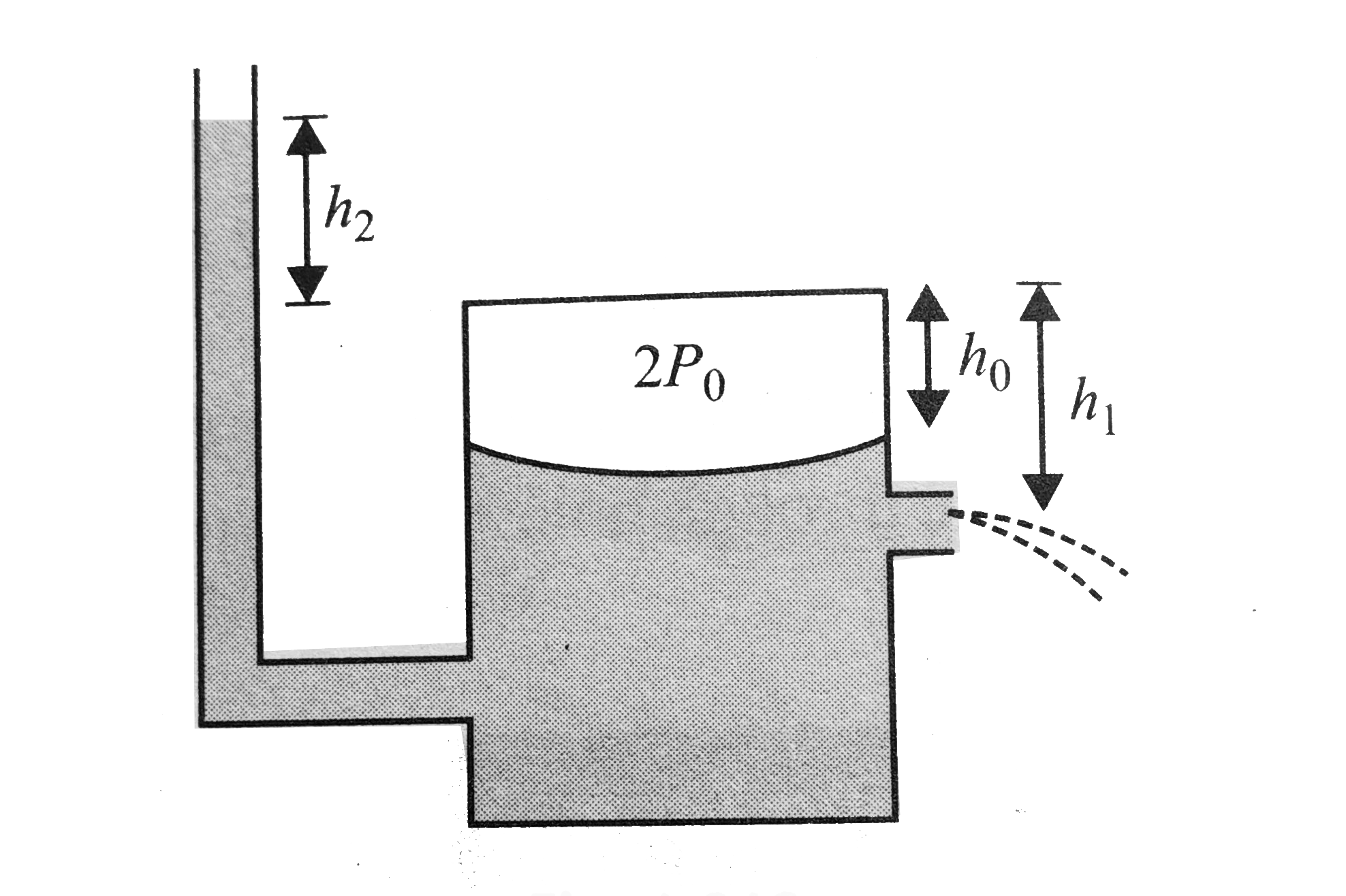
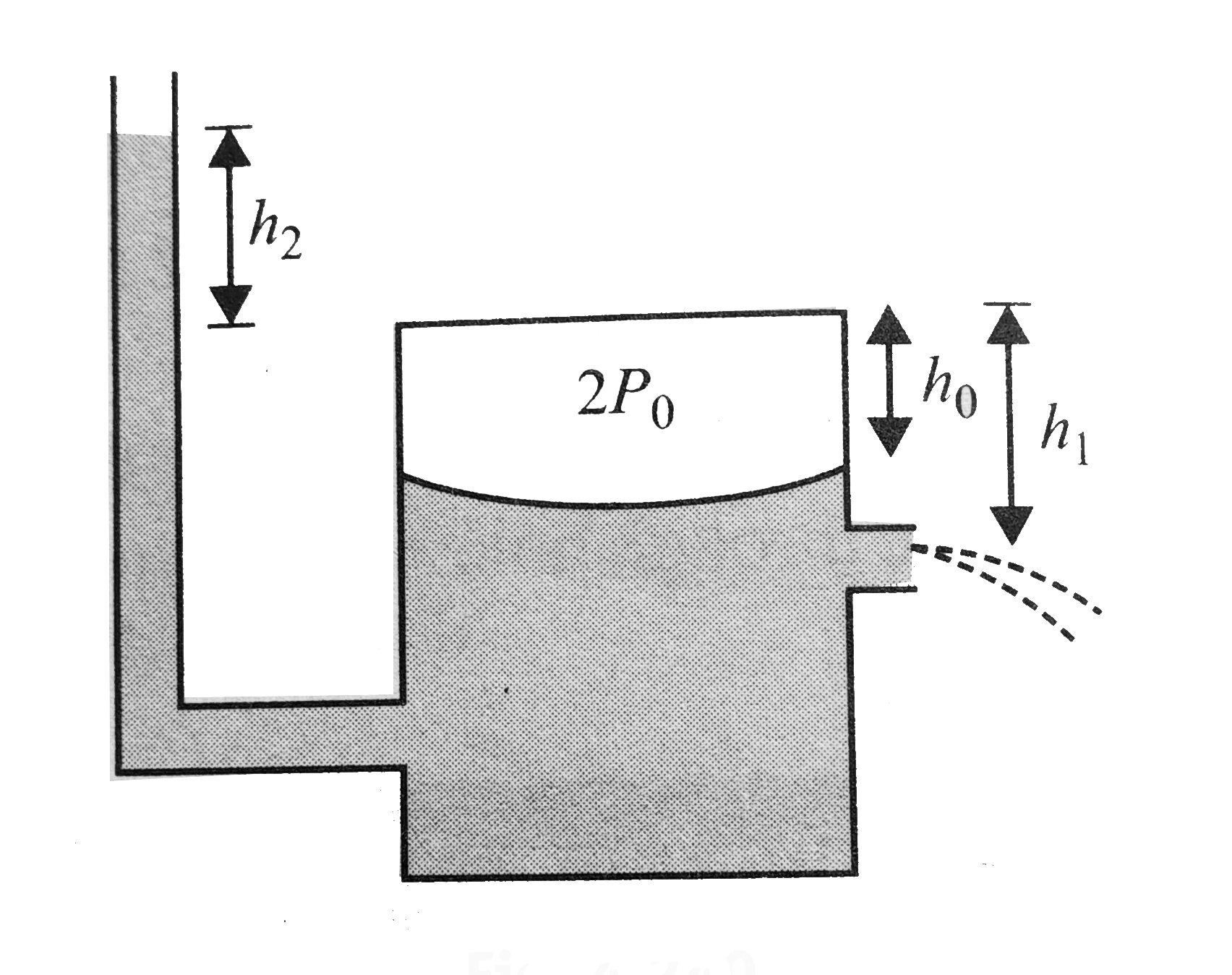
View SolutionFigure shows a large closed cylindrical tank containing water, Initially the air trapped above the water surface has a height h0and pressure 2p0 where p0is the atmospheric pressure. There is a hole in the wall of tank at a depth h1below the top from which water comes out. A long vertical tube is connected as shown. (a) Find the height h2 of the water in the long tube above the top initially. (b) Find the speed with which water comes out of hole.(c)Find the height of the water in the long tube above the top when the water stops coming out of the hole.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ - Question 2 - Select One
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth
Figure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.

Find the height h2 of the water in the long tube above top initially.View SolutionFigure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.
Find the height of the water in the long tube above the top when the water stops coming out of the hole.View SolutionFigure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.
Find the speed with which water comes out of the holeView SolutionTwo holes are made at depths h and 2h from the top. A liquid of density ρ and height h is filled over another liquid of density 2ρ. Speeds of liquid ejecting from two holes are say v1 and v2.
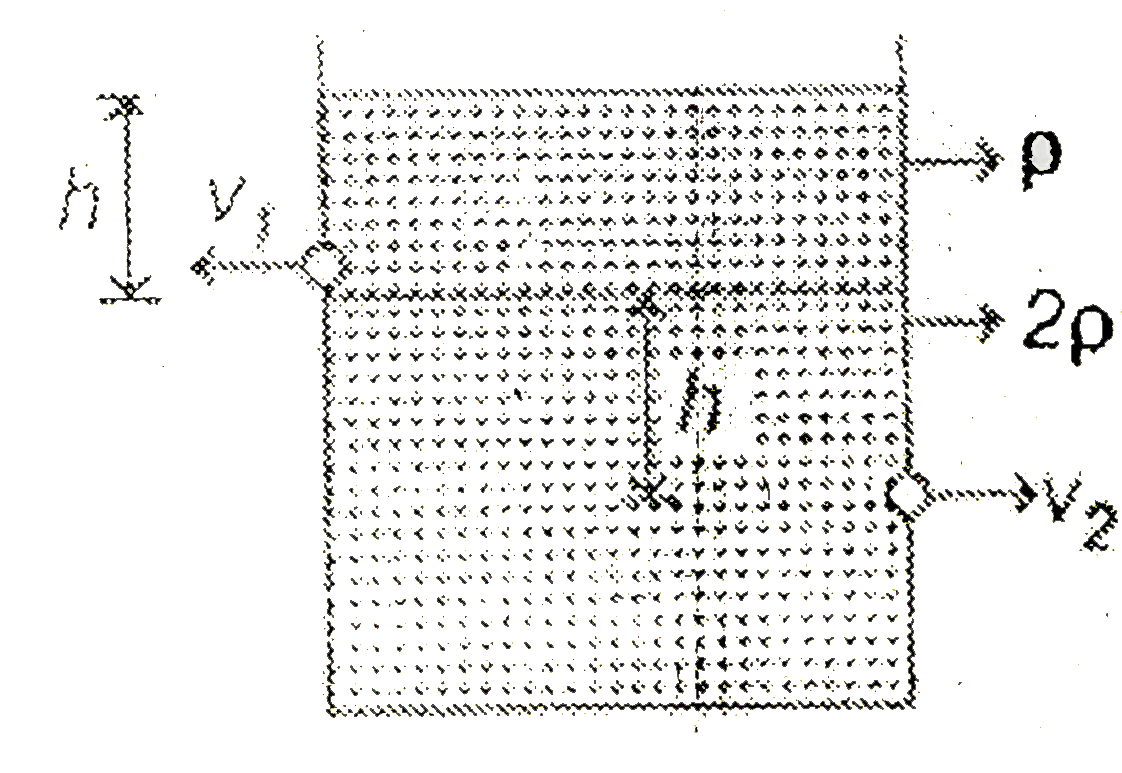
If density of lower liquid is increasedView SolutionTwo holes are made at depths h and 2h from the top. A liquid of density ρ and height h is filled over another liquid of density 2ρ. Speeds of liquid ejecting from two holes are say v1 and v2.
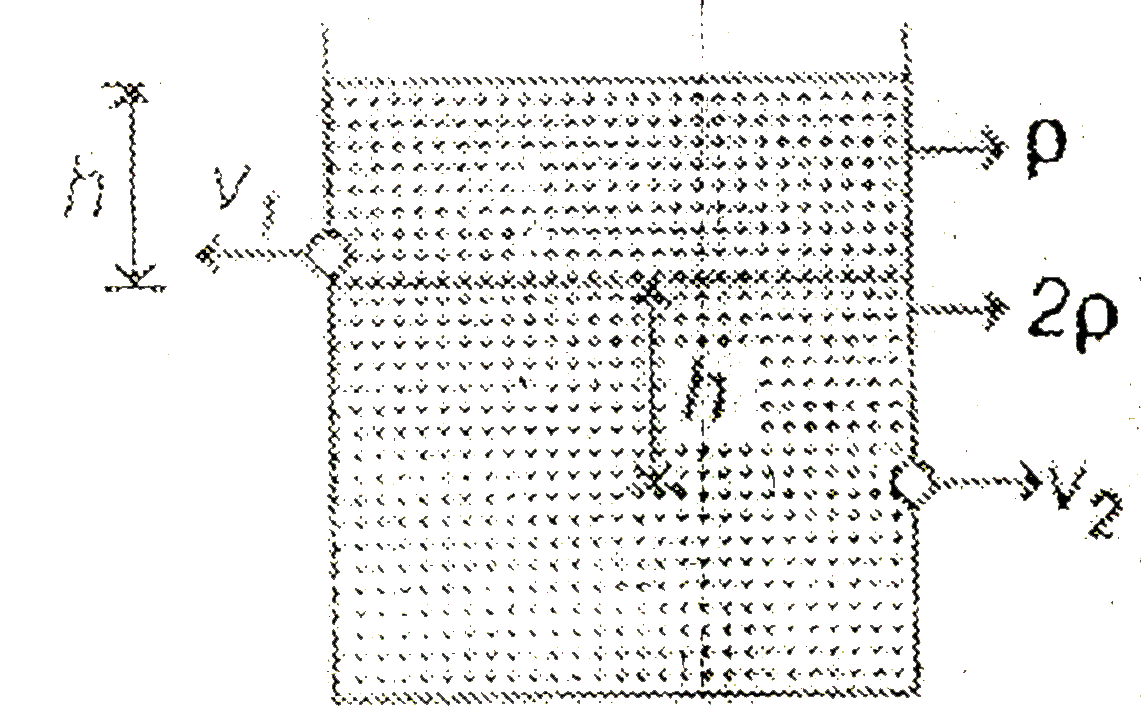
The ratio v1v2 will beView SolutionAn ideal fluid flows in the pipe as shown in the figure. The pressure in the fluid at the bottom P2 is the same as it is at the top P1. If the velocity of the top v1=2m/s. Then the ratio of areas A1.A2, is
 View Solution
View Solution

