Prove that-by joining the points '(2,1)', '(3,4),(-3,6)' we get a right triangle.Solution in Malayalam
Step by step video & image solution for Prove that-by joining the points '(2,1)', '(3,4),(-3,6)' we get a right triangle. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Suppose we draw circle with the bottom side of the. triangles in the picture as diameter. Find out whether the top comer of each triangle is inside the circle, on the circle. or outside the circle.
'(##VPU_TTT_MAT_X_P01_C02_E01_001_Q01##)'
View SolutionWhat are the coordinates of the fourth vertex of the parallelogram shown below.
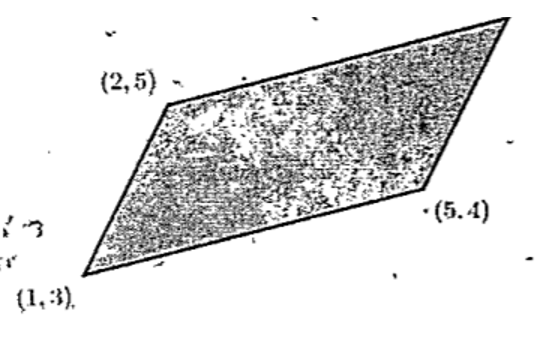
View SolutionThe vertices of a triangle ABC are (2,1),(5,2) and (3,4) respectively.The circumcentre is the point
View SolutionIf two vertices joining the hypotenuse of a right angled triangle are (0,0) and (3,4) ,then the length of the median through the vertex having right angle is-
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1.2).
View SolutionWe get a rhombus by joining the mid-points of the sides of (a) parallelogram (b) rhombus (c) rectangle (d) triangle
View SolutionIf points A(2,1);B(3,4);C(a,9) are vertices of right triangle at 'B' then find value of 'a'.
View SolutionWithout using Pythagoras theorem, show that the points A(2,1), B(5,4) and C(3,6) are the vertices of a right-angled triangle.
View Solutionबताओ बिंदु A(2,1),B(5,4) और C(3,6) एक किस त्रिभुज के शीर्ष है।
View SolutionProve that the triangle formed by joining the points
( - 4 , 9 , 6) , ( 0,7,10) , ( - 1 , 6 , 6 ) is an isosceles right angled triangle.
View Solutionसाबित करें कि बिंदु (3,4),(8,−6) तथा (13,9) एक समकोण त्रिभुज के शीर्ष हैं।
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2) .
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4)and (-1,2)
View Solution
