A steel wire of length 1 m, mass 0.1 kg and uniform cross-sectional area 10−6m2 is rigidly fixed at both ends. The temperature of wire is lowered by 20°C. If transverse waves are set up by plucking the string in the middle, calculate the frequency (In S.I. units) of the fundamental mode of vibration. Young’s modulus of steel =2×1011N/m2, coefficient of linear expansion of steel=1.21×10−6(degC)−1.
The mechanical strain =△ll=α△T=1.21×10−5×20=2.42×10−5
The tension in wire =T=Y△llA=2×1011×2.42×10−5×10−6=48.4N
∴ speed of wave in wire
V=√Tμ=√48.40.1=22m/s
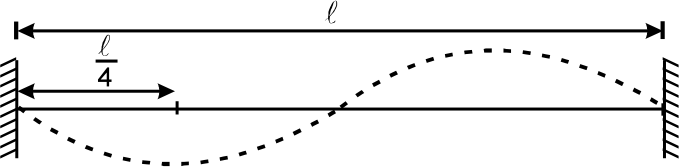
Since the wire is plucked at l4 from one end the wire shall oscillate in 1st overtone (for minimum number of loops)
λ=l=1m
Now V=fλ or f=Vλ=22Hz
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
View SolutionWhen p−n junction diode is forward biased, then
View SolutionA steel wire of length 1m , mass 0.1kg and uniform cross-sectional area 10^(-6)m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C . If transverse calculate the frequency of the fundamental mode of vibration. Given for steel Y = 2 xx 10^(11)N//m^(2) alpha = 1.21 xx 10^(-5) per ^(@)C
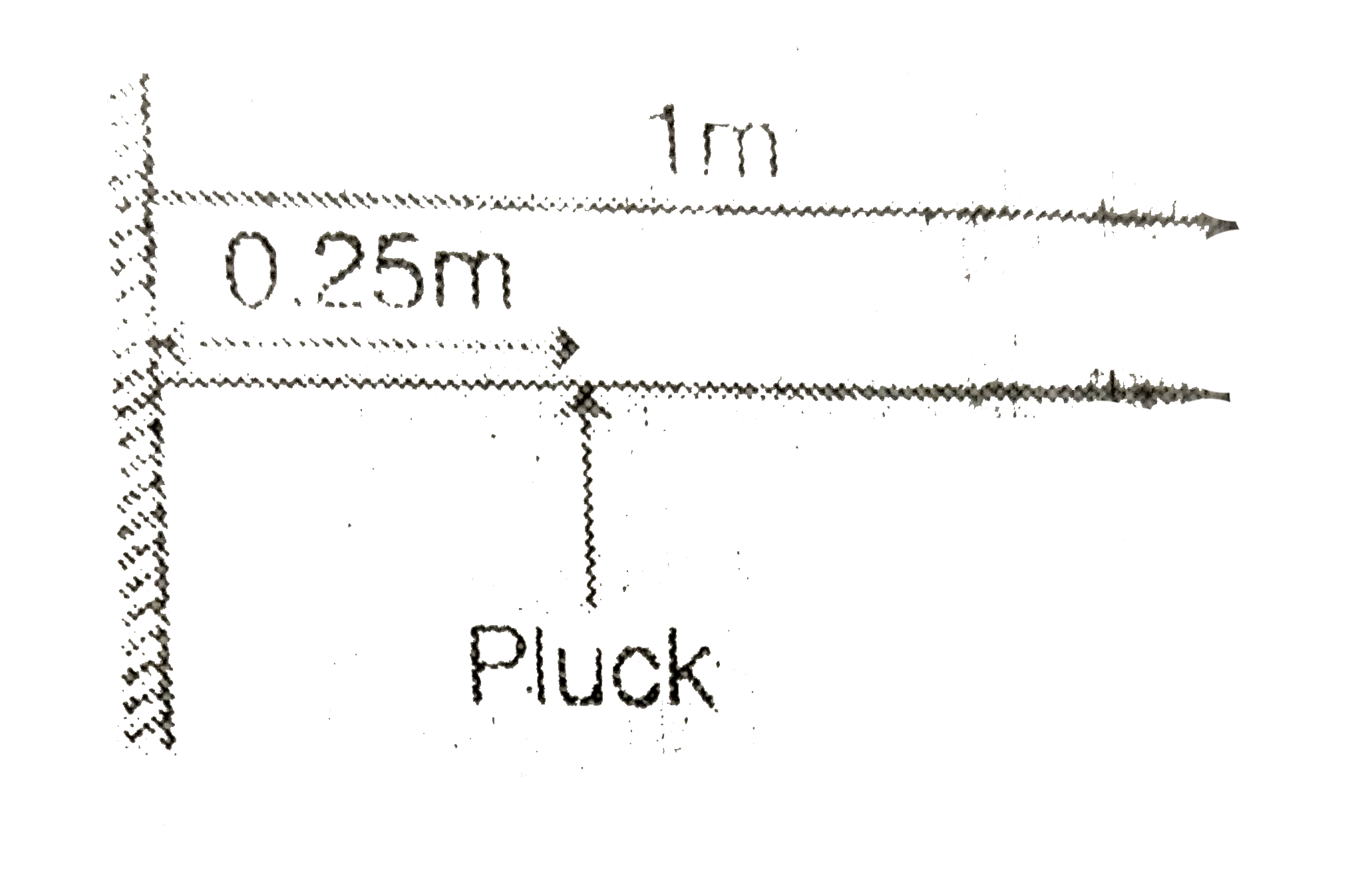
View SolutionA steel wire is rigidly fixed at both ends its length mass and cross sectional area are 1m, 0.1 kg and 106m2 respectively then the temperature of the wire is lowered by 20∘C if the transverse waves are setup by plucking the wire at 0.25m from one end and assuming that wire vibrates with minimum number of loops possible for such a case. Find the frequency of vibration (in Hz). [coefficient of linear expansion of steel =1.21×10−6/.∘C and young's modulus =2×1011/N/m2]
View SolutionA steel wire of length 20 cm and uniform cross-section 1mm^(2) is tied rigidly at both the ends. If the temperature of the wire is altered from 40^(@)C to 20^(@)C , the change in tension. [Given coefficient of linear expansion of steel is 1.1xx10^(5) .^(@)C^(-1) and Young's modulus for steel is 2.0xx10^(11) Nm^(-2) ]
View SolutionA steel wire of length 1 m and mass 0.1 kg and having a uniform cross-sectional area of 10^(-6) m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C . If the wire is vibrating in fundamental mode, find the frequency (in Hz). (Y_(steel)=2xx 10^(11) N//m^(2),alpha_(steel)=1.21 xx 10^(-5)//.^(@)C) .
View SolutionA steel wire is rigidly fixed at both ends. Its length mass and cross-sectionl area are 1m, 0.1kg and 10−8m2 respectively. Tension in the wire is produced by lowering the temperature by 20∘C. If the transerverse waves are some up by plucking the wire at 0.25m from one end and assuming that the wire viberates with minimum number of loops possble for such a case. The frequency of viberation (in Hz) is found to be. 1.11. Find the value of K. Given α=1.21×10−5.∘C−1.Y−2×1011N/m2
View Solutionस्टील का एक तार जिसकी लम्बाई 1 मीटर, द्रव्यमान 0.1 किग्रा तथा एकसमान परिच्छेद-क्षेत्रफल 10−6 मीटर2 है, दोनों सिरों पर दृढ़तापूर्वक बँधा है। तार का ताप 20∘C कम कर दिया जाता है। यदि तार को मध्य में कर्षित करके (by plucking) अनुप्रस्थ तरंगें उत्पन्न की जायें, तो कम्पन की मूल विधा की आवृत्ति की गणना कीजिए। स्टील का यंग-प्रत्यास्थता गुणांक =2×1011 न्यूटन/मीटर2, स्टील का रेखीय प्रसार गुणांक =1.21×10−5 प्रति∘C.
View SolutionA steel wire of length 5 m and area of cross-section 4mm2 is stretched by 2 mm by the application of a force. If young's modulus of steel is 2×1011N/m2, then the energy stored in the wire is
View SolutionA steel wire of length 0.20m and uniform cross section 10^(-6)m^(2) is tied rigidly at both ends. The temperature of the wire is changed from 40^(@) C to 20^(@)C . Calculate the thermal tension in the wire given alpha =1.1. xx 10^(-5)//.^(@)C and Y=2xx10^(11)Nm^(-2)
View SolutionCalculate the force required to increase the length of a steel wire of cross- sectional area 10^(-6)m^(2) by 0.5% given: Y_(("for steel"))=2xx10^(11)N-m^(2)
View SolutionA steel wire of length 1 m, mass 0.1 kg and uniform cross sectional area 10^(-6)m^(2) is rigidly fixed at two ends. The temperature of the wire is lowered by 20^(@) C . If transverse waves are now set up by lucking the string at the middle, calculate the frequency of the fundamental tone. Givne, for steel, co-efficient of linear expansion = 1.21 xx 10^(-5_(@))C^(1) and Young's modulus = 2 xx 10 ^(11) N* m^(-2) .
View SolutionA steel wire of length lm, mass 0.1 kg and uniform cross-sectional area 10−6m2 is rigidly fixed at both ends. The temperature of the wire is lowered by 200C. If the transverse waves are set up by plucking the string in the middle, calculate the frequency of the fundamental mode of vibration [Y=2×1011N/m2andα=1.21×10−5l0C]
View SolutionThe uniform transverse cut area of a steel wire of length 1m and mass 0.1 kg 10^(-6)m^(2) is. Is tightly tightened. Wire temperature 20^(@)C Let's reduce it. If the wire vibrates in its core, find the frequency (in hertz) Y_("steel")=2xx10^(11)N//m^(2), alpha_("steel")=1.21xx10^(-5)//""^(@)C Take)
View SolutionA steel wire of length lm, mass 0.1 kg and uniform cross-sectional area 10^(-6)m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(0)C . If the transverse waves are set up by plucking the string in the middle, calculate the frequency of the fundamental mode of vibration [Y=2xx10^(11)N//m^(2) and alpha=1.21xx10^(-5)l^(0)C]
View SolutionA steel wire of length im, mass 0.1kg and uniform cross sectional area 10−6m2 is rigidly fixed at both ends. The temperature of the wire is lowered by 20∘C. If the transverse waves are set up plucking the string in the middle, calculate the frequency of the fundamental mode of vibration. (Y=2×1011N/m2,a=1.21×10−5/∘C)
View Solution
