Number of integral values of x satisfying the inequality
(ex−πx)log2(x2−5x+62)(x−8)31(x−5)5(x+2)3(x8−x6+x4−x2+1)≥0
is/are
The correct Answer is:8
∵x8−x6+x4−x2+1>0∀×∈R
Domin x2−5x+6>0
(x−3)(x−2)>0
x∈(−∞,2)∪(3,∞)
Critical points xx−ex=0
log2(x2−5x+62)=0
x2−5x+62=1
x2+5x+4=0
(x−4)(x−1)=0
x=1,4
(ex−πx)log2'(x2−5x+62)(x−8)31(x−5)5(x+2)3(x8−x6+x4−x2+1)
⇒(πx−ex)log2'(x2−5x+62)(x−8)31(x−5)5(x+2)3
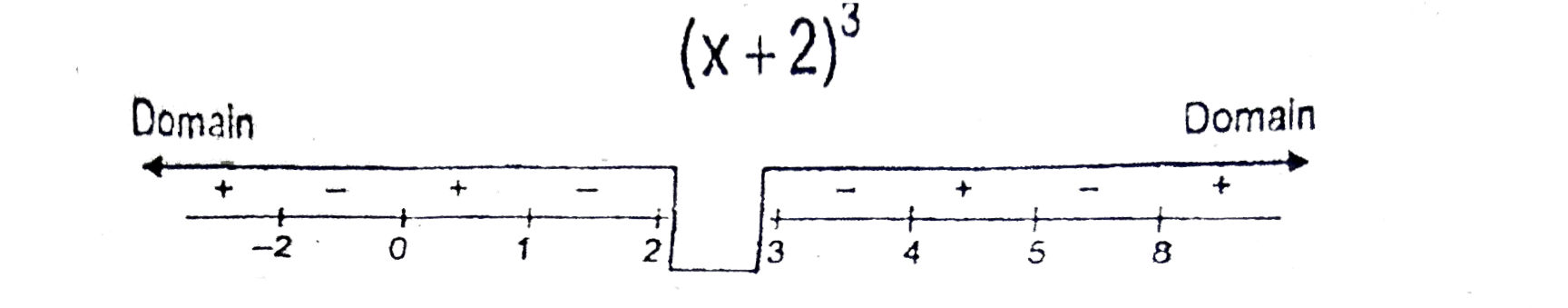
x∈(−2,]∪(1,2)∪(3,4]∪[5,8]
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - I MATHEMATICS SEC - 1
14 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - I MATHEMATICS SEC - 2
1 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART : 1MATHEMATICS
9 Videos
- TEST PAPERBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPEREXERCISE - MATHEMATICS
48 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - MATHEMATICS
132 Videos
Similar Questions
Find the number of integral values of x satisfying the inequality, x2−5x−6<0.
View SolutionFind the number of positive integral value of x satisfying the inequality (3x−5x)(x−2)(x2+5x+2)≥0
View SolutionFind the number of positive integral value of x satisfying the inequality (3x−5x)(x−2)(x2+5x+2)≥0
View SolutionFind the values of x satisfying the inequalities : log2(x2−24)>log2(5x)
View SolutionNumber of integral values of x which satisfy the inequality logx+1x(1+5x−x2)>0, is :
View SolutionThe number of negative integral values of x satisfying the inequality log(x+52)(x−52x−3)2<0 is :
View SolutionThe number of negative integral values of x satisfying the inequality log(x+52)(x−52x−3)2<0 is :
View SolutionNumber of integral value(s) of ' x ' satisfying the equation
|2x+1|+|5−2x|=6, is
View SolutionNumber of real values of x satisfying the equation logx2+6x+8(log2x2+2x+3(x2−2x))=0 is equal to
View SolutionThe value of x, satisfying the inequality log0.3(x2+8)>log0.39x, lies in
View Solution
