Question

Lines $5x+12y-10=0$ and $5x-12y-40=0$ touch a circle C1 of diameter 6. If the center of C1, lies in the first quadrant then the equation of the circle C2, which is concentric with C1, and cuts intercept of length 8 on these lines

Answer

5

Explanation

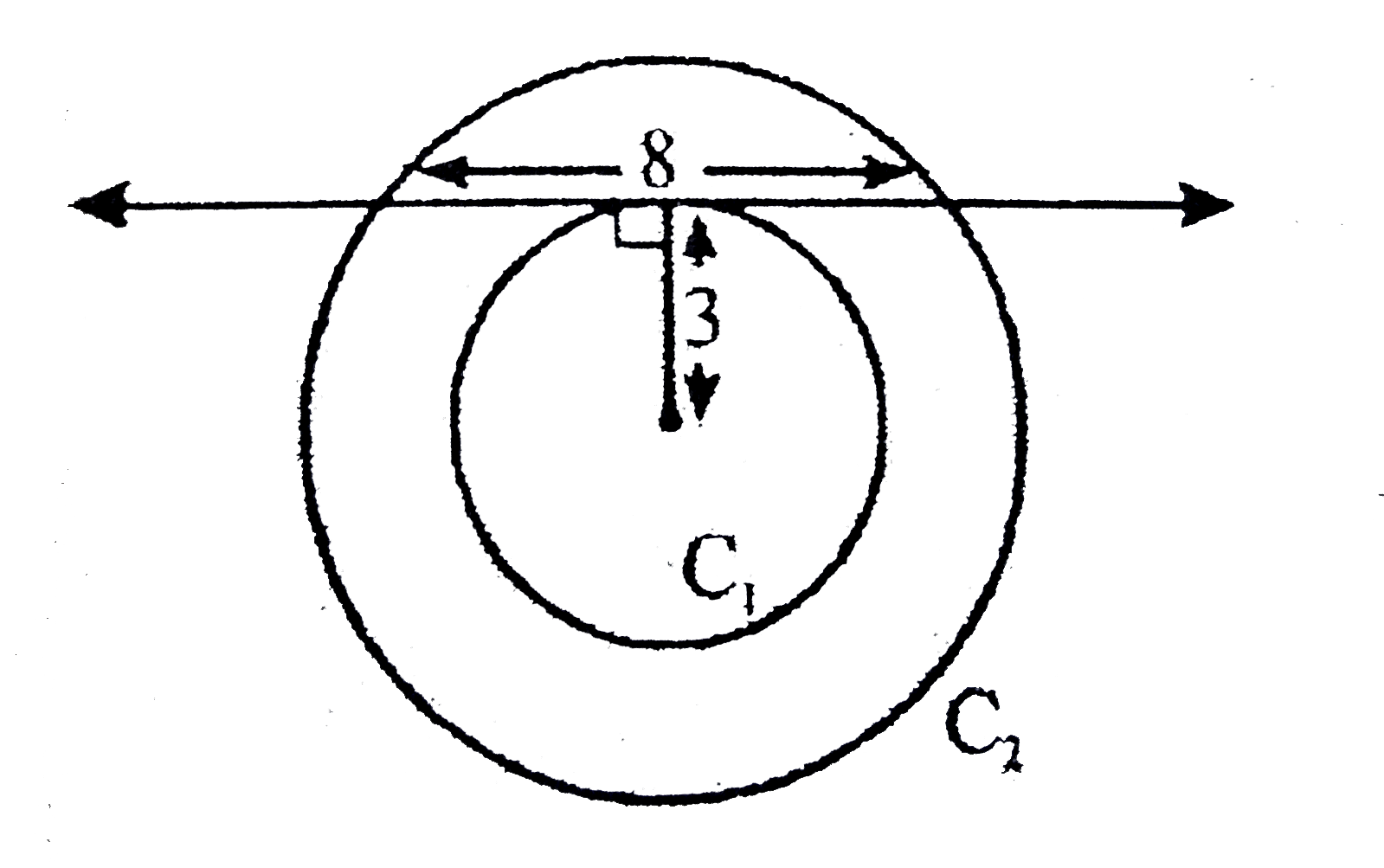
Radius of `C_(1) = 3`. If a tangent to `C_(1)` cuts an intercept of legth `8` on `C_(2)`
Radius of `C_(2)` on the point of contact `=` perpendicular draw from centre of `C_(2)` on its chord.
`rArr` Chord is bisected at the point of contact of `C_(1)`
`:.` Radius of `C_(2) sqrt(4^(2) + 3^(2)) = 5`