An iron block of mass 2kg, fall form a height 10m. After colliding with the ground it loses 25% enegry to surroundings. Then find the temperature rise of block. (Take sp. Heat of iron 470J/kg∘C)
To solve the problem step by step, we will follow these calculations:
Step 1: Calculate the Potential Energy (PE) of the Iron Block
The potential energy (PE) of the block can be calculated using the formula:
PE=mgh
Where:
- m=2kg (mass of the iron block)
- g=10m/s2 (acceleration due to gravity)
- h=10m (height from which the block falls)
Substituting the values:
PE=2kg×10m/s2×10m=200J
Step 2: Calculate the Energy Lost to Surroundings
The block loses 25% of its energy to the surroundings upon colliding with the ground. Therefore, the energy lost is:
Energy lost=0.25×PE=14×200J=50J
Step 3: Calculate the Energy Gained by the Block
According to the conservation of energy, the energy lost to the surroundings is equal to the energy gained by the block in the form of heat. Thus:
Energy gained by the block=50J
Step 4: Relate the Energy Gained to Temperature Rise
The energy gained by the block can also be expressed in terms of its specific heat capacity and temperature change:
Q=msΔT
Where:
- Q=50J (energy gained)
- m=2kg (mass of the block)
- s = 470 \, \text{J/(kg} \cdot \degree C) (specific heat of iron)
- ΔT = change in temperature (which we need to find)
Substituting the known values into the equation:
50 \, \text{J} = 2 \, \text{kg} \times 470 \, \text{J/(kg} \cdot \degree C) \times \Delta T
Step 5: Solve for Temperature Change (ΔT)
Rearranging the equation to solve for ΔT:
\Delta T = \frac{50 \, \text{J}}{2 \, \text{kg} \times 470 \, \text{J/(kg} \cdot \degree C)}
ΔT=50940
\Delta T \approx 0.053 \, \degree C
Final Answer
The temperature rise of the iron block is approximately:
\Delta T \approx 0.05 \, \degree C
---
The potential energy (PE) of the block can be calculated using the formula:
PE=mgh
Where:
- m=2kg (mass of the iron block)
- g=10m/s2 (acceleration due to gravity)
- h=10m (height from which the block falls)
PE=2kg×10m/s2×10m=200J
The block loses 25% of its energy to the surroundings upon colliding with the ground. Therefore, the energy lost is:
Energy lost=0.25×PE=14×200J=50J
According to the conservation of energy, the energy lost to the surroundings is equal to the energy gained by the block in the form of heat. Thus:
Energy gained by the block=50J
The energy gained by the block can also be expressed in terms of its specific heat capacity and temperature change:
Q=msΔT
Where:
- Q=50J (energy gained)
- m=2kg (mass of the block)
- s = 470 \, \text{J/(kg} \cdot \degree C) (specific heat of iron)
- ΔT = change in temperature (which we need to find)
50 \, \text{J} = 2 \, \text{kg} \times 470 \, \text{J/(kg} \cdot \degree C) \times \Delta T
Rearranging the equation to solve for ΔT:
\Delta T = \frac{50 \, \text{J}}{2 \, \text{kg} \times 470 \, \text{J/(kg} \cdot \degree C)}
ΔT=50940
\Delta T \approx 0.053 \, \degree C
The temperature rise of the iron block is approximately:
\Delta T \approx 0.05 \, \degree C
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Solved miscellaneous problems1videos
CALORIMETRY AND THERMAL EXPANSION
- BOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Problem26videos
CALORIMETRY AND THERMAL EXPANSION
- BOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Advanced Level Problems13videos
CALORIMETRY AND THERMAL EXPANSION
- BOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise19videos
CALORIMETRY
- BOOK - RESONANCE ENGLISHCHAPTER - CAPACITOREXERCISE - Exercise45videos
CAPACITOR
Similar Questions
A body of mass 0.2 kg falls from a height of 10 m to a height of 6 m above the ground. Find the loss in potential energy taking place in the body.
[g=10ms−2]View SolutionA body of mass m=2kg falls from a height h=10m from the ground level .
What is the magnitude of total change in momentum of the body before it strikes the ground?
View SolutionTwo identical masses of 5 kg each fall on a wheel from a height of 10 m . The wheel disturbs a mass of 2 kg water, the rise in temperature of water will be :-
View SolutionA steel ball of mass 0.1 kg falls freely from a height of 10 m and bounces to a height of 5.4 m from the ground. If the dissipated energy in this process is absorbed by the ball, the rise in its temperature is (specific heat of steel =460K/kg∘/C,g=10m/s2)
View SolutionA hot iron ball of mass 0.2 kg is added into 0.5 kg of water at 10∘C. The resulting temperature is 30∘C. Calculate the temperature of hot ball. Specific heat capacity of iron = 336 J kg−1K−1 and specific heat capacity of water = 4.2×103 J kg−1K−1.
View SolutionA body of mass 10 kg is kept at a height of 5 m. It is allowed to fall and reach the ground.
What is the kinetic energy possessed by the body just before hitting the ground? (Take g = 10m/s2).View SolutionBlock B of mass 2kg rests on block A of mass 10kg. All surfaces are rought with the value of coefficient of friction as shown in the figure. Find the minimum force F that should be applied on block A to cause relative motion between A and B. (g=10m/s2)
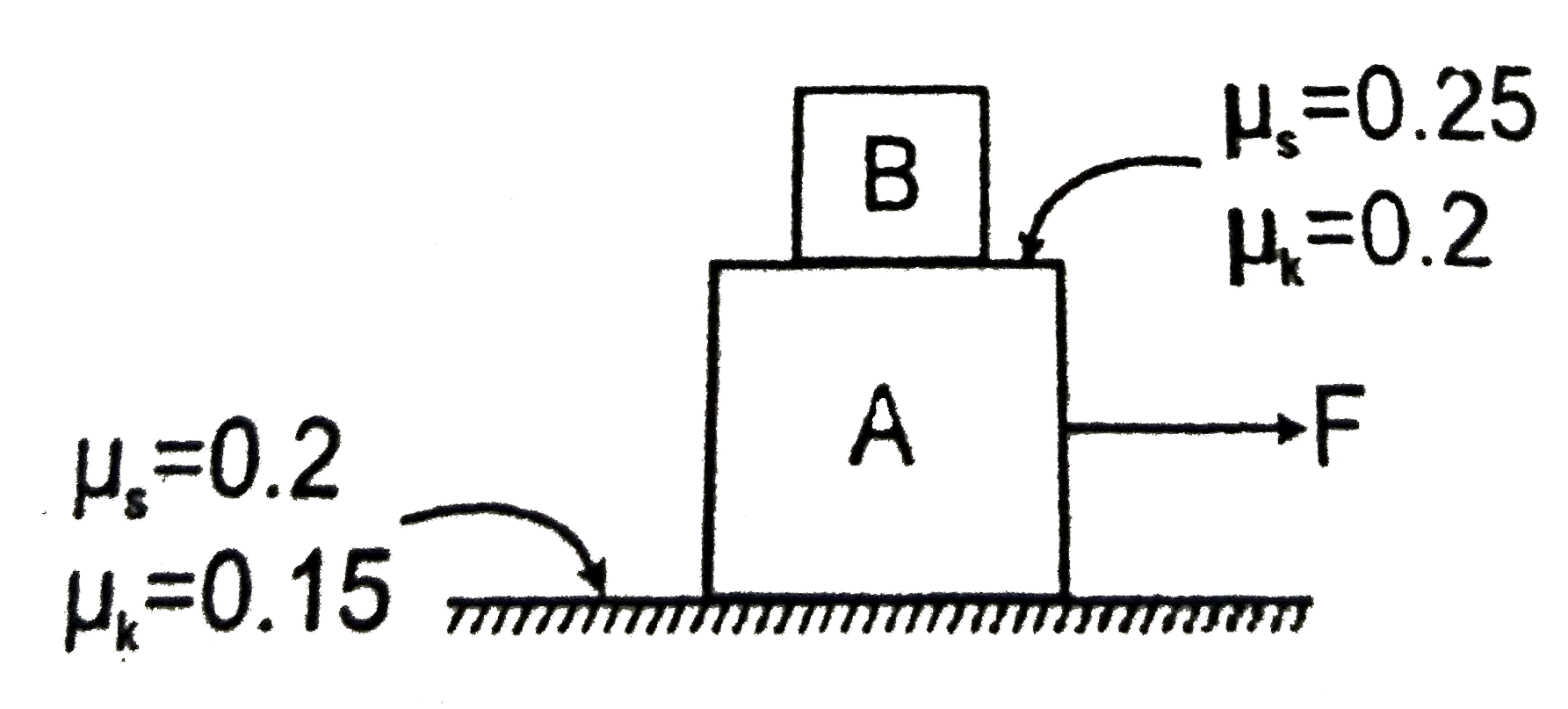 View Solution
View Solution
Block B of mass 2 kg rests on block A of mass 10 kg. All surfaces are rough with the value of coefficient of friction as shown in the figure. Find the minimum force F that should ber appplied on blocks A to cause relative motion between A and B.(g=10ms2)View Solution
Block B of mass 2 kg rests on block A of mass 10 kg. All surfaces are rough with the value of coefficient of friction as shown in the figure. Find the minimum force F that should ber appplied on blocks A to cause relative motion between A and B.(g=10ms2)View SolutionA container of negligible heat capacity contains 1kg of water. It is connected by a steel rod of length 10m and area of cross-section 10cm2 to a large steam chamber which is maintained at 100∘C. If initial temperature of water is 0∘C, find the time after which it beomes 50∘C. (Neglect heat capacity of steel rod and assume no loss of heat to surroundings) (use table 3.1, take specific heat of water =4180J/kg.∘C)
View Solution

