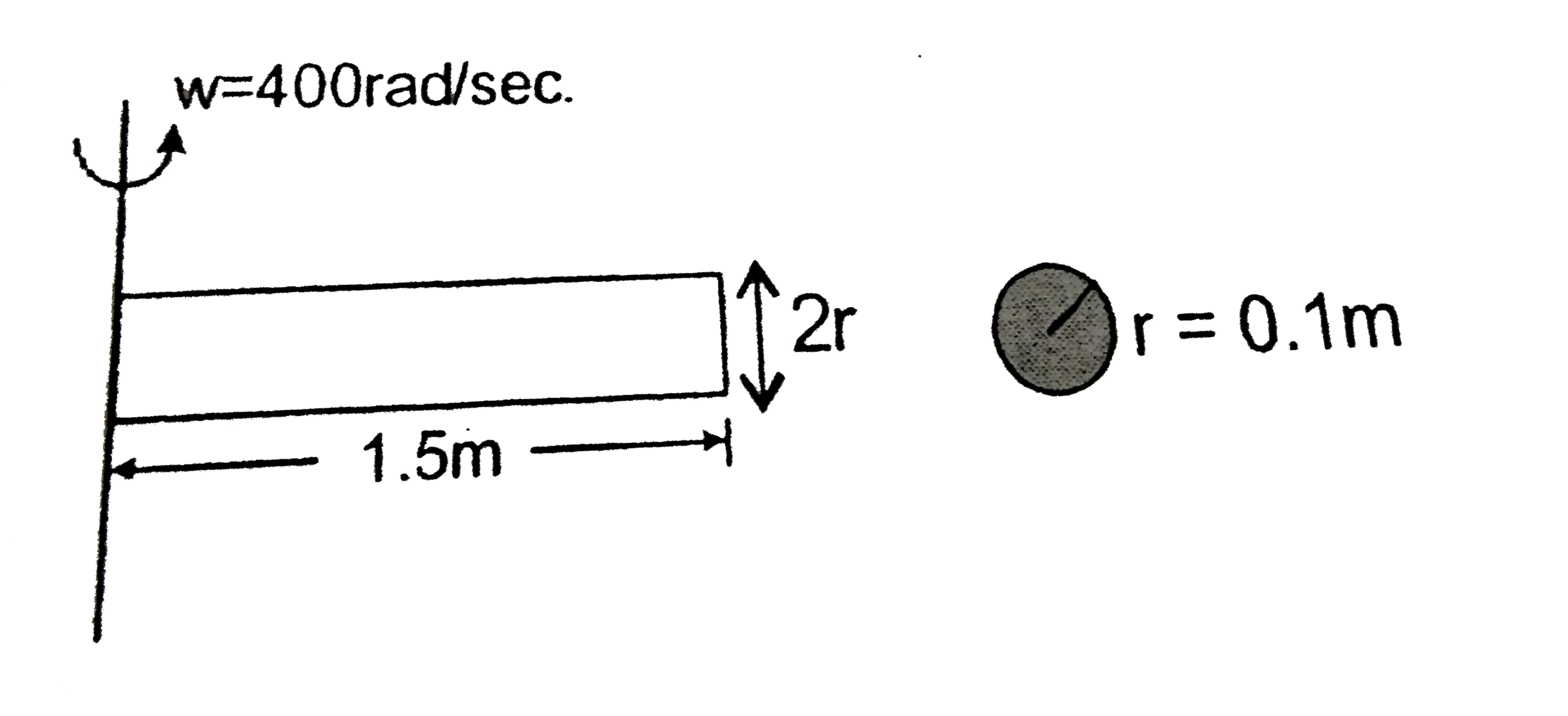
A rod of 1.5m length and uniform density 104kg/m3 is rotating at an angular velocity 400rad/sec about its one end in a horizontal plane. Find out elongation in rod.
Given y=2×1011N/m2
mass of shaded portion
m'−ml(l−x)[ where m= total mass ρAl]
T=m'ω2[l−x2+x]⇒T=ml(l−x)ω2(l+x2)T=mω22l(l2−x2)
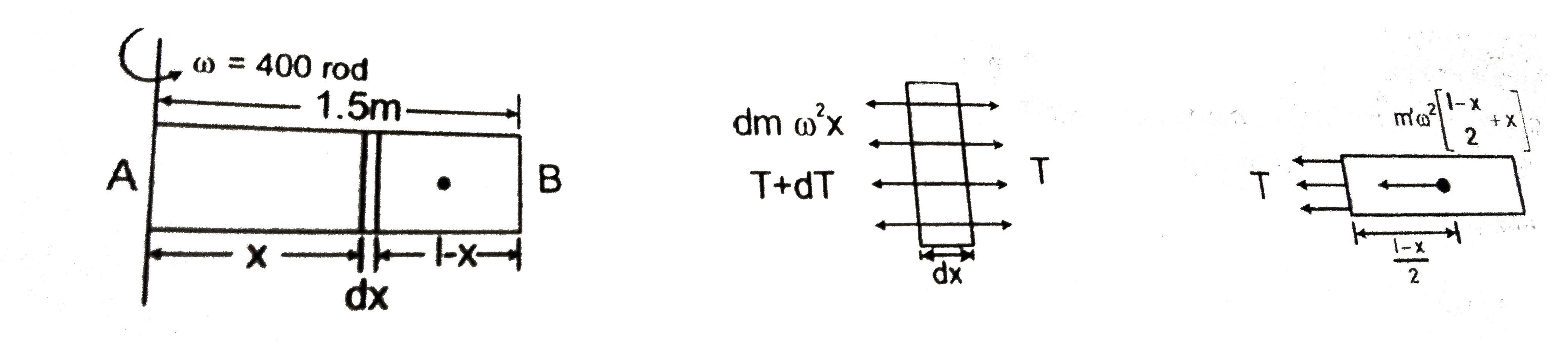
this tension will be maximum at A(mω2l2) and minum at 'B' (zero), elongation in element of width dx'=TdxAy
Total elongation
δ=∫TdxAy=l∫0mω2(l2−x2)2lAydx
δ=mω22lAy[l2x−x33]l0=mω2×2l32lAy×3=mω2l23Ay=ρAlω2l23Ay
δ=ρω2l33y=104×(400)×(1.5)33×2×1011=9×10−3m=9mm
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 90 Illustration2videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 91 illustration2videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - Now answer the following :20videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise53videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions

A rod of mass 2 kg ad length 2 m is rotating about its one end O with an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.View SolutionA copper wire of negligible mass, 1m length and cross-sectional area 10−6m2 is kept on a smooth horizontal table with one end fixed. A ball of mass 1kg is attached to the other end. The wire and the ball are rotating with an angular velocity of 20rad/s. If the elongation in the wire is 10−3m.
a. Find the Young's modulus of the wire (in terms of ×1011N/m2).
b. If for the same wire as stated above, the angular velocity is increased to 100rad/s and the wire breaks down, find the breaking stress (in terms of ×1010N/m2).View SolutionA spherical planet has uniform density π2×104kg/m3. Find out the minimum period for a satellite in a circular orbit around it in seconds (Use G=203×10−11N−m2kg2).
View SolutionA unifrom rod AB of length 4m and mass 12kg is thrown such that just after the projection the centre of mass of the rod moves vertically upwards with a velocity 10m/s and at the same time its is rotating with an angular velocity π2rad/sec about a horizontal axis passing through its mid point. Just after the rod is thrown it is horizontal and is as shown in the figure. Find the acceleration (in m/sec2) of the point A in m/s2 when the centre of mass is at the highest point. (Take =10m/s2 and π2=10)
 View Solution
View SolutionA rod of length l and total charge 'q' which is uniformly distributed is rotating with angular velocity ω about an axis passing through the centre of rod and perpendicular to rod. Find the magnitude of magnetic dipole moment (in Amp. m2) of rod. If q=4C,ω=3rad/s and l=2m
View SolutionA rod of length L is pivoted at one end and is rotated with as uniform angular velocity in a horizontal plane. Let T1andT2 be the tensions at the points L/4 and 3L/4 away from the pivoted ends.
View SolutionThe thin rod shown below has mases M and length L. A force F acts at one end as shown and the rod is free to rotate about the other end in horizontal plane. Initial angular acceleration of the rod is :
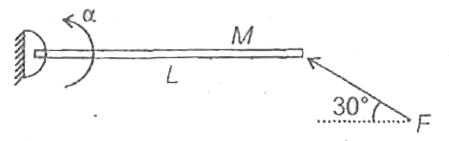 View Solution
View Solution
The kinetic energy of a uniform rod of mass m rotating with constant angular velocit ω about one of its end which is fixed as shown in figure is mω2l2N find NView SolutionA thin rod of mass m has an angular velocity ω0 while rotating on a smooth surface. Determine its new angular velocity (in rads//s) just after its end strike and hooks onto the peg and the rod starts to rotate about P without rebounding. Solve the problem using the parameters given. m=2kg,ω0=rad/s,l=1.5m
 View Solution
View SolutionA thin rod of mass m has an angular velocity ω0 while rotating on a smooth surface. Determine its new angular velocity (in rads//s) just after its end strike and hooks onto the peg and the rod starts to rotate about P without rebounding. Solve the problem using the parameters given. m=2kg,ω0=rad/s,l=1.5m
 View Solution
View Solution

