Result of calculations for four different design of a fusion reactor using D−D reaction are given below. which of these is most promising based on Lawson criterion ?
deuteron density =2.0×1012cm−3, cofinement time =5.0×10−3s
deuteron density =8.0×1014cm−3, cofinement time =9.0×10−1s
deuteron density =4.0×1023cm−3, confinement time =1.0×10−11s
deuteron density =1.0×1024cm−3, confinement time =4.0×10−12s
The correct Answer is:B
To determine which fusion reactor design is the most promising based on the Lawson criterion, we need to analyze the given values for deuteron density (N) and confinement time (T₀) for each design. The Lawson criterion states that for a fusion reactor to be considered promising, the product of the deuteron density and confinement time must exceed 5×1014cm−3.
1. Understand the Lawson Criterion: The criterion is given by the equation:
N×T0>5×1014cm−3
where N is the deuteron density in cm−3 and T0 is the confinement time in seconds.
2. Identify the Values for Each Design: We need to look at the values of N and T0 for each of the four designs. Let's assume we have the following values:
- Design A: NA=x1cm−3,T0A=y1s
- Design B: NB=8×1014cm−3,T0B=9×10−1s
- Design C: NC=x2cm−3,T0C=y2s
- Design D: ND=x3cm−3,T0D=y3s
3. Calculate the Product for Each Design:
- For Design A:
PA=NA×T0A
- For Design B:
PB=NB×T0B=(8×1014)×(9×10−1)=7.2×1014cm−3
- For Design C:
PC=NC×T0C
- For Design D:
PD=ND×T0D
4. Compare Each Product with the Lawson Criterion:
- Check if PA>5×1014
- Check if PB>5×1014 (we already calculated this and found it is true)
- Check if PC>5×1014
- Check if PD>5×1014
5. Determine the Most Promising Design: The design with the highest product P that also satisfies the Lawson criterion will be the most promising.
Conclusion:
Based on the calculations, since PB=7.2×1014cm−3 is greater than 5×1014cm−3, Design B is the most promising option.
N×T0>5×1014cm−3
where N is the deuteron density in cm−3 and T0 is the confinement time in seconds.
- Design A: NA=x1cm−3,T0A=y1s
- Design B: NB=8×1014cm−3,T0B=9×10−1s
- Design C: NC=x2cm−3,T0C=y2s
- Design D: ND=x3cm−3,T0D=y3s
- For Design A:
PA=NA×T0A
- For Design B:
PB=NB×T0B=(8×1014)×(9×10−1)=7.2×1014cm−3
- For Design C:
PC=NC×T0C
- For Design D:
PD=ND×T0D
- Check if PA>5×1014
- Check if PB>5×1014 (we already calculated this and found it is true)
- Check if PC>5×1014
- Check if PD>5×1014
Based on the calculations, since PB=7.2×1014cm−3 is greater than 5×1014cm−3, Design B is the most promising option.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.29
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.30
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.27
20 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Give the products of the reactions given below.
b.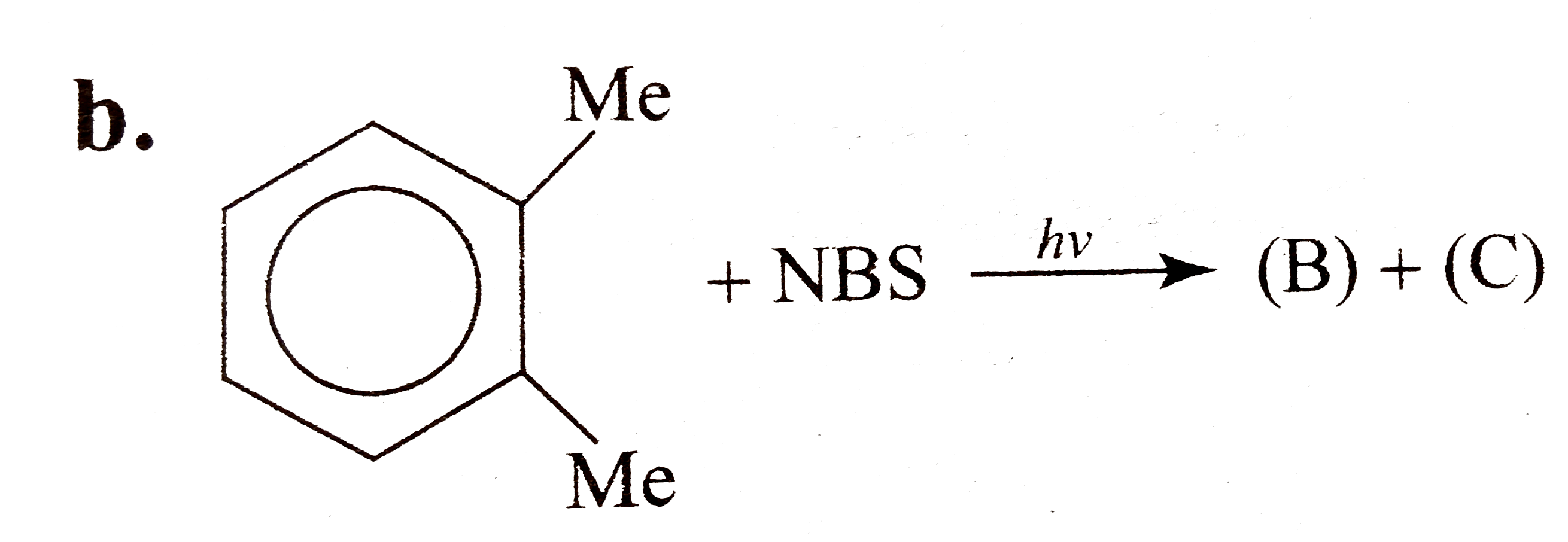
c.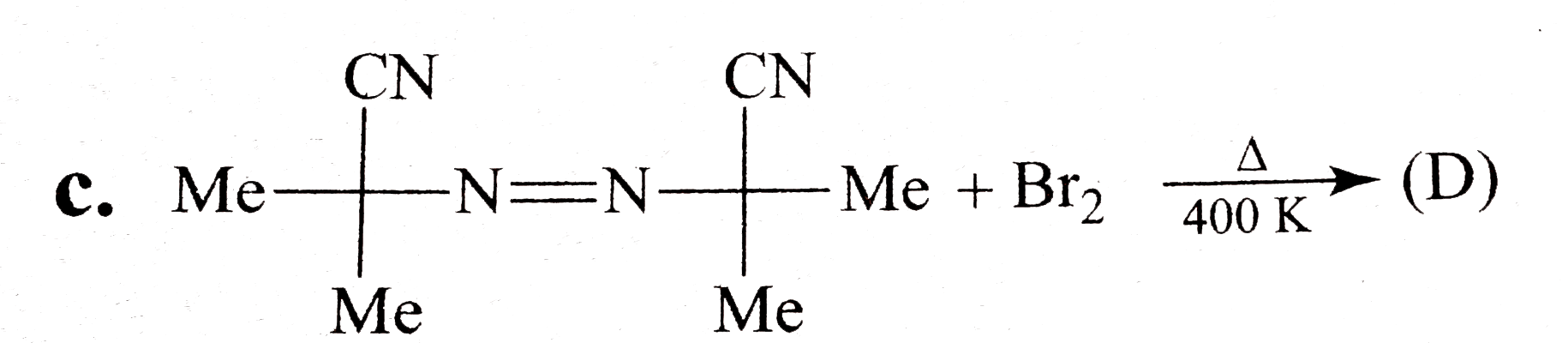
d.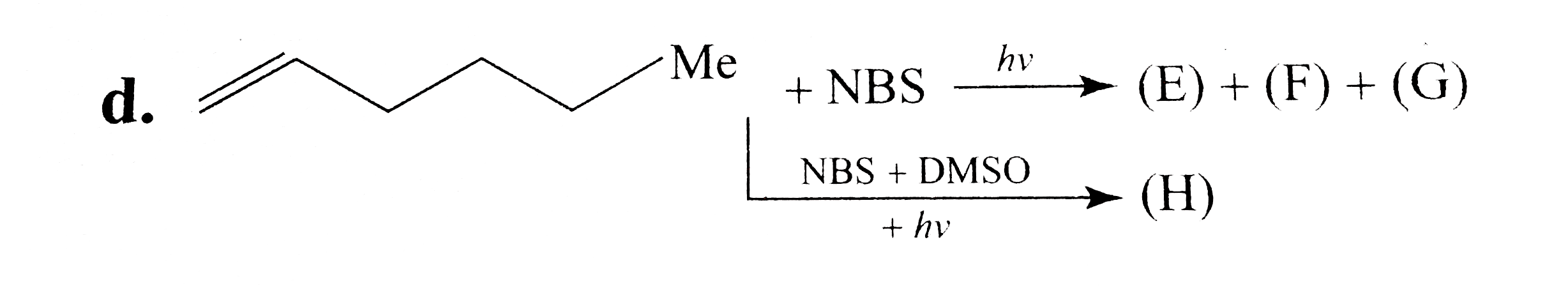
e.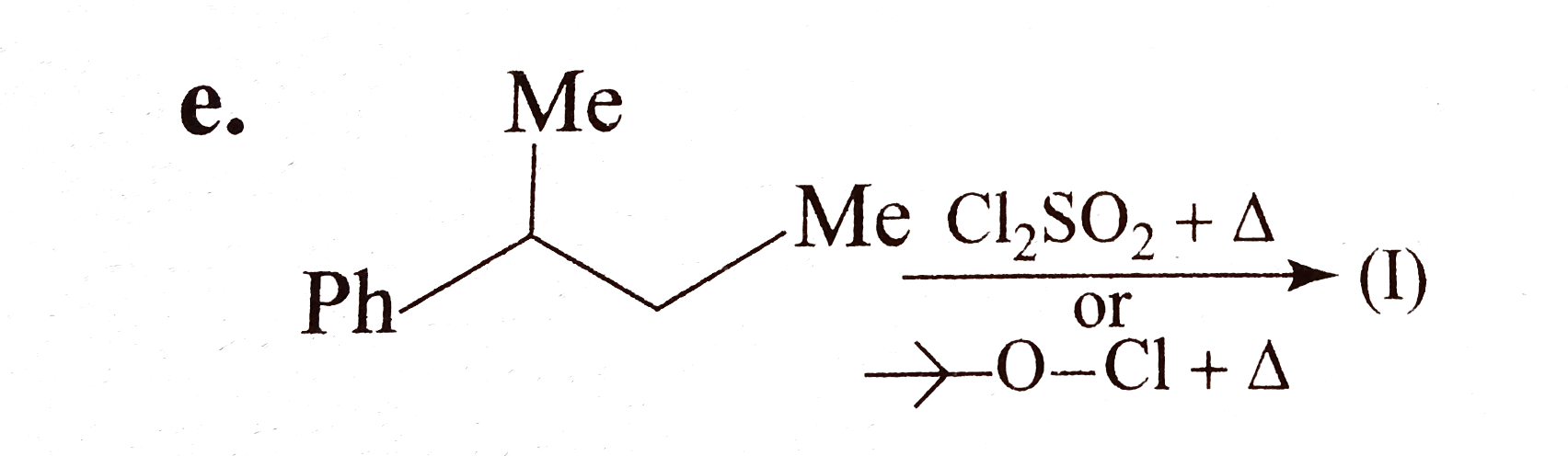
View SolutionWhich of the following reagents can be used for the reaction given below ?
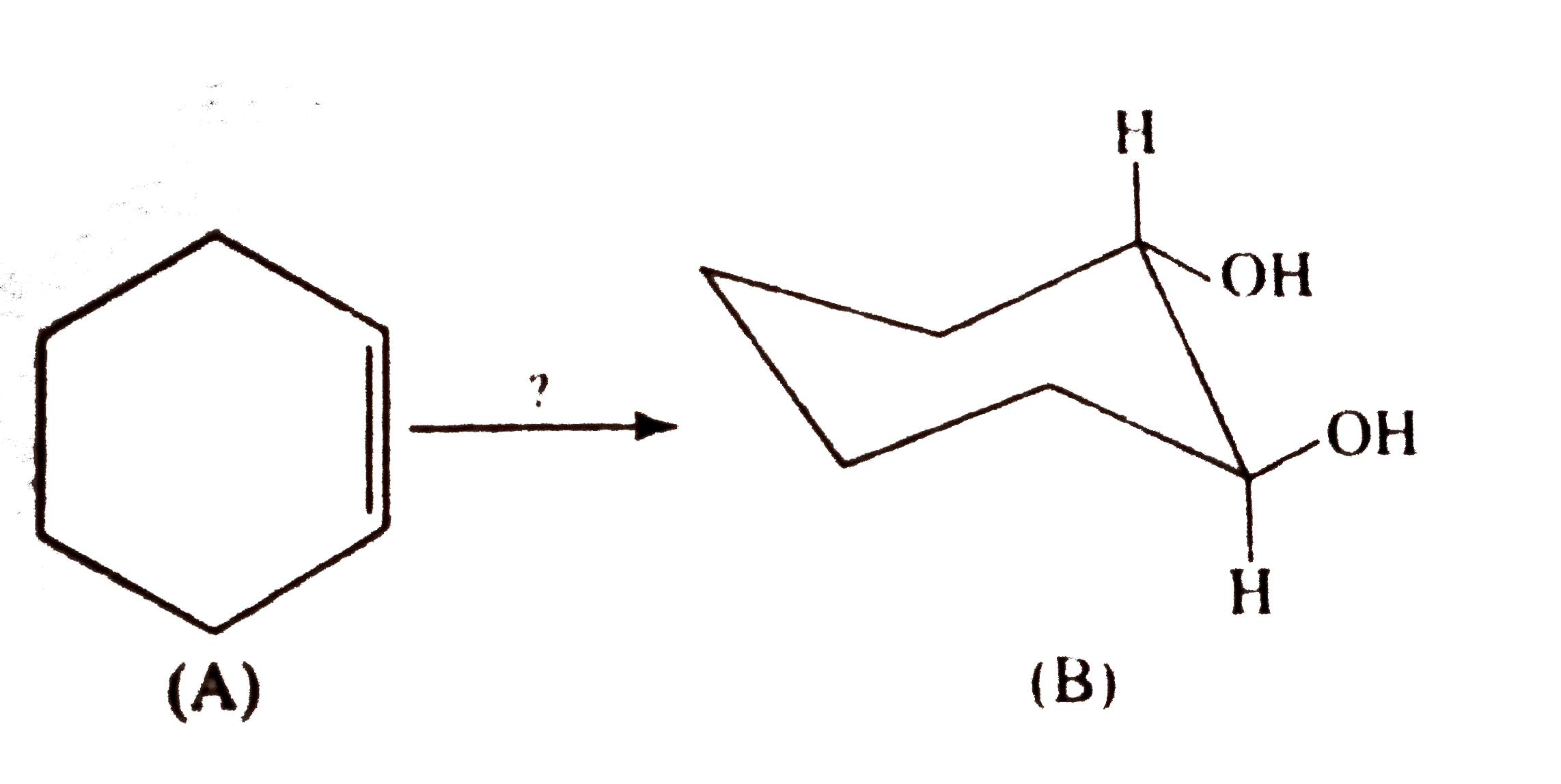
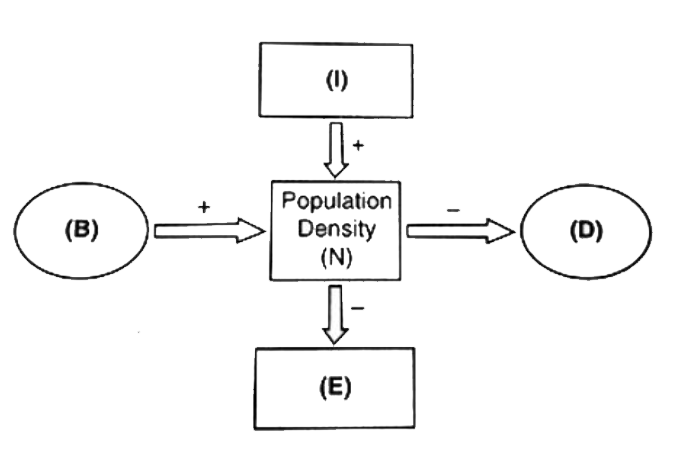
View SolutionDifferent factors affecting population density are given below . I ,B ,D and E represent :
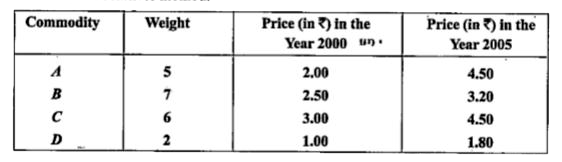
View SolutionThe quotations for four different commodities for the years 2000 and 2005 are given below. Calculate the index number for 2005, with 2000 as the base year, by using the weighted average of price relatives method.
View SolutionFor the reaction A+B→C+D, the initial rate for different reactions and initial concentration of reactants are given below:
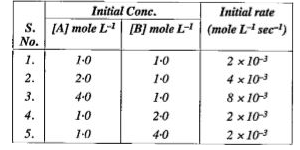
(i) What is the overall order of reaction ?
(ii) Write the rate law equation.
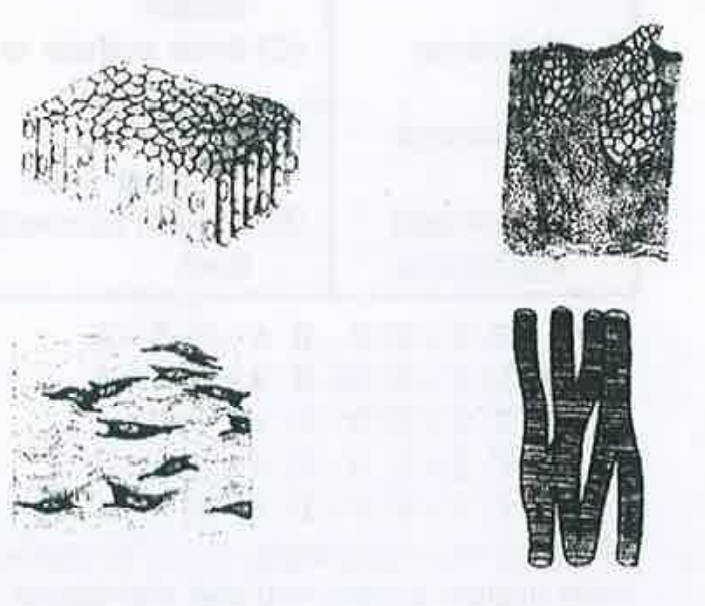
View SolutionThe four sketches (A, B, C and D) given below, represent four different types of animal tissues. Which one of these is correctly identified in the options given, along with its correct location and function ?

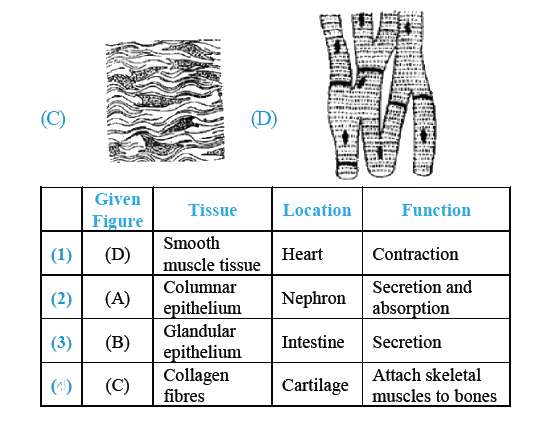
View SolutionThe four sketches A, B,C and D given below
represent four different types of animal tissues .
which one of these is correctly identified
in the options given along with its correct location and function ?
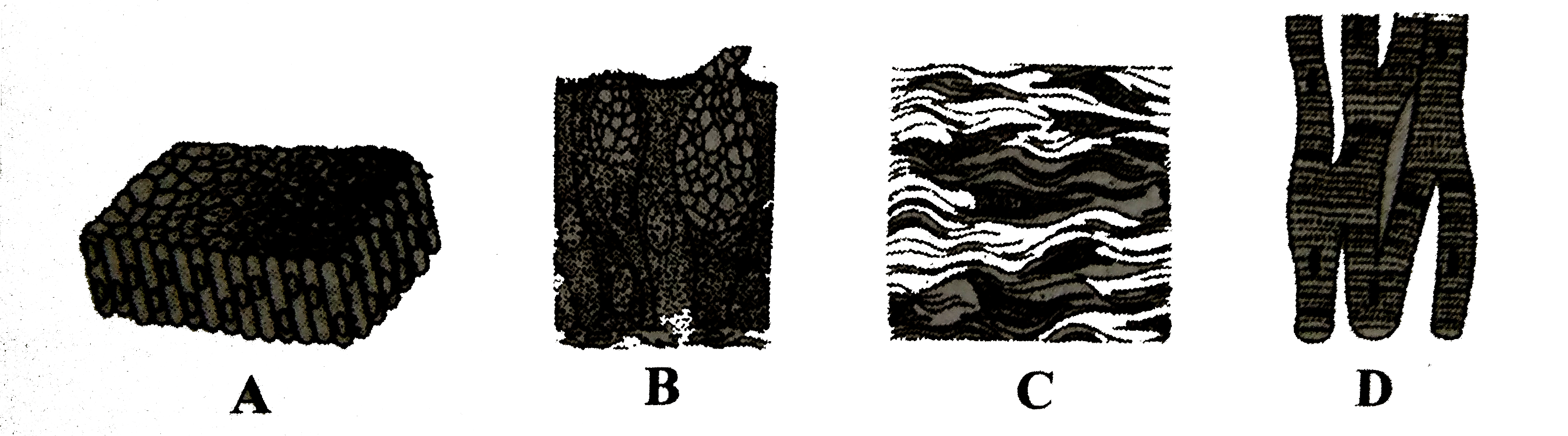
View SolutionThe four figures (A, B, C and D) given below represent four different types of animal tissues. Which one of these is correctly identified in the given options along with its correct location and function ?
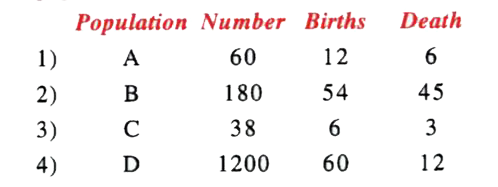
View SolutionThe births and deaths of four populations A, B, C and D in a year are given below. Which population has the highest growth rate?
View SolutionConsider the reaction, A+B→C+D.
The initial rate for different initial concentrations of the reactants are given below :
What are the orders with respect to A and B ?
View Solution
