Consider a uniform wire of length l. Cross-sectional area A, Young's modulus of the material of the wave is Y. Some information related to the wire is given in column-1 and dependence of the result is given in column-2. Then match the approprite choice between the columns and match the list given in options
Column-I Column-II(A) Let us suspend wire vertically from a (p) Young'sModulusrigud support and attach a mass m atits lower end. If the mass is slightly pulleddown and released, it executes S.H.Mof a time period which will depend on(B) Work done in stretching the wire up to length (q) elongation (x)l+xwill depend on(C) If the given is fixed between two rigid (r) length (l)supports and its temperature is decreasedthermal stress that develops in the wirewill depend on(D) If the wire is pulled at its ends equal and (s) area of cross-section (A) opposite forces of magnitude F so that itundergoes an elongation x, according toHook's law, F=kx, where k is the forceconstant.Force constant (k) of the wirewill depend on
The correct Answer is:(A)-p,r,s (B) -p,q,r,s, (C) -p(D)-p,r,s
To solve the problem, we need to match the items in Column-I with the corresponding dependencies in Column-II. Let's analyze each part step by step.
Step 1: Analyze Part (A)
Statement: Let us suspend the wire vertically from a rigid support and attach a mass m at its lower end. If the mass is slightly pulled down and released, it executes SHM of a time period which will depend on...
Solution:
The time period T of the simple harmonic motion (SHM) can be expressed as:
T=2π√mk
where k is the force constant. From the relationship of Young's modulus, we know:
k=YAL
Thus, substituting for k:
T=2π√mLYA
This shows that the time period depends on:
- Length l
- Area of cross-section A
- Young's modulus Y
Matching: (A) → (P), (R), (S)
Step 2: Analyze Part (B)
Statement: Work done in stretching the wire up to length l+x will depend on...
Solution:
The work done W in stretching the wire can be calculated using:
W=∫x0Fdx
where F=YALx. Thus,
W=∫x0YALxdx=YAL⋅x22=YAx22L
This indicates that the work done depends on:
- Young's modulus Y
- Area of cross-section A
- Length l
- Elongation x
Matching: (B) → (P), (Q), (R), (S)
Step 3: Analyze Part (C)
Statement: If the wire is fixed between two rigid supports and its temperature is decreased, the thermal stress that develops in the wire will depend on...
Solution:
The thermal stress σ can be expressed as:
σ=Y⋅αΔT
where α is the coefficient of linear expansion and ΔT is the change in temperature. The stress depends on Young's modulus Y but does not depend on the area or length explicitly in this context.
Matching: (C) → (P)
Step 4: Analyze Part (D)
Statement: If the wire is pulled at its ends with equal and opposite forces of magnitude F so that it undergoes an elongation x, according to Hook's law, F=kx, where k is the force constant. The force constant k of the wire will depend on...
Solution:
From the earlier derivation, we have:
k=YAL
Thus, k depends on:
- Young's modulus Y
- Area of cross-section A
- Length l
Matching: (D) → (P), (R), (S)
Final Matching Summary:
- (A) → (P), (R), (S)
- (B) → (P), (Q), (R), (S)
- (C) → (P)
- (D) → (P), (R), (S)
Statement: Let us suspend the wire vertically from a rigid support and attach a mass m at its lower end. If the mass is slightly pulled down and released, it executes SHM of a time period which will depend on...
The time period T of the simple harmonic motion (SHM) can be expressed as:
T=2π√mk
where k is the force constant. From the relationship of Young's modulus, we know:
k=YAL
Thus, substituting for k:
T=2π√mLYA
This shows that the time period depends on:
- Length l
- Area of cross-section A
- Young's modulus Y
Statement: Work done in stretching the wire up to length l+x will depend on...
The work done W in stretching the wire can be calculated using:
W=∫x0Fdx
where F=YALx. Thus,
W=∫x0YALxdx=YAL⋅x22=YAx22L
This indicates that the work done depends on:
- Young's modulus Y
- Area of cross-section A
- Length l
- Elongation x
Statement: If the wire is fixed between two rigid supports and its temperature is decreased, the thermal stress that develops in the wire will depend on...
The thermal stress σ can be expressed as:
σ=Y⋅αΔT
where α is the coefficient of linear expansion and ΔT is the change in temperature. The stress depends on Young's modulus Y but does not depend on the area or length explicitly in this context.
Statement: If the wire is pulled at its ends with equal and opposite forces of magnitude F so that it undergoes an elongation x, according to Hook's law, F=kx, where k is the force constant. The force constant k of the wire will depend on...
From the earlier derivation, we have:
k=YAL
Thus, k depends on:
- Young's modulus Y
- Area of cross-section A
- Length l
- (A) → (P), (R), (S)
- (B) → (P), (Q), (R), (S)
- (C) → (P)
- (D) → (P), (R), (S)
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.72
20 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Knowledge Check
A wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2L
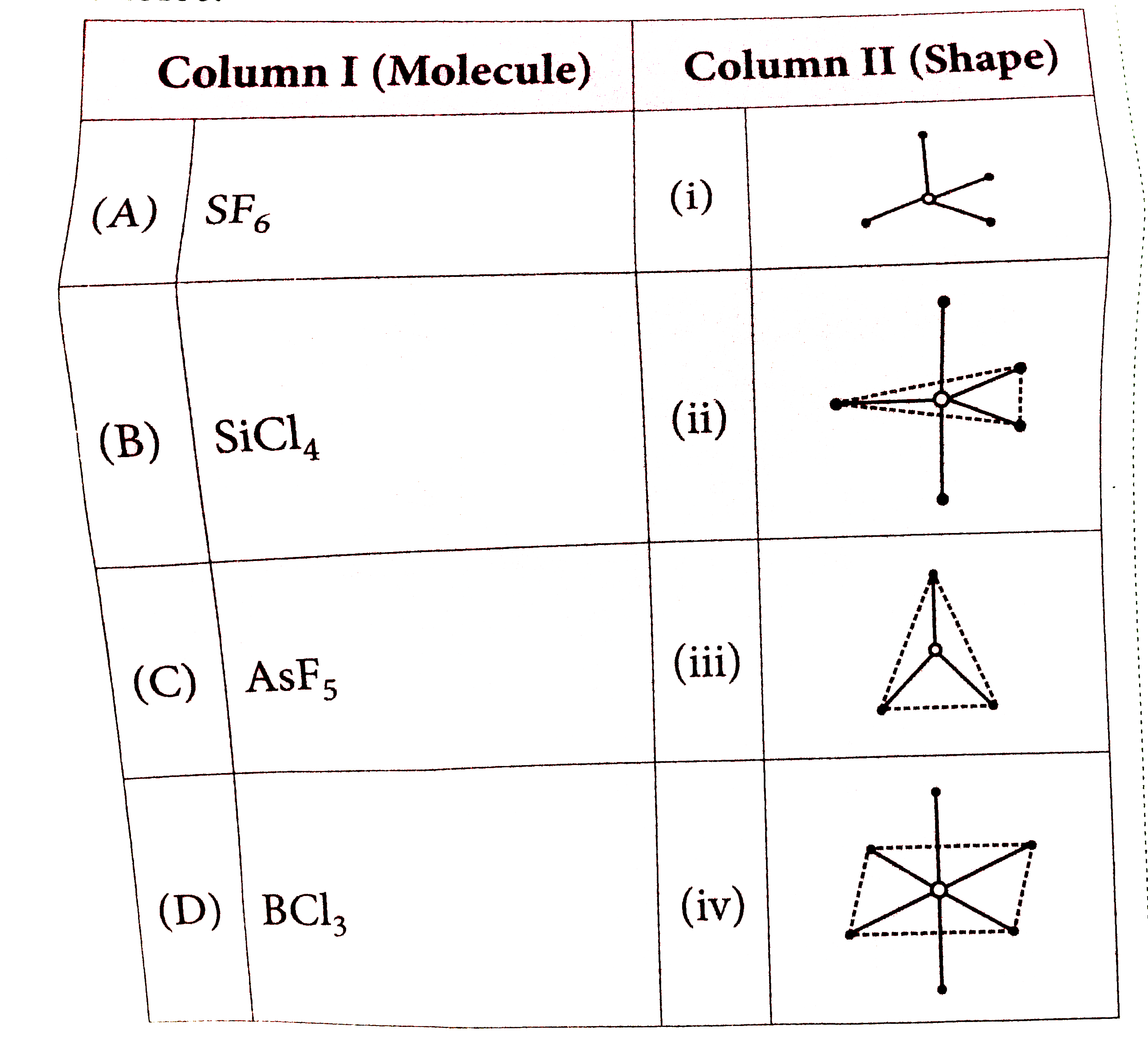
Match the complex ions given in column I with their colour given in column II and mark the appropriate choice .
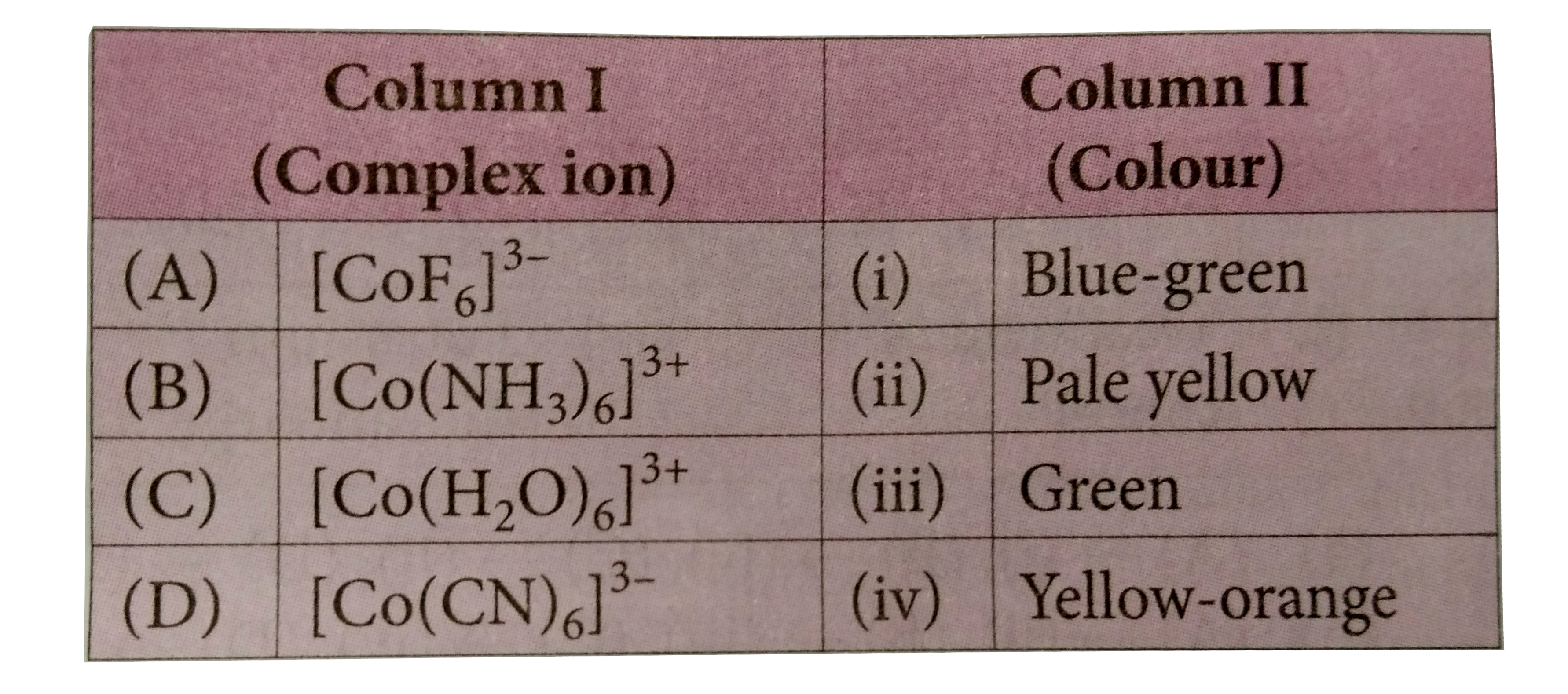
View SolutionMatch the compounds given in column I with the IUPAC names given in column II and mark the appropriate choice.
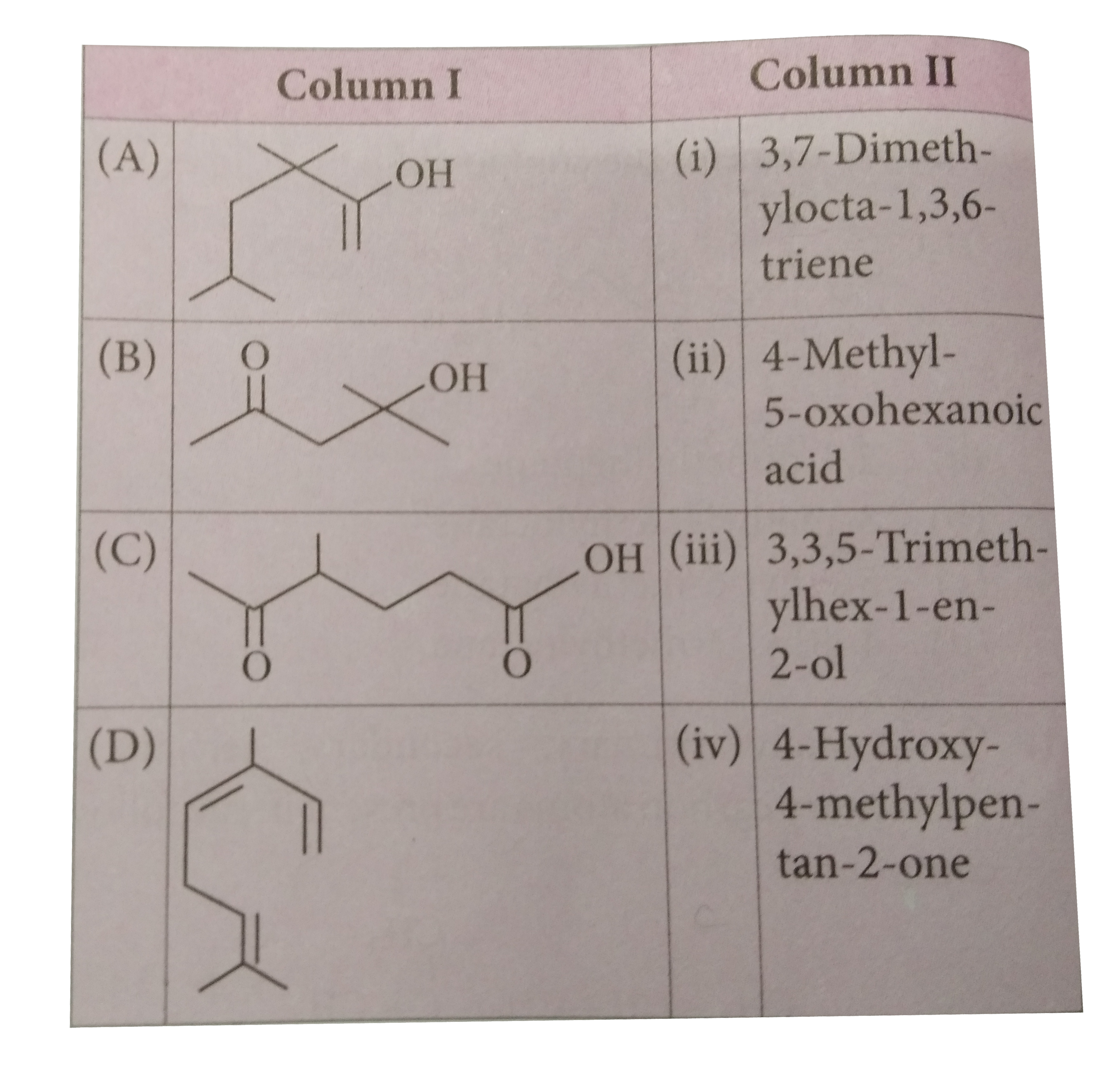
View SolutionMatch the constants given in column I with their values given in column II and mark the appropriate choice.
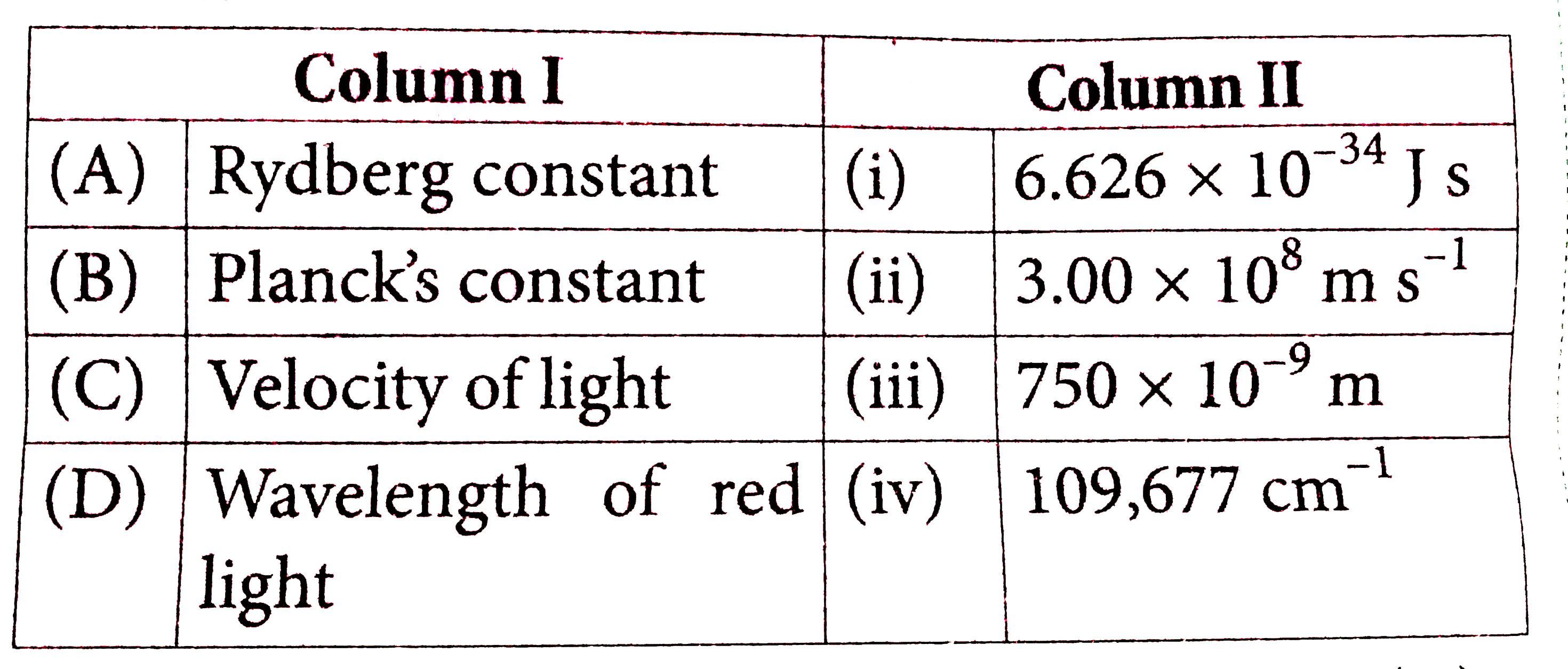
View SolutionMatch the atomic numbers of the elements given in column I with the periods given in column II and mark the appropriate choice.
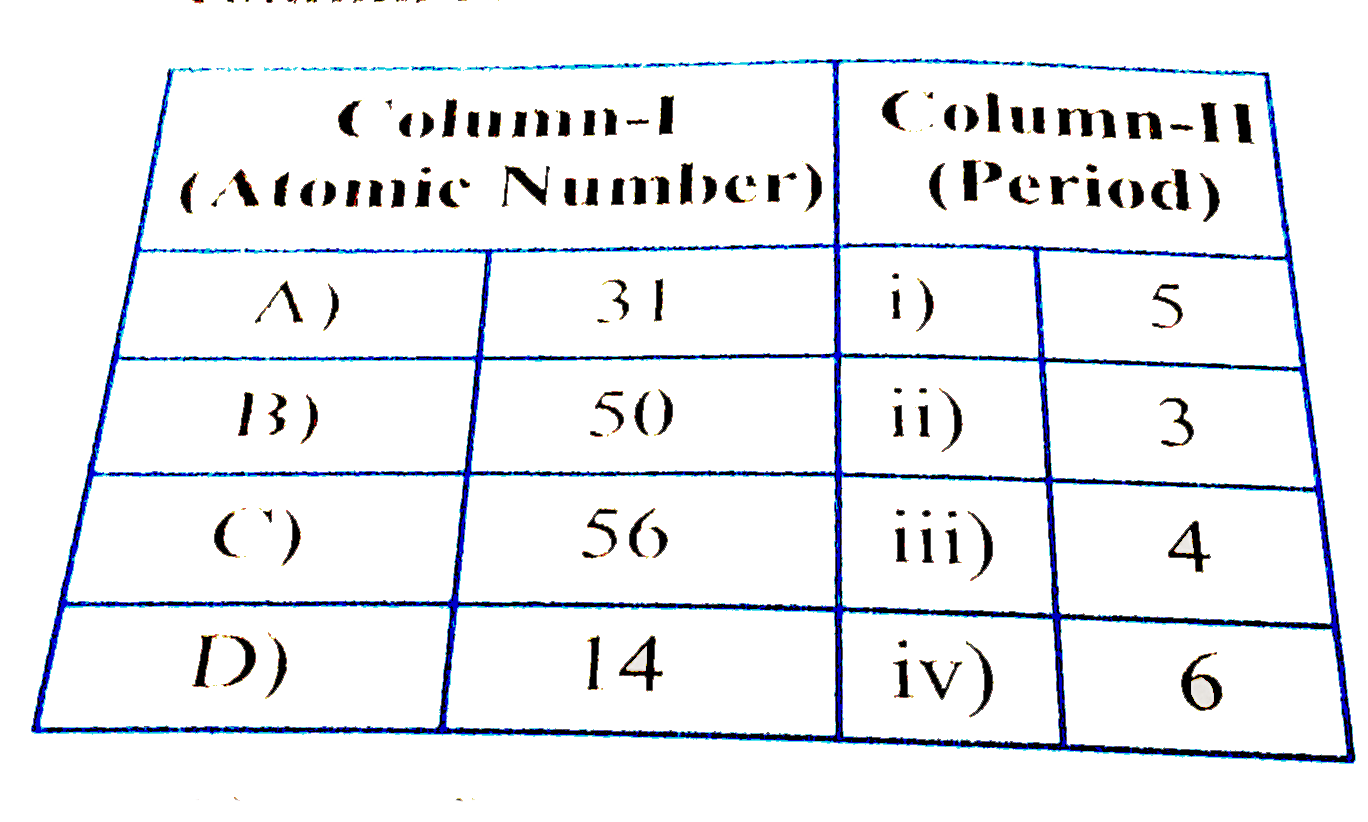
View SolutionMatch the atomic numbers of the elements given in column I with the periods given in column II and mark the appropriate choice.

View SolutionMatch the isomers given in column I with their names given in column II and mark the appropriate choice.
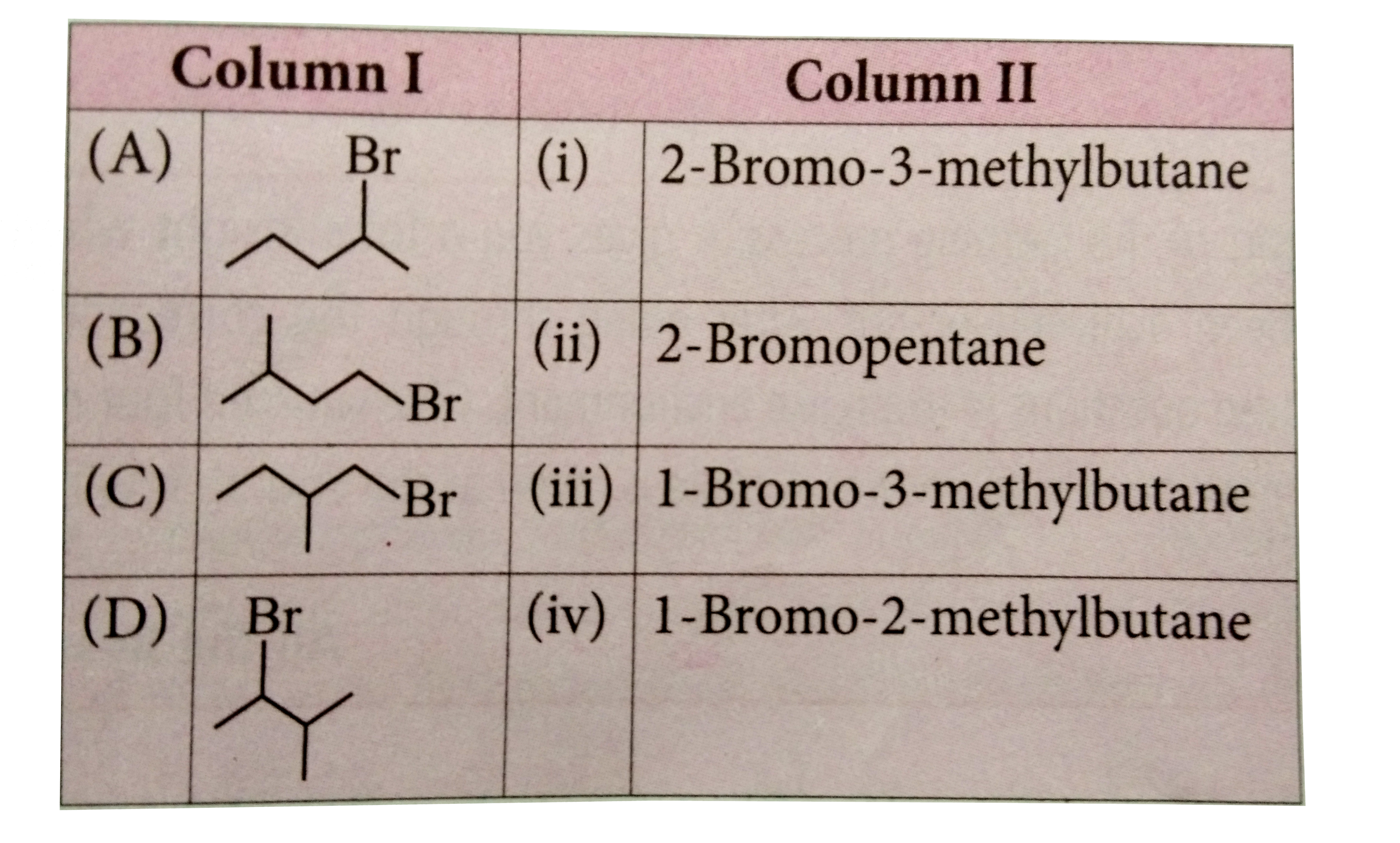
View SolutionMatch the alloys given in column I to the uses given in column II.

View Solution