When a small magnetising field H is applied to a magnetic material the intensity of magnetisation (I) is proportional to:
A. H−2
B. H1/2
C. H
D. H2
H−2
H1/2
H
H2
The correct Answer is:C
To solve the question, we need to determine how the intensity of magnetization (I) is related to the magnetizing field (H) when a small magnetizing field is applied to a magnetic material.
1. Understanding Intensity of Magnetization (I):
- The intensity of magnetization (I) is defined as the magnetic moment per unit volume of the material.
- Mathematically, it can be expressed as:
I=MV
where M is the magnetic moment and V is the volume.
2. Magnetic Susceptibility (χ):
- Magnetic susceptibility (χ) is a dimensionless quantity that indicates how much a material will become magnetized in an applied magnetic field.
- It is defined as:
χ=IH
Rearranging this gives:
I=χ⋅H
3. Proportional Relationship:
- For small magnetizing fields, the magnetic susceptibility (χ) is typically considered to be a constant for a given material.
- Therefore, we can express the relationship between I and H as:
I∝H
This indicates that the intensity of magnetization (I) is directly proportional to the magnetizing field (H).
4. Conclusion:
- From the above analysis, we can conclude that when a small magnetizing field H is applied to a magnetic material, the intensity of magnetization (I) is proportional to H.
- Thus, the correct answer to the question is C. H.
- The intensity of magnetization (I) is defined as the magnetic moment per unit volume of the material.
- Mathematically, it can be expressed as:
I=MV
where M is the magnetic moment and V is the volume.
- Magnetic susceptibility (χ) is a dimensionless quantity that indicates how much a material will become magnetized in an applied magnetic field.
- It is defined as:
χ=IH
Rearranging this gives:
I=χ⋅H
- For small magnetizing fields, the magnetic susceptibility (χ) is typically considered to be a constant for a given material.
- Therefore, we can express the relationship between I and H as:
I∝H
This indicates that the intensity of magnetization (I) is directly proportional to the magnetizing field (H).
- From the above analysis, we can conclude that when a small magnetizing field H is applied to a magnetic material, the intensity of magnetization (I) is proportional to H.
- Thus, the correct answer to the question is C. H.
Topper's Solved these Questions
Similar Questions
The dimensional formula for magnetising field H is
View SolutionArea under magnetic field induction and magnetizing field intensity (B-H) loop gives the
View SolutionThe ratio of magnetisation I to the magnetic field intensity H is
View SolutionThe magnetic induction and magnetising field intensity in a sample of magnetic material are B and H respectively . The magnetic susceptibility of the material is
View SolutionThe following figure shows the variation of intensity of magnetisation versus the applied magnetic field intensity, H, for two magnetic materials A and B :
(a) Identify the materials A and B.
(b) Why does the material B, have a larger susceptibility than A, for a given field at constant temperature?

View SolutionA magnetising field intensity of 10Am−1 is applied on a sample of paramagnetic material of susceptibility 1999. The net magnetic field inside the material is
View SolutionThe following figure shows the variation of intensity of magnetisation I versus the applied magnetic field intensity H, for two magnetic materials A and B
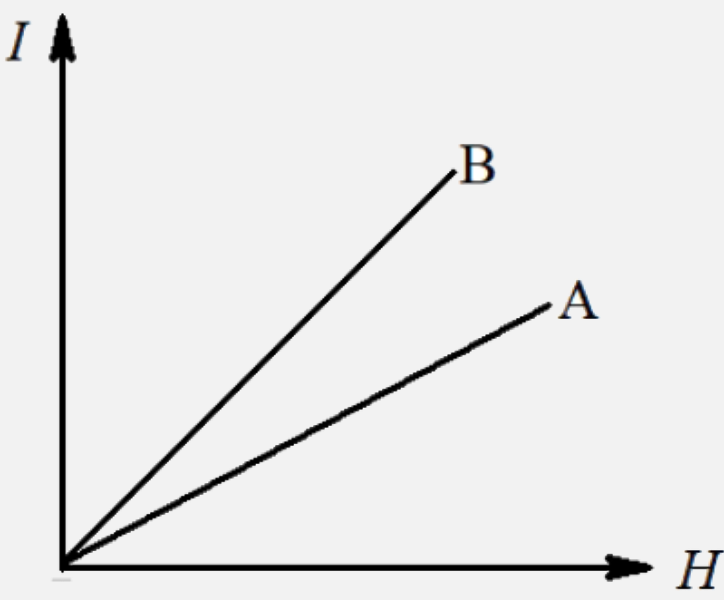
Which of the material have a larger susceptibility for a given field at constant temperature?
View SolutionA sample of cast iron exhibits a magnetic field B = 0.5 T , when intensity of magnetising field (H) is 10Am.
(i) Find permeability of iron .
(ii) What would be the field in air for this value of H ?
View SolutionAssertion: Suceptibility is defined as the ration of intensity of magnetisation I to magnetic intensituy H.
Reason: Greater the value of susceptibility smaller the value of intensity of magnetisation I.
View SolutionIf H be the harmonic mean of a and b then find the value of Ha+Hb−2
View Solution
