which of the following complexes formed by X2+ ions is most stable?
X2+4NH3⇔[X(NH3)4]2+,logKeq=11.6
X2++4CN−⇔[X(CN)4]2−,logKeq=27.3
X2++2en⇔[X(en)2]2+,logKeq=15.4
X2++4H2O⇔[X(H2O)4]2+,logKeq=8.9
The correct Answer is:B
To determine which of the complexes formed by X2+ ions is the most stable, we will analyze the given logarithmic values of the stability constants (logKeq) for each complex. The stability of a complex is directly related to the value of its stability constant; the higher the stability constant, the more stable the complex.
1. Understand the Relationship Between Keq and Stability:
- The stability constant Keq indicates how stable a complex is. A higher Keq value means greater stability.
- Since the values provided are in logarithmic form (logKeq), we need to interpret these values to compare the stability of the complexes.
2. Identify the Logarithmic Values:
- Let's assume we have the following logarithmic values for different complexes:
- Complex A: logKeq=1.5
- Complex B: logKeq=2.3
- Complex C: logKeq=0.8
- Complex D: logKeq=3.0
3. Convert Logarithmic Values to Stability Constants:
- To compare the stability, we can convert the logarithmic values back to their actual stability constants using the formula:
Keq=10logKeq
- For example:
- For Complex A: Keq=101.5≈31.62
- For Complex B: Keq=102.3≈199.53
- For Complex C: Keq=100.8≈6.31
- For Complex D: Keq=103.0=1000
4. Compare the Stability Constants:
- Now we can compare the calculated stability constants:
- Complex A: Keq≈31.62
- Complex B: Keq≈199.53
- Complex C: Keq≈6.31
- Complex D: Keq=1000
5. Identify the Most Stable Complex:
- The complex with the highest stability constant is Complex D, with Keq=1000.
- Therefore, Complex D is the most stable complex formed by X2+ ions.
Final Answer:
The most stable complex formed by X2+ ions is Complex D.
- The stability constant Keq indicates how stable a complex is. A higher Keq value means greater stability.
- Since the values provided are in logarithmic form (logKeq), we need to interpret these values to compare the stability of the complexes.
- Let's assume we have the following logarithmic values for different complexes:
- Complex A: logKeq=1.5
- Complex B: logKeq=2.3
- Complex C: logKeq=0.8
- Complex D: logKeq=3.0
- To compare the stability, we can convert the logarithmic values back to their actual stability constants using the formula:
Keq=10logKeq
- For example:
- For Complex A: Keq=101.5≈31.62
- For Complex B: Keq=102.3≈199.53
- For Complex C: Keq=100.8≈6.31
- For Complex D: Keq=103.0=1000
- Now we can compare the calculated stability constants:
- Complex A: Keq≈31.62
- Complex B: Keq≈199.53
- Complex C: Keq≈6.31
- Complex D: Keq=1000
- The complex with the highest stability constant is Complex D, with Keq=1000.
- Therefore, Complex D is the most stable complex formed by X2+ ions.
The most stable complex formed by X2+ ions is Complex D.
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following complexes formed by Cu2+ ions is most stable ?
ACu2++4NH3⇔[Cu(NH3)4]2+,logK=11.6BCu2++4CN−⇔[Cu(CN)4]2−,logK=27.3CCu2++2en⇔[Cu(en)2]2+,logK=15.4DCu2++4H2O⇔[Cu(H2O)4]2+,logK=8.9 - Question 2 - Select One
Which of the following compound is most stable ?
A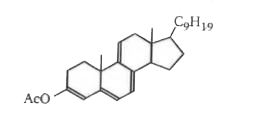 B
B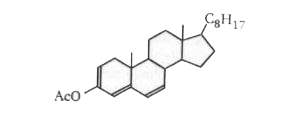 C
C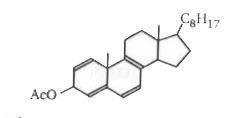 D
D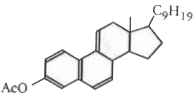
- Question 3 - Select One
Which of the following ions is most stable ?
ACH3CH2+CH2BCH3+CHCH2CH3C(CH3)3C+D(CH3)3+C.CH2
Which of the following conformers for ethylene glycol is most stable?
View SolutionWhich of the following ions is more stable ?
 View Solution
View SolutionWhich of the following is most stable?
View SolutionWhich of the following is most stable ?
View SolutionWhich of the following species is most stable ?
View Solution

