For positive integers $K = 1, 2, 3, \dots \dots \dots . n$ Let $S_{k}$ denotes the area of $Δ O A B_{k}$ (where $O$ is the origin) such that $∠ A O B_{k} = \frac{K π}{2 n}, O A = 1$ and $O B_{K} = K$ . The value of the $lim n \to \infty \frac{1}{n^{2}} . n \sum K = 1 S_{K}$ is

Video Solution

Text Solution

Verified by Experts

The correct Answer is:0

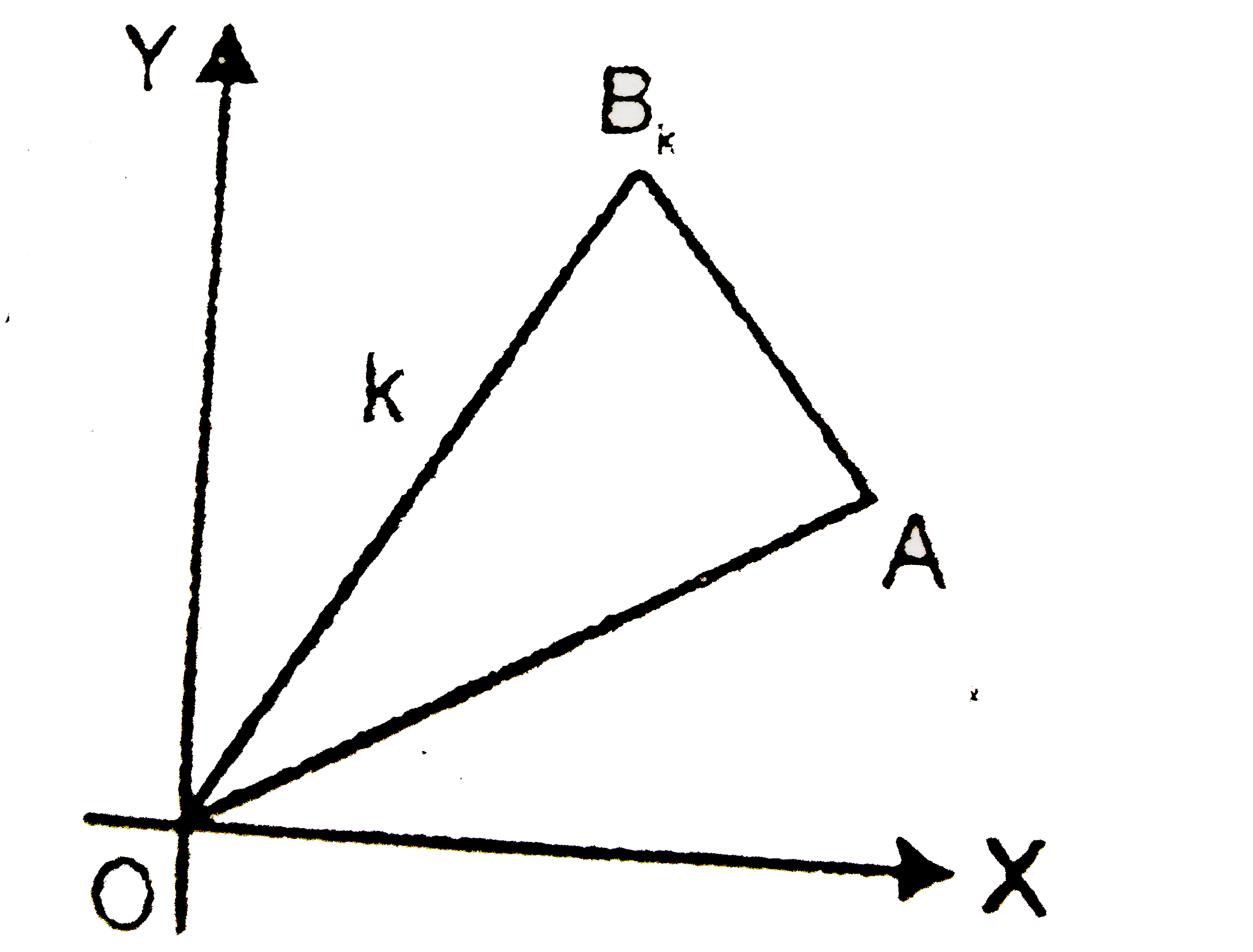
$O B_{k} = k, ∠ A O B_{k} = \frac{k π}{2 n}$
$S_{k} = \frac{1}{2} k sin \frac{k π}{2 n}$ (using $Δ = \frac{1}{2} ∣ ∣ ∣ \int ∣ ∣ ∣ h η$ )
$∴ L = lim n \to \infty \frac{1}{n^{2}} n \sum k = 1 S_{k} = \frac{k}{2 n^{2}} \infty \sum k = 1 sin \frac{k π}{2 n}$
$= \frac{1}{2 n} \infty \sum k = 1 \frac{k}{n} sin \frac{k π}{2 n} = \frac{1}{2} \int_{0}^{1} x . sin \frac{π x}{2} d x$
$= \frac{1}{2} [\frac{- 2}{π} \times cos \frac{π x}{2} ∣_{0}^{1} + \frac{2}{π} \int_{0}^{1} cos \frac{π x}{2} d x]$
$= \frac{1}{2} [0 + \frac{2}{π} \frac{.2}{π} {(sin \frac{π x}{2})}_{0}^{1}] = \frac{2}{π}$

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-TEST PAPERS-MATHEMATICS

Home
Ask Doubt
Profile