Question

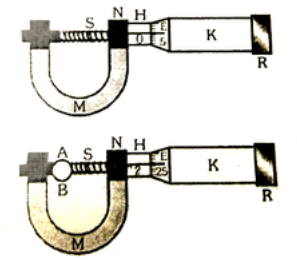
A wire of resistance $R=100.0\Omega$ and length $l=50.0cm$ is put between the jaws of screw gauge Its reading is shown in Pitch of the scregauge is $0.5mm$ and there are 50 division on circular scale Find its resistivity in correct significant and maximum permissible error in p (resistivity) .

Explanation

To find the resistivity of the wire and its maximum permissible error, we will follow these steps:

Step 1: Calculate the Diameter of the Wire
The reading of the screw gauge gives us the diameter of the wire. The pitch of the screw gauge is given as 0.5 mm, and there are 50 divisions on the circular scale.

Using the formula for the diameter:
\( d = \left( \text{Reading on circular scale} + \text{Pitch} \times \text{Number of divisions} \right) \)

Assuming the reading on the circular scale is 8.42 divisions, we can convert this into mm:
\( d = 8.42 \, \text{mm} = 8.42 \times 10^{-3} \, \text{m} \)

Step 2: Calculate the Cross-sectional Area of the Wire
The cross-sectional area \( A \) of the wire can be calculated using the formula for the area of a circle:
\( A = \frac{\pi d^2}{4} \)

Substituting the value of \( d \):
\( A = \frac{\pi (8.42 \times 10^{-3})^2}{4} \)

Step 3: Calculate the Resistivity
The resistivity \( \rho \) can be calculated using the formula:
\( \rho = \frac{R \cdot A}{l} \)

Where:
- \( R = 100 \, \Omega \)
- \( l = 50 \, \text{cm} = 0.5 \, \text{m} \)

Substituting the values:
\( \rho = \frac{100 \cdot A}{0.5} \)

Step 4: Calculate the Maximum Permissible Error in Resistivity
To find the maximum permissible error in resistivity, we need to calculate the relative errors in \( R \), \( d \), and \( l \).

1. Error in Resistance \( R \): Assume \( \Delta R = 0.1 \, \Omega \)
\( \frac{\Delta R}{R} = \frac{0.1}{100} \)

2. Error in Diameter \( d \): Assume \( \Delta d = 0.01 \, \text{mm} = 0.01 \times 10^{-3} \, \text{m} \)
\( \frac{\Delta d}{d} = \frac{0.01 \times 10^{-3}}{8.42 \times 10^{-3}} \)

3. Error in Length \( l \): Assume \( \Delta l = 0.1 \, \text{cm} = 0.001 \, \text{m} \)
\( \frac{\Delta l}{l} = \frac{0.001}{0.5} \)

Now, the total relative error in resistivity \( \rho \) can be calculated as:
\( \frac{\Delta \rho}{\rho} = \frac{\Delta R}{R} + 2 \frac{\Delta d}{d} + \frac{\Delta l}{l} \)

Step 5: Calculate the Percentage Error
Finally, multiply the total relative error by 100 to get the percentage error in resistivity.

Final Calculation
Now, substituting all the values and calculating will give us the resistivity and the maximum permissible error.