A sample of mixture of A(g),B(g) and C(g) under equilibrium has a mean molecular weight (observed ) is 80.
The equilibrium is A(g)(mol.wt.=100)⇔B(g)(mol.wt.=60)+C(g)(mol.wt.=40)
Find the degree of dissociation α for A(g)
0.25
0.5
0.75
0.8
The correct Answer is:A
To solve the problem of finding the degree of dissociation (α) for A(g) in the equilibrium reaction:
A(g)⇌B(g)+C(g)
given the mean molecular weight of the mixture is 80, we can follow these steps:
Step 1: Define the Initial Conditions
Let:
- The initial number of moles of A = c
- The initial number of moles of B = 0
- The initial number of moles of C = 0
Step 2: Define the Change in Moles at Equilibrium
At equilibrium, if α is the degree of dissociation of A, then:
- Moles of A at equilibrium = c(1−α)
- Moles of B at equilibrium = cα
- Moles of C at equilibrium = cα
Step 3: Calculate Total Moles at Equilibrium
Total moles at equilibrium = Moles of A + Moles of B + Moles of C
=c(1−α)+cα+cα=c(1+α)
Step 4: Calculate the Mean Molecular Weight
The mean molecular weight (MW) of the mixture can be calculated using the formula:
Mean Molecular Weight=Total MassTotal Moles
The total mass of the mixture at equilibrium is:
Total Mass=Moles of A×Molecular Weight of A+Moles of B×Molecular Weight of B+Moles of C×Molecular Weight of C
=c(1−α)×100+cα×60+cα×40
=c(100(1−α)+60α+40α)
=c(100−100α+100α)=c(100)
Thus, the total mass = 100c.
Now, substituting into the mean molecular weight formula:
80=100cc(1+α)
Step 5: Simplify the Equation
Cancelling c from numerator and denominator:
80=1001+α
Step 6: Solve for α
Rearranging the equation:
1+α=10080
1+α=1.25
α=1.25−1=0.25
Conclusion
The degree of dissociation α for A(g) is:
α=0.25or14
Let:
- The initial number of moles of A = c
- The initial number of moles of B = 0
- The initial number of moles of C = 0
At equilibrium, if α is the degree of dissociation of A, then:
- Moles of A at equilibrium = c(1−α)
- Moles of B at equilibrium = cα
- Moles of C at equilibrium = cα
Total moles at equilibrium = Moles of A + Moles of B + Moles of C
=c(1−α)+cα+cα=c(1+α)
The mean molecular weight (MW) of the mixture can be calculated using the formula:
Mean Molecular Weight=Total MassTotal Moles
Total Mass=Moles of A×Molecular Weight of A+Moles of B×Molecular Weight of B+Moles of C×Molecular Weight of C
=c(1−α)×100+cα×60+cα×40
=c(100(1−α)+60α+40α)
=c(100−100α+100α)=c(100)
80=100cc(1+α)
Cancelling c from numerator and denominator:
80=1001+α
Rearranging the equation:
1+α=10080
1+α=1.25
α=1.25−1=0.25
The degree of dissociation α for A(g) is:
α=0.25or14
Topper's Solved these Questions
Similar Questions
Degree of dissociation (α)
α are the number of moles which are dissociating from 1 mole of given reactants and gas density measurements can be used to determine the degree of dissociatin. Let us take a general case where one molecule of a substance A splits up into n molecules of A(g) on heating i.e.,
An(g)⇔nA(g)
t=0a
t=teqa−xnx α=xa⇒x=aα
a−aαnaα
Total number of Moles =a−aα+naα
=[1+(n−1)α]a
Observed molecular weight of molar mass of the mixture
Mmixture=MAn[1+(n−1)α],MAn= Molar mass of An
A sample of mixture A(g), B(g)and C(g) under equlibrium has a mean molecular weight (observed) of 80.
The equlibrium is
A(g)⇔B(g)+C(g)
(Mol wt =100) (Mol. wt=60) (Mol. wt=40) Calculate the Degree of dissociation for given reaction.View SolutionA sample of mixture of A(g),B(g) and C(g) under equilibrium has mean molecular mass equal to 80. The equilibrium is :
A(g)⇔B(g)+C(g)
If initially 4 mole of 'A' gas is present then total number of mole at equilibrium is :
[MA=100,MB=60,MC=40]View SolutionA(g)+3B(g)→4C(g)Initially concentration of A is equal to that of B. The equilibrium concentrations of A and C are equal. Kc is :
View SolutionAttainment of the equilibrium A(g)⇔2C(g)+B(g)gave the following graph . Find the correct option .(%dissociation=Frationdissoiatedxx100)
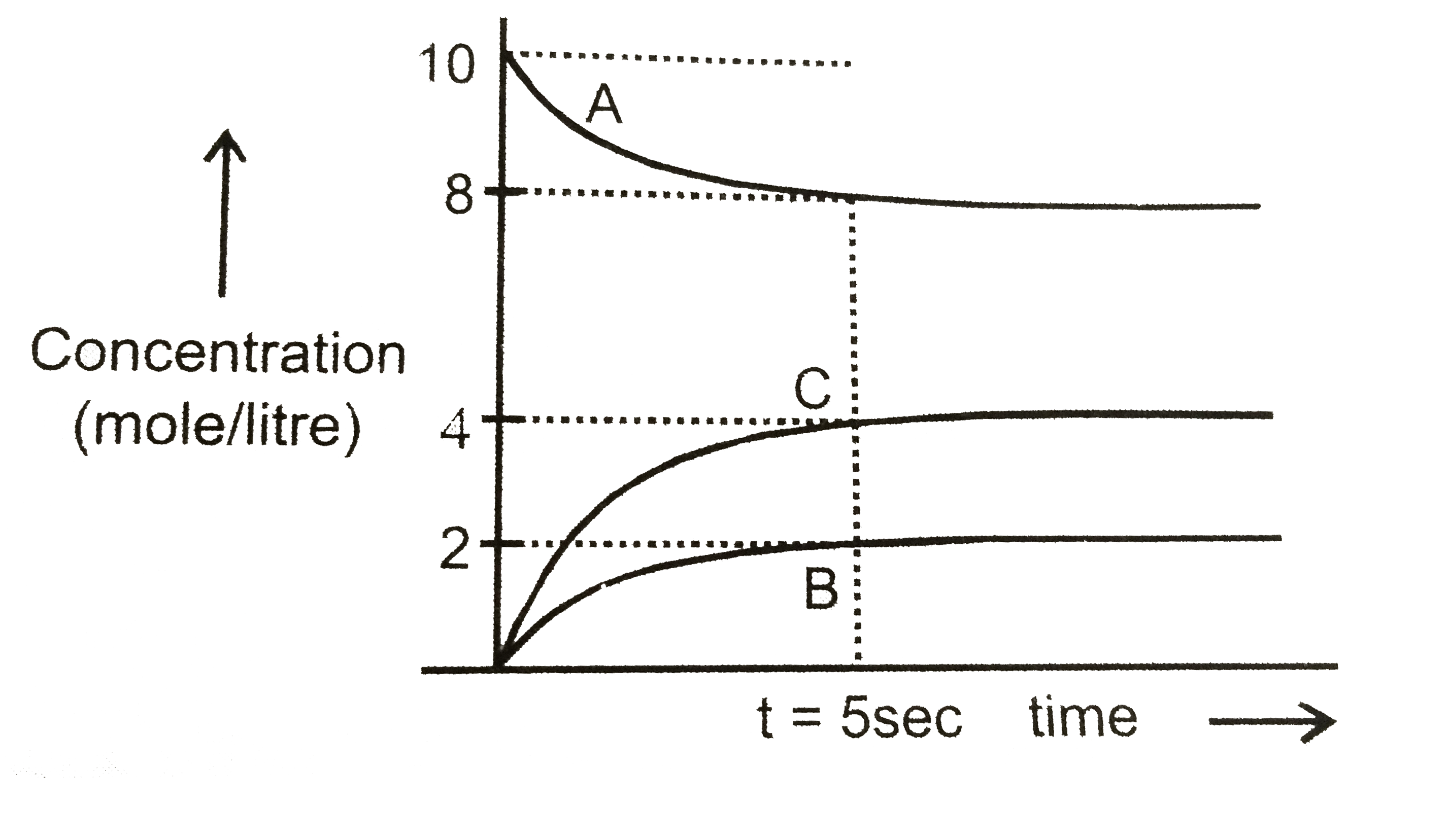 View Solution
View SolutionA mixture of 1.65×1021 molecules of X and 1.85×1021 molecules of Y weighs 0.688 g . The mol . wt . Of X is (Assume mol . wt . Of Y is 187)
View SolutionConsider the imaginary equilibrium 4A(g)+5B(g)⇔4X(g)+6Y(g), The unit of equilibrium constant KC is
View SolutionConsider the following equilibrium
2AB(g)⇔A2(g)+B2(g)
The vapour density of the equilibrium mixture does not depend uponView SolutionA mixture of O2 and gas Y (mol.wt.80) in the mole ratio a:b has a mean molecular weight 40. What would be mean molecular weight, if the gases are mixed in the ratio b:a under identical conditions ? ( gases are ideal)
View SolutionA mixture of O2 and gas Y (mol.wt.80) in the mole ratio a:b has a mean molecular weight 40. What would be mean molecular weight, if the gases are mixed in the ratio b:a under identical conditions ? ( gases are )
View SolutionAn aqueous solution has a density of 1.37g/ml. If molecular wt of solute is 68.5g/mol, then calculate molarity of solution.
View Solution

