(a) Draw the ray diagram showing the geometry of formation of the image of a point object situated on the principal axis, and on the convex side, of a spherical of radius of curvature $R$ . Taking the rays as incident from a medium of refractive index $n_{1}$ to anothr of refractive index $n_{2}$ show that $\frac{n_{2}}{v} - \frac{n_{1}}{u} = \frac{n_{2} - n_{1}}{R}$ where the symbols have their usual meaning.
(b) Use this relation to obtain the (thin) lens maker's formula.

Text Solution

Verified by Experts

Refraction of spherical surface :
(a) Sign conventions : (i) All distances are measured from the pole of the spherical surface.
(ii) Distances measured in the direction of incident light are taken positive.
(iii) Distances measured in the opposite direction of incident light are negative.
Assumptions : (i) The object is a point object placed on the principal axis.
(ii) Aperture of the refracting surface is small.
(iii) Angle of incidence and angle of refraction are small
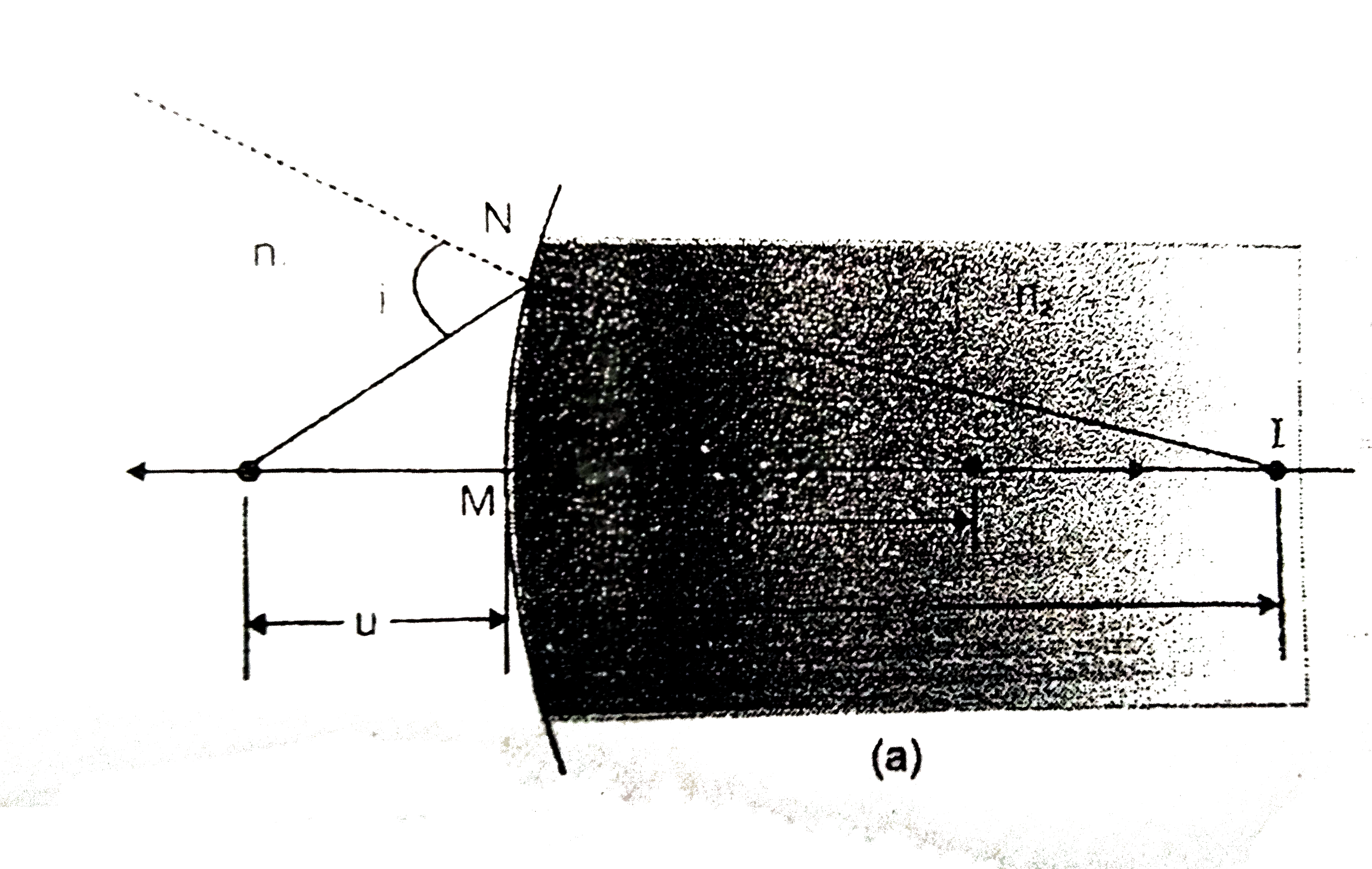
Figure $(a)$ shows the geometry of formation of image $I$ of an object $O$ on the principal axis of a spherical surface with centre of curvature $C$ , and radius of curvature $R$ . The rays are incident from a medium of refractive index $n_{1}$ , to another of refractive index $n_{2}$ . As before, we take the aperture (or the lateral size) of the surface to be small compared to other distances involved, so that small angle approximation can be made. In particular, $N M$ will be taken to be nearly equal to the length of the perpendicular from the point $N$ on the principal axis. We have, for small angles,
$tan ∠ N O M = \frac{M N}{O M}$
$tan ∠ N C M = \frac{M N}{M C}$
$tan ∠ N L M = \frac{M N}{M I}$
Now, for $Δ N O C$ , $I$ is the exterior angle.
Therefore, $i = ∠ N O M + ∠ N C M$
$i = \frac{M N}{O M} + \frac{M N}{M C}$
similarly
$r = ∠ N C M - ∠ N L M$
$i . e . r = \frac{M N}{M C} - \frac{M N}{M I}$
Now, by Snell's law
$n_{1} sin i = n_{2} sin r$
or for small angles
$n_{1} i = n_{2} r$
Substituting $i$ and $r$ from Eqs we get
$\frac{n_{1}}{O M} + \frac{n_{2}}{M I} = \frac{m_{2} - n_{1}}{M C}$
Here, $O M, M I$ and and $M C$ represent magnitudes of distances. Applying the cartesian convention, substuting sign convention,
$O M = - μ, M I = + v, M C = + R$
substituting these in Eq. we get,
$\frac{n_{2}}{v} - \frac{n_{1}}{u} = \frac{n_{2} - n_{1}}{R}$
Equation gives us a relation between object image distance in terms of refractive index of the medium and the radius of cuvature of the curved spherical, It holds for any curved spherical surface.

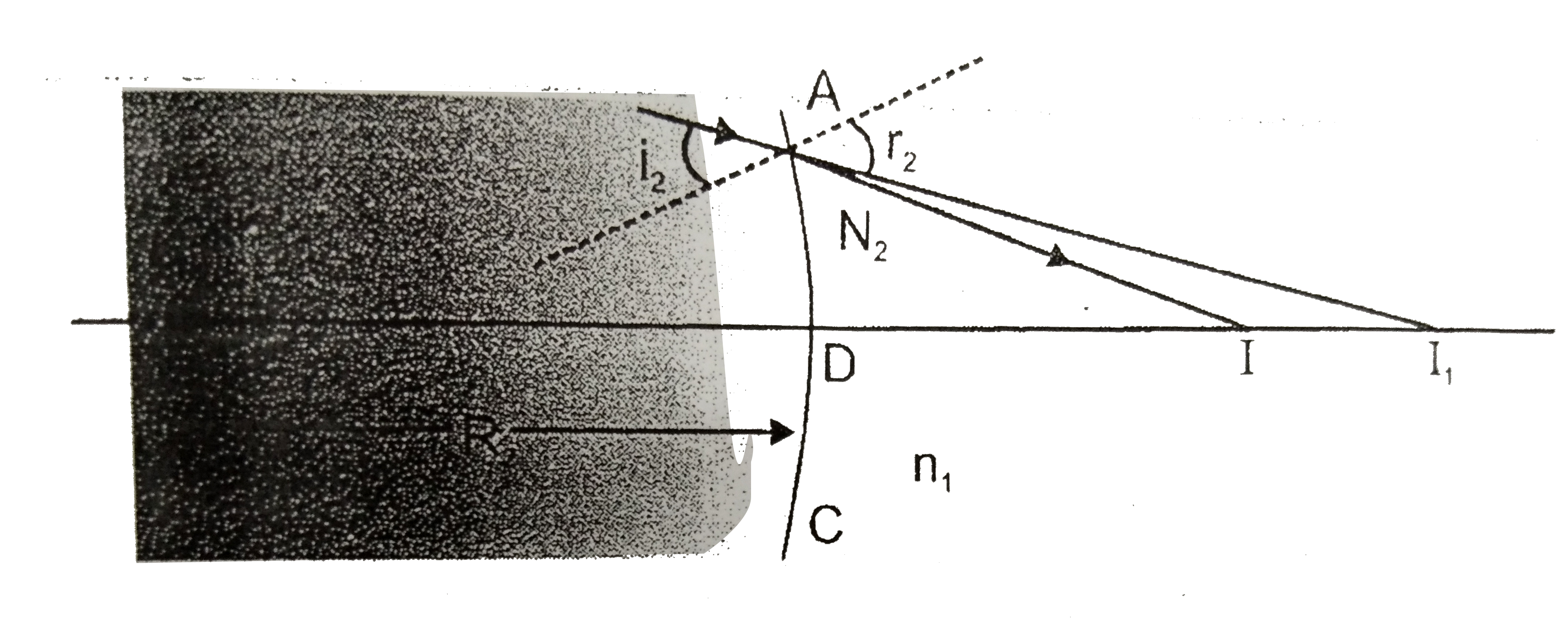
(b) Lens Maker's Formula :
The first refractive surface forms the image $I_{1}$ of object $O$ . The image $I_{1}$ acts as a virtual object for the second surface that forms the image at $I$ [Fig. $(b)$ ]. Applying to the first interface $A B C$ , we get
$\frac{n_{1}}{O B} + \frac{n_{1}}{D I} = \frac{n_{2} - n_{1}}{D C_{21}} ... ... (i)$
A similar procedure applied to the second interface $A D C$ gives,
$- \frac{n_{2}}{D I_{1}} + \frac{n_{1}}{D I} = \frac{n_{2} - n_{1}}{D C_{2}} ... ... ... (i i)$
For a thin lens, $B I_{1} = D I_{1}$ .
Adding Eqs. $(i)$ and $(i i)$ , we get
$\frac{n_{1}}{O B} + \frac{n_{1}}{D I} = (n_{2}) (\frac{1}{B C_{1}} + \frac{1}{D C_{2}})$
Suppose the object is at infinity,
$i . e ., O B \to \infty$ and $D I = f$ , Eq. $(i i)$ gives

$\frac{n_{1}}{f} = (n_{2} - n_{1}) (\frac{1}{B C_{1}} + \frac{1}{D C_{2}}) ... ... ... (i i i)$
The point where image of an object placed at infinity is formula is called the focus $F$ , of the lens and the distance $f$ given its focal length. $A$ lens has two foci, $F$ and $F'$ , on either side of it . By the sign convention, $B C_{1} = + R_{1}$
$D C_{2} = - R_{2}$
So Eq. $(i i i)$ can be written as
$\frac{1}{f} = (n_{21} - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}}) ... ... ... ... (i v)$ $(n_{21} = \frac{n_{2}}{n_{1}})$
Equation $(i v)$ is known as the lens maker's formula. It is useful to design lenses of desired focal length using surfaces of sutable radii of curvature. Note that the formula is true for a concave lens also. In that case $R_{1}$ is negative, $R_{2}$ positive and therefore, $f$ is negative.

Updated on:21/07/2023

Light travels from medium 1 (refractive index = $n_{1}$ ) to medium 2 (refractive index = $n_{2}$ ). If it is found that deviation d=r-i. We can say that
A $n_{1} = n_{2}$
B $n_{1} < n_{2}$
C $n_{1} > n_{2}$
Dnone of these
A ray of light travels from a medium of refractive index $n_{1}$ to
a medium of refractive index $n_{2}$ . If angle of incidence is i and
angle of refraction is r, than $\frac{sin i}{sin r}$ is equal to
Aa $n_{1}$
Bb $n_{2}$
Cc $\frac{n_{2}}{n_{1}}$
Dd $\frac{n_{1}}{n_{2}}$
When a lens of refractive index $n_{1}$ is placed in a liquid of refractive index $n_{2}$ , the lens looks to be disappeared only if
A $n_{1} = \frac{n_{2}}{2}$
B $n_{1} = \frac{3}{4} n_{2}$
C $n_{1} = n_{2}$
D $n_{1} = \frac{5}{4} n_{2}$