Curves in the graph shown in Fig. give, as function of radius distance r, the magnitude B of the magnetic field inside and outside four long wire a,b,c and d, carrying currents that are uniformly distributed across the cross sections of the wires. Overlapping portions of the plots are indicated by double labels.
Which wire has the greatest radius?
A. a
B. b
C. c
D. d
a
b
c
d
The correct Answer is:A
To solve the question of which wire has the greatest radius based on the provided graph of magnetic field strength as a function of radius, we can follow these steps:
1. Understand the Magnetic Field Behavior:
The magnetic field B inside a long straight wire carrying a current is given by the formula:
B=μ0Ir2πR2
where μ0 is the permeability of free space, I is the current, r is the distance from the center of the wire, and R is the radius of the wire.
2. Analyze the Graph:
The graph shows the magnetic field B as a function of the radius r for four wires (A, B, C, and D). The curves represent the magnetic field inside and outside each wire.
3. Identify the Regions of the Graph:
- Inside the wire, the magnetic field increases linearly with r until it reaches the wire's radius R.
- Outside the wire, the magnetic field decreases with 1/r.
4. Look for Maximum Radius:
To determine which wire has the greatest radius, we need to identify the point where each wire's curve transitions from the linear increase (inside the wire) to the decrease (outside the wire). This transition point indicates the radius of the wire.
5. Compare the Transition Points:
By examining the graph:
- Identify the point where each wire's curve ends (the maximum radius).
- Compare these points for wires A, B, C, and D.
6. Conclusion:
From the analysis of the graph, it is observed that wire C has the highest transition point, indicating that it has the greatest radius compared to wires A, B, and D.
Final Answer:
The wire with the greatest radius is C.
---
The magnetic field B inside a long straight wire carrying a current is given by the formula:
B=μ0Ir2πR2
where μ0 is the permeability of free space, I is the current, r is the distance from the center of the wire, and R is the radius of the wire.
The graph shows the magnetic field B as a function of the radius r for four wires (A, B, C, and D). The curves represent the magnetic field inside and outside each wire.
- Inside the wire, the magnetic field increases linearly with r until it reaches the wire's radius R.
- Outside the wire, the magnetic field decreases with 1/r.
To determine which wire has the greatest radius, we need to identify the point where each wire's curve transitions from the linear increase (inside the wire) to the decrease (outside the wire). This transition point indicates the radius of the wire.
By examining the graph:
- Identify the point where each wire's curve ends (the maximum radius).
- Compare these points for wires A, B, C, and D.
From the analysis of the graph, it is observed that wire C has the highest transition point, indicating that it has the greatest radius compared to wires A, B, and D.
The wire with the greatest radius is C.
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-2 PART-3 COMPREHENSION- 35videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-3 PART-120videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-2 PART-3 COMPREHENSION-15videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P19videos
ELECTROMAGNETIC INDUCTION
Similar Questions
Curves in the graph shown in Fig. give, as function of radius distance r, the magnitude B of the magnetic field inside and outside four long wire a,b,c and d, carrying currents that are uniformly distributed across the cross sections of the wires. Overlapping portions of the plots are indicated by double labels.
Which wire has the greatest radius?
A. a
B. b
C. c
D. dView SolutionCurves in the graph shown in Fig. give, as function of radius distance r, the magnitude B of the magnetic field inside and outside four long wire a,b,c and d, carrying currents that are uniformly distributed across the cross sections of the wires. Overlapping portions of the plots are indicated by double labels.

which wire has the greatest magnitude of the magnetic field on the surface?View SolutionCurves in the graph shown in Fig. give, as function of radius distance r, the magnitude B of the magnetic field inside and outside four long wire a,b,c and d, carrying currents that are uniformly distributed across the cross sections of the wires. Overlapping portions of the plots are indicated by double labels.

The current density in wire a isView SolutionCurves in the graph shown in Fig. give, as function of radius distance r, the magnitude B of the magnetic field inside and outside four long wire a,b,c and d, carrying currents that are uniformly distributed across the cross sections of the wires. Overlapping portions of the plots are indicated by double labels.
The current density in wire a is
A. greater than in wire c.
B. less than in wire c.
C. equal to that in wire c.
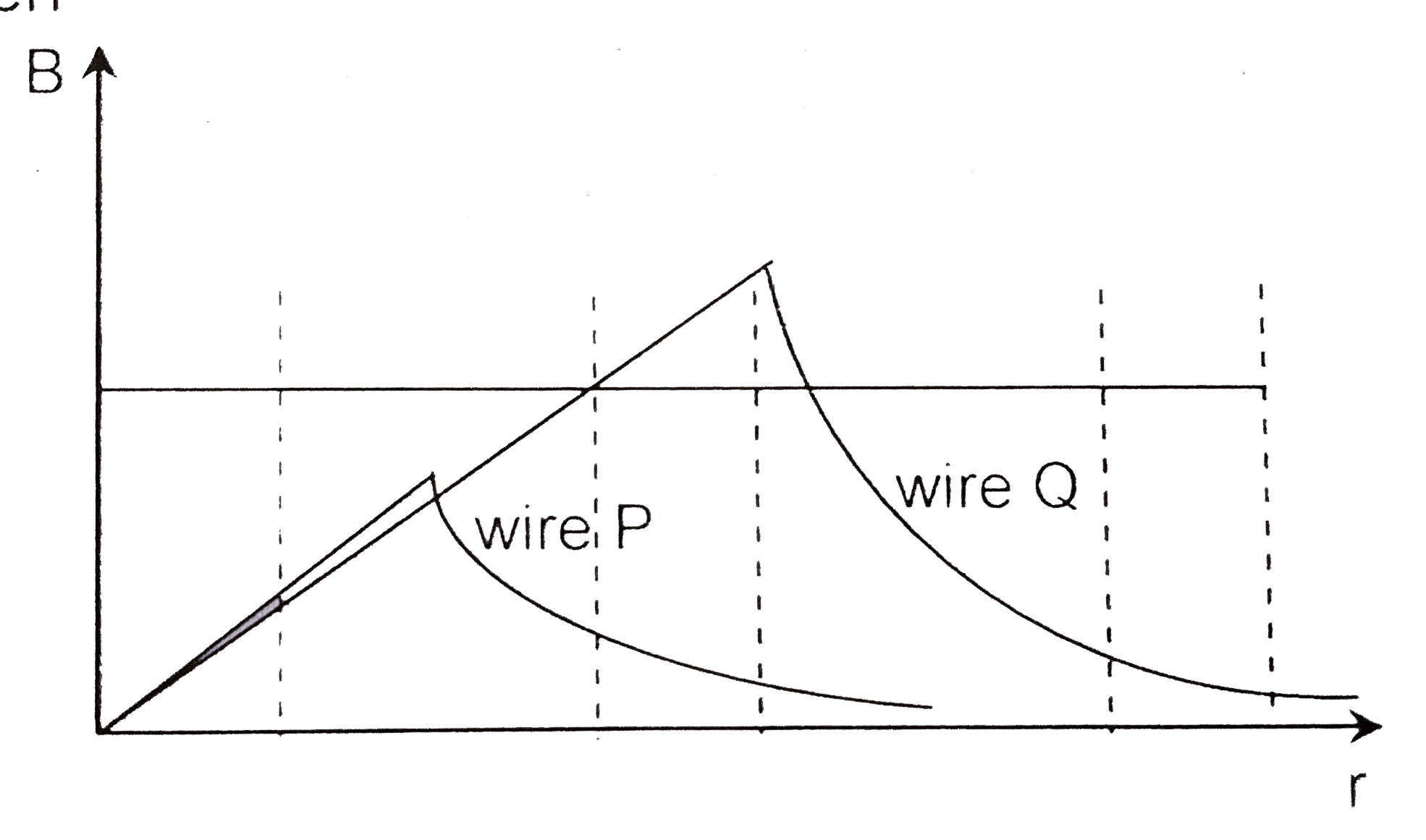
D. not comparable to that of in wire c due to lack of informationView SolutionFigure shown variation of magnetic field inside and outside two cylindrical wires P and Q carrying currents that are uniformly distributed across the cross section of wires. If current density for two wires are JP and JQ then
 View Solution
View SolutionA uniform current I is flowing in a long wire of radius R. If the current is uniformly distributed across the cross-sectional area of the wire, then
View SolutionA cylindrical long wire of radius R carries a current I uniformly distributed over the cross sectional area of the wire. The magnetic field at a distance x from the surface inside the wire is
View SolutionA long straight wire of radius a carries a steady current i. The current is uniformly distributed across its cross section. The ratio of the magnetis field at (a)/(2)and(2a) is
View SolutionA long, straight wire of radius R carries a current distributed uniformly over its cross section. The magnitude of the magnetic field is
View SolutionA long straight conducting solid cylindrical wire of radius R carries a steady current / that is uniformly distributed throughout the cross section of the wire. Draw graph of magnetic field B versus r (where r is distance from the axis of the wire)
View Solution

