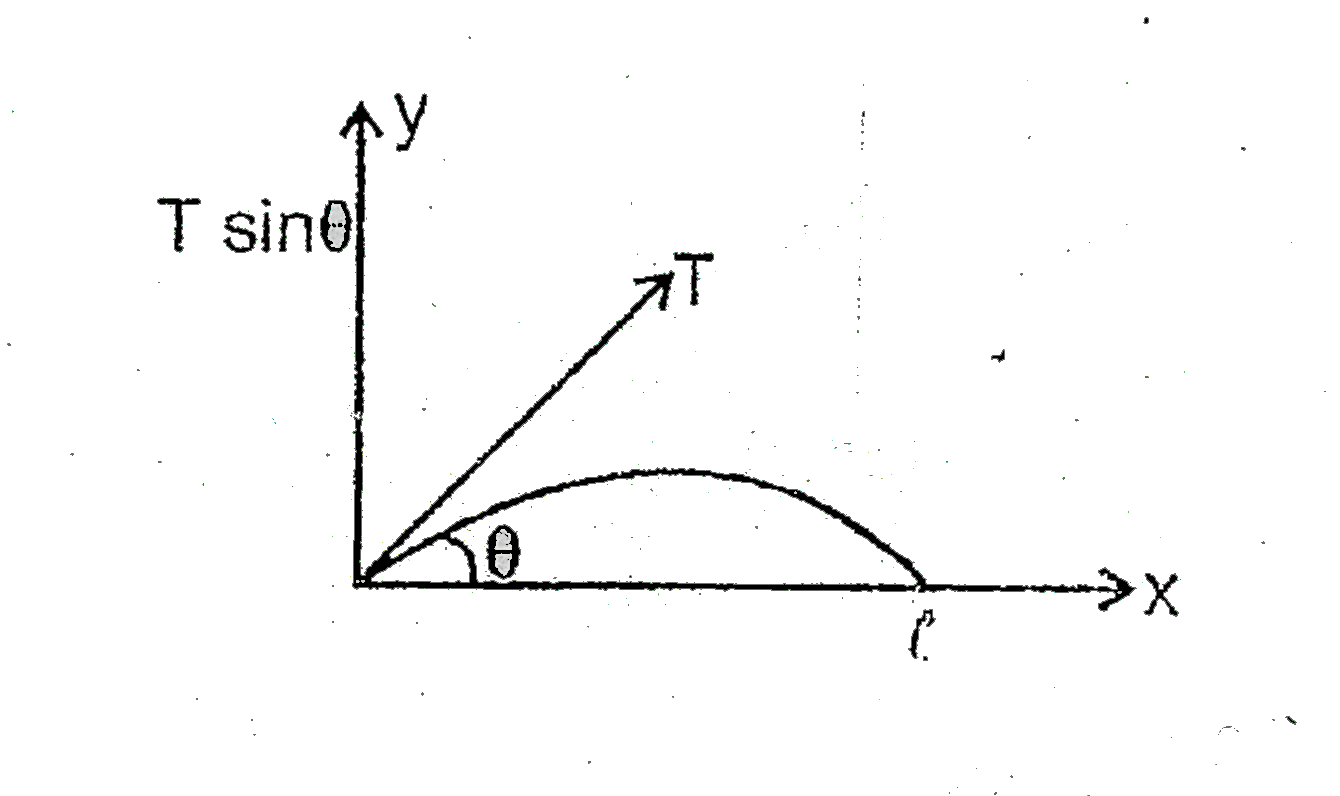
A string of length 50cm is vibrating with a fundamental frequency of 400Hz in horizontal position. In the fundamental mode the maximum displacement at the middle is 2cm from equilibrium position and the tension in the string is 10N. Determine the maximum value of the vertical component of force on the end supporting.
Fy=Tsinθ=tsinθ
=T∂y∂x
y=Asin(ωT−kx)
=0.02sin(ωt−2π0.80×2x)
∂y∂x=−0.02×π0.80cos(ωt−π0.80x)
At x=0
∂y∂x=−280πcosωt
[∂y∂x]max=π40
Fy=[T∂y∂x]=10π40=π4Ns
Topper's Solved these Questions
Similar Questions
A string has a length of 5 cm between fixed points and has a fundamental frequency of 20 Hz. What is the frequency of the second overtone?
View SolutionA string of length 105 cm produces fundamental frequency of 142 Hz. How much should its length be reduced to produce a fundamental frequency of 213 Hz.
View SolutionA wire of length 2.00 m is stretched to a tension of 160 N. If the fundamental frequency of vibration is 100 Hz, find its linear mass density.
View SolutionA 1 cm long string vibrates with fundamental frequency of 256 Hz . If the length is reduced to 14 cm keeping the tension unaltered, the new fundamental frequency will be
View SolutionA uniform string is clamped at x=0 and x = L and is vibrating in its fundamental mode. Mass per unit length of the string is μ, tension in it is T and the maximum displacement of its midpoint is A. Find the total energy stored in the string. Assume to be small so that changes in tension and length of the string can be ignored
View SolutionA string of length 1.5 m with its two ends clamped is vibrating in fundamental mode. Amplitude at the centre of the string is 4 mm. Minimum distance between the two points having amplitude 2 mm is:
View SolutionA string of length 1.5 m with its two ends clamped is vibrating in fundamental mode. Amplitude at the centre of the string is 4 mm. Minimum distance between the two points having amplitude 2 mm is:
View SolutionA string in a musical instrument is 50 cm long and its fundamental frequency is 800 Hz. If a frequency of 1000 Hz is to be produced, then required length of string is
View SolutionThe fundamental frequency of a wire of certain length is 400 Hz. When the length of the wire is decreased by 10 cm, without changing the tension in the wire, the frequency becomes 500 Hz. What was the original length of the wire ?
View SolutionA stone of mass 2.0 kg is tied to the end of a string of 2m length. It is whirled in a horizontal circle. If the breaking tension of the string is 400 N, calculate the maximum velocity of the stone.
View Solution

