Hydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the wavelength of light (nm) for the electronic transition of H-atom from the first excited state to ground state.
In the model of hydrogen like atom put forward by Niels Bohr (1913) the electron orbits around the central nucleus. the bohr radius of nth orbit of a hydrogen-like species is given by
r=kn2Z " " where, k is constant
The correct Answer is:121.9nm
To calculate the wavelength of light for the electronic transition of the hydrogen atom from the first excited state to the ground state, we will follow these steps:
Step 1: Identify the energy levels
The energy of the electron in a hydrogen-like atom is given by the formula:
En=−RHZ2n2
For hydrogen, Z=1.
Step 2: Calculate the energy of the ground state (n=1)
Using n=1:
E1=−RH⋅1212=−RH
Step 3: Calculate the energy of the first excited state (n=2)
Using n=2:
E2=−RH⋅1222=−RH4
Step 4: Calculate the energy difference between the two states
The energy difference (ΔE) between the first excited state and the ground state is:
ΔE=E2−E1
ΔE=(−RH4)−(−RH)
ΔE=−RH4+RH
ΔE=RH−RH4=3RH4
Step 5: Use the energy-wavelength relationship
The energy of a photon is related to its wavelength (λ) by the equation:
E=hcλ
Where:
- h is Planck's constant (6.626×10−34Js)
- c is the speed of light (3.00×108m/s)
Rearranging for λ:
λ=hcΔE
Step 6: Substitute ΔE into the wavelength equation
Substituting ΔE=3RH4:
λ=hc3RH4=4hc3RH
Step 7: Calculate the wavelength
Using the value of RH (approximately 2.18×10−18J):
λ=4⋅(6.626×10−34Js)⋅(3.00×108m/s)3⋅(2.18×10−18J)
Calculating this gives:
λ≈7.957×10−256.54×10−18
λ≈121.6nm
Final Answer:
The wavelength of light for the electronic transition of the H-atom from the first excited state to the ground state is approximately 121.6 nm.
The energy of the electron in a hydrogen-like atom is given by the formula:
En=−RHZ2n2
For hydrogen, Z=1.
Using n=1:
E1=−RH⋅1212=−RH
Using n=2:
E2=−RH⋅1222=−RH4
The energy difference (ΔE) between the first excited state and the ground state is:
ΔE=E2−E1
ΔE=(−RH4)−(−RH)
ΔE=−RH4+RH
ΔE=RH−RH4=3RH4
The energy of a photon is related to its wavelength (λ) by the equation:
E=hcλ
Where:
- h is Planck's constant (6.626×10−34Js)
- c is the speed of light (3.00×108m/s)
λ=hcΔE
Substituting ΔE=3RH4:
λ=hc3RH4=4hc3RH
Using the value of RH (approximately 2.18×10−18J):
λ=4⋅(6.626×10−34Js)⋅(3.00×108m/s)3⋅(2.18×10−18J)
λ≈7.957×10−256.54×10−18
λ≈121.6nm
The wavelength of light for the electronic transition of the H-atom from the first excited state to the ground state is approximately 121.6 nm.
Similar Questions
Hydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy in Joule of an electron in the second orbit of H- atom is:
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
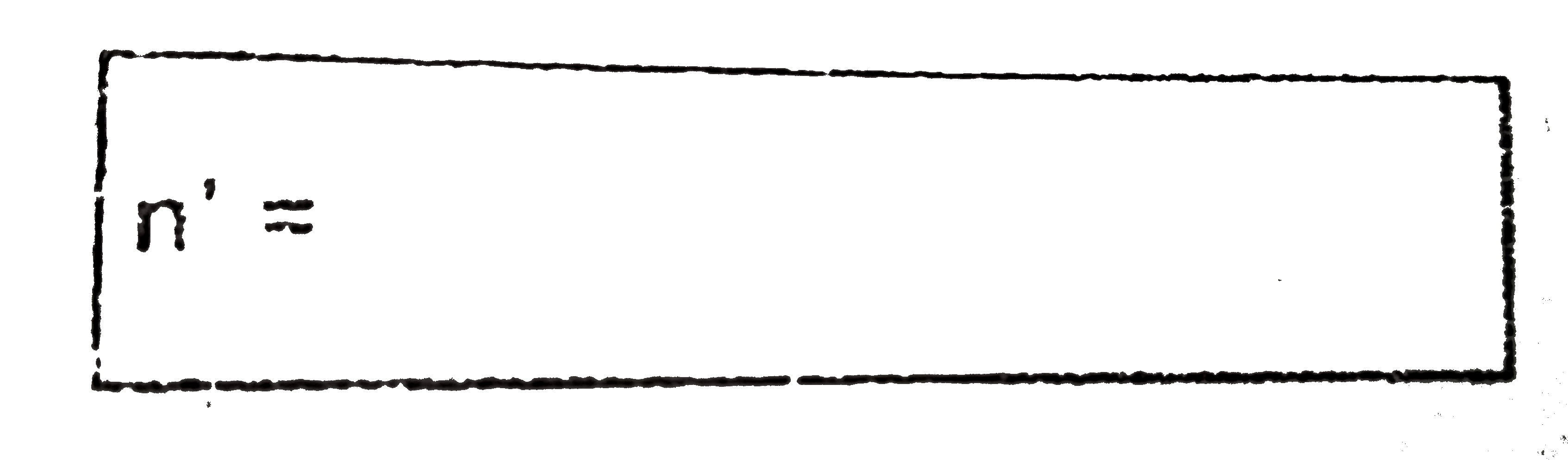
What is the principal quantum number, n' of the orbit of Be3 that has the same Bohr radius as that of ground state hydrogen atom ?
View Solution
Knowledge Check
the energy required to excite an electron in hydrogen atom to its first excited state is
A8.5eVB10.2eVC12.7eVD13.6eV
Hydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the following :
(a) the kinetic energy (in eV) of an electron in the ground state of hydrogen atom.
(b) the potential energy (in eV) of an electron in the ground state of hydrogen atom.
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy required to promote the ground state electron of H-atom to the first excited state is:
When an electron returns from a higher energy level to a lower energy level, energy is given out in the form of UV//Visible radiation.
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The ratio of energy of an electron in the ground state Be3− ion to that of ground state H atom is:
The kinetic and potential energies of an electron in the H atoms are given as
K.E.=e24πε02r and P.E.=−14πε0e2r
View SolutionIf the electron of a hydrogen atom is present in the first orbit. The total energy of the electrons is
View SolutionThe energy of the electron in the ground state of hydrogen atom is −13.6eV. Find the kinetic energy of electron in this state.
View SolutionThe speed of an electron in the orbit of hydrogen atom in the ground state is
View SolutionThe energy of the electron in the ground state of hydrogen atom is −13.6eV. Find the kinetic energy and potential energy of electron in this state.
View Solution
