You are given 4 identical balls. What is the maximum number of square voids and triangular voids (in separate arrangements) that can be created ?
1,2
2,1
3,1
1,3
The correct Answer is:A
To solve the problem of determining the maximum number of square voids and triangular voids that can be created using four identical balls, we will analyze the arrangements of these balls step by step.
Step 1: Understanding Square Voids
A square void is formed when four balls are arranged in such a way that they create a square-shaped empty space in the center. This arrangement requires exactly four balls.
- Arrangement: Place the four balls at the corners of a square.
- Result: This arrangement creates one square void in the center.
Step 2: Counting Square Voids
Since we only have four balls, and they can only form one square void when arranged at the corners, the maximum number of square voids is:
- Maximum Square Voids = 1
Step 3: Understanding Triangular Voids
A triangular void is formed when three balls are arranged in such a way that they create a triangular-shaped empty space in the center. This arrangement requires exactly three balls.
- Arrangement: Place three balls in a triangular formation (e.g., one ball at the top and two balls at the bottom).
- Result: This arrangement creates one triangular void in the center.
Step 4: Counting Triangular Voids
With the remaining ball, we can create additional triangular voids by rearranging the balls. Here’s how we can do it:
1. First Triangular Void: Arrange three balls in a triangular formation.
2. Second Triangular Void: The fourth ball can be placed in such a way that it does not interfere with the triangular void created by the first three balls. For example, it can be placed on top of one of the balls or outside the triangular formation.
- Result: We can create two distinct triangular voids with the four balls.
Conclusion
After analyzing both arrangements, we conclude:
- Maximum Square Voids = 1
- Maximum Triangular Voids = 2
Thus, the final answer is:
1 square void and 2 triangular voids.
Final Answer:
1, 2
---
A square void is formed when four balls are arranged in such a way that they create a square-shaped empty space in the center. This arrangement requires exactly four balls.
- Result: This arrangement creates one square void in the center.
Since we only have four balls, and they can only form one square void when arranged at the corners, the maximum number of square voids is:
- Maximum Square Voids = 1
A triangular void is formed when three balls are arranged in such a way that they create a triangular-shaped empty space in the center. This arrangement requires exactly three balls.
- Result: This arrangement creates one triangular void in the center.
With the remaining ball, we can create additional triangular voids by rearranging the balls. Here’s how we can do it:
2. Second Triangular Void: The fourth ball can be placed in such a way that it does not interfere with the triangular void created by the first three balls. For example, it can be placed on top of one of the balls or outside the triangular formation.
After analyzing both arrangements, we conclude:
- Maximum Square Voids = 1
- Maximum Triangular Voids = 2
1 square void and 2 triangular voids.
1, 2
Topper's Solved these Questions
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -2 (Part-I)
19 Videos
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -2 (Part-II Single correct double value integer type)
9 Videos
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -1
20 Videos
- S BLOCK ELEMENTSBOOK - RESONANCE ENGLISHCHAPTER - S BLOCK ELEMENTSEXERCISE - ORGANIC CHEMISTRY(Biomolecules & Polymer)
4 Videos
- STRUCTURAL IDENTIFICATION & PRACTICAL ORGANIC CHEMISTRYBOOK - RESONANCE ENGLISHCHAPTER - STRUCTURAL IDENTIFICATION & PRACTICAL ORGANIC CHEMISTRYEXERCISE - Section -B
18 Videos
Similar Questions
Knowledge Check
What is the maximum number of 3x3 square that can be formed from the square in the 6x6 checkerboard shown?
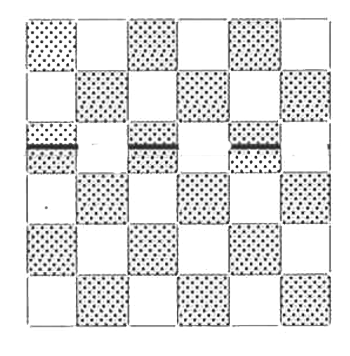 A4B6C12D16
A4B6C12D16
Some identical balls are arranged in rows to form an equilateral triangle. The first row consists of one ball, the second row consists of row balls and so on. If 99 more identical balls are added to the total number of balls used in forming the equilateral triangle, then all these balls can be arranged in a square whose each side contains exactly 2 balls less than the number of balls each side of the triangle contains. Then, the number of balls used to form the equilateral triangle is
View SolutionWhat is the maximum number of electron in an atom that can have the quantum numbers n=4,ml=+1?
View SolutionWhat is the number and closest distance between two octahedral voids and two tetrahdral voids in fcc unit cell ?
View SolutionCan a rectangular number also be a square number? Can a triangular number also be a square number?
View SolutionYou are given 8 balls of different colour (black,white ......). The number of ways in which these balls can be arranged in a row so that the two balls of particular colour (say red and white) may never come together is-
View SolutionYou are given a mixture of ZnSandPbS. The two compounds can be separated by
View SolutionThere are 2 identical white balls, 3 identical red balls, and 4 green balls of different shades. Find the number of ways in which they can be arranged in a row so that atleast one ball is separated from the balls of the same color.
View Solution
