A line is drawn passing through point P(1,2) to cut positive coordinate axes at A and B . Find minimum area of .
The correct Answer is:
To find the minimum area of triangle PAB formed by a line passing through the point P(1, 2) and cutting the positive coordinate axes at points A and B, we can follow these steps:
Step 1: Define Points A and B
Let the coordinates of point A (where the line intersects the y-axis) be (0, k) and the coordinates of point B (where the line intersects the x-axis) be (h, 0).
Step 2: Equation of the Line
Using the intercept form of the equation of a line, we have:
xh+yk=1
Since the line passes through the point P(1, 2), we can substitute these values into the equation:
1h+2k=1
Step 3: Express k in terms of h
From the equation above, we can express k in terms of h:
2k=1−1h
2k=h−1h
Cross-multiplying gives:
2h=k(h−1)
Thus, we can express k as:
k=2hh−1
Step 4: Area of Triangle PAB
The area A of triangle PAB can be calculated using the formula:
A=12×base×height=12×h×k
Substituting the value of k we found:
A=12×h×2hh−1=h2h−1
Step 5: Find the Minimum Area
To find the minimum area, we need to differentiate A with respect to h and set the derivative to zero:
A(h)=h2h−1
Using the quotient rule for differentiation:
A′=(h−1)(2h)−h2(1)(h−1)2
Simplifying the numerator:
=2h2−2h−h2(h−1)2=h2−2h(h−1)2
Setting the derivative equal to zero to find critical points:
h2−2h=0⟹h(h−2)=0
Thus, h=0 or h=2. Since we are interested in positive values, we take h=2.
Step 6: Calculate Minimum Area
Substituting h=2 back into the area formula:
A=222−1=41=4
Conclusion
The minimum area of triangle PAB is 4 square units.
---
Let the coordinates of point A (where the line intersects the y-axis) be (0, k) and the coordinates of point B (where the line intersects the x-axis) be (h, 0).
Using the intercept form of the equation of a line, we have:
xh+yk=1
Since the line passes through the point P(1, 2), we can substitute these values into the equation:
1h+2k=1
From the equation above, we can express k in terms of h:
2k=1−1h
2k=h−1h
Cross-multiplying gives:
2h=k(h−1)
Thus, we can express k as:
k=2hh−1
The area A of triangle PAB can be calculated using the formula:
A=12×base×height=12×h×k
Substituting the value of k we found:
A=12×h×2hh−1=h2h−1
To find the minimum area, we need to differentiate A with respect to h and set the derivative to zero:
A(h)=h2h−1
Using the quotient rule for differentiation:
A′=(h−1)(2h)−h2(1)(h−1)2
Simplifying the numerator:
=2h2−2h−h2(h−1)2=h2−2h(h−1)2
Setting the derivative equal to zero to find critical points:
h2−2h=0⟹h(h−2)=0
Thus, h=0 or h=2. Since we are interested in positive values, we take h=2.
Substituting h=2 back into the area formula:
A=222−1=41=4
The minimum area of triangle PAB is 4 square units.
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1A
5 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise -1B
6 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - High Level Problems (HLP)
35 Videos
- COMBINATORICSBOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)
5 Videos
Similar Questions
Let (h,k) be a fixed point, where h>0,k>0. A straight line passing through this point cuts the positive direction of the coordinate axes at the point PandQ . Find the minimum area of triangle OPQ,O being the origin.
View SolutionA straight line l with negative slope passes through (8,2) and cuts the coordinate axes at P and Q. Find absolute minimum value of ''OP+OQ where O is the origin-
View SolutionA line is drawn through the point (1, 2) to meet the coordinate axes at P and Q such that it forms a triangle OPQ, where O is the origin. If the area of the triangle OPQ is least, then the slope of the line PQ is
View SolutionA line is drawn through the point P(3,11) to cut the circle x2+y2=9 at A and B. Then PA.PB is equal to
View SolutionA variable line passing through point P(2,1) meets the axes at A and B . Find the locus of the circumcenter of triangle OAB (where O is the origin).
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
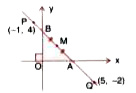
The equation of the line.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
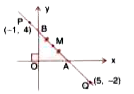
The co-ordinates of A and B.
View SolutionFind the eqution of the curve passing through the point (1,1), if the tangent drawn at any point P(x,y) on the curve meets the coordinate axes at A and B such that P is the mid point of AB.
View SolutionFind the equation of straight line which passes through the point P(2,6) and cuts the coordinate axis at the point A and B respectively so that AP:BP=2:3.
View SolutionIf a line passes through the point P(1,−2) and cuts the x2+y2−x−y=0at A and B, then themaxiμmofPA+PB is
View Solution
